
Question Number 52867 by hassentimol last updated on 14/Jan/19
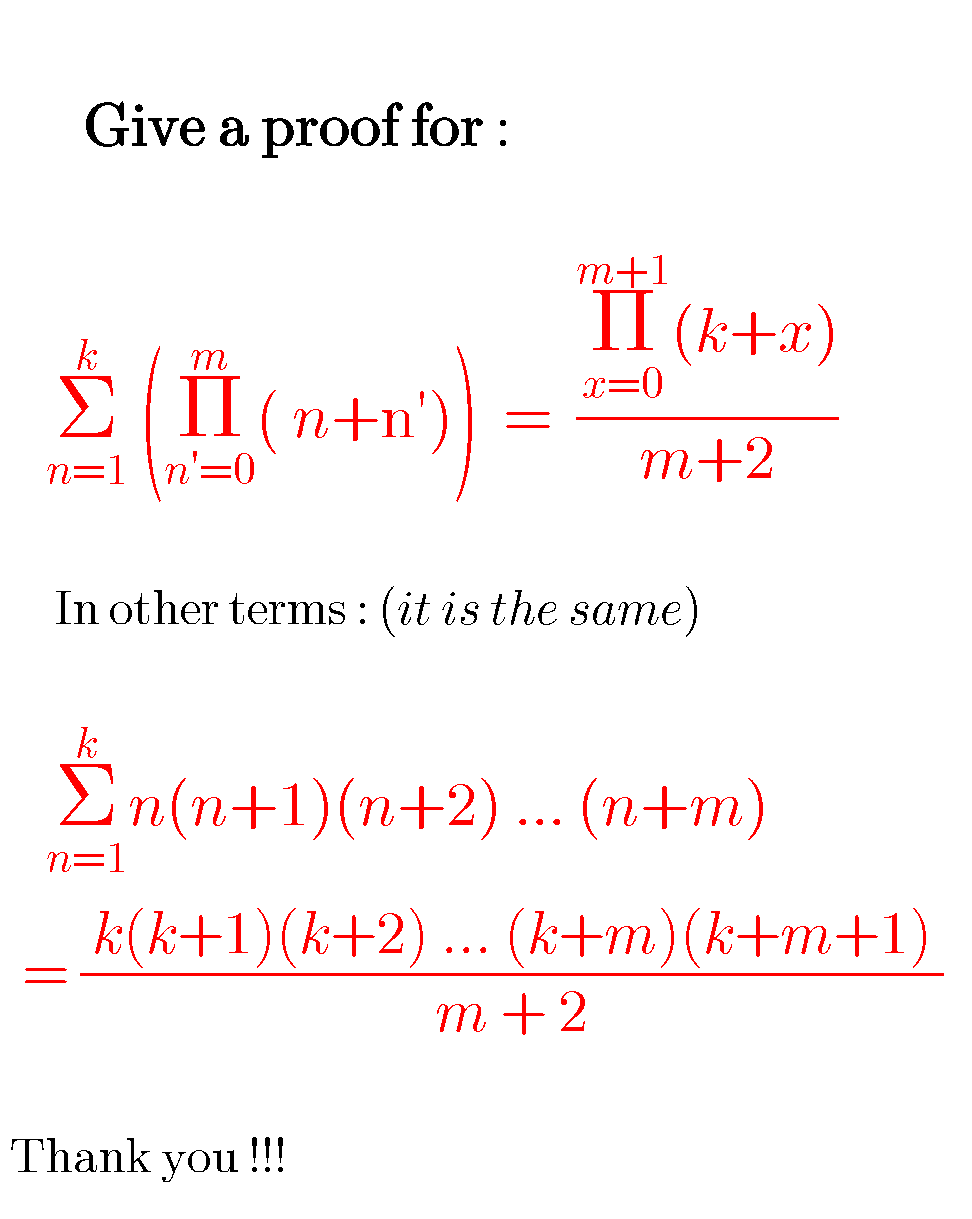
Answered by iv@0uja last updated on 19/Jan/19
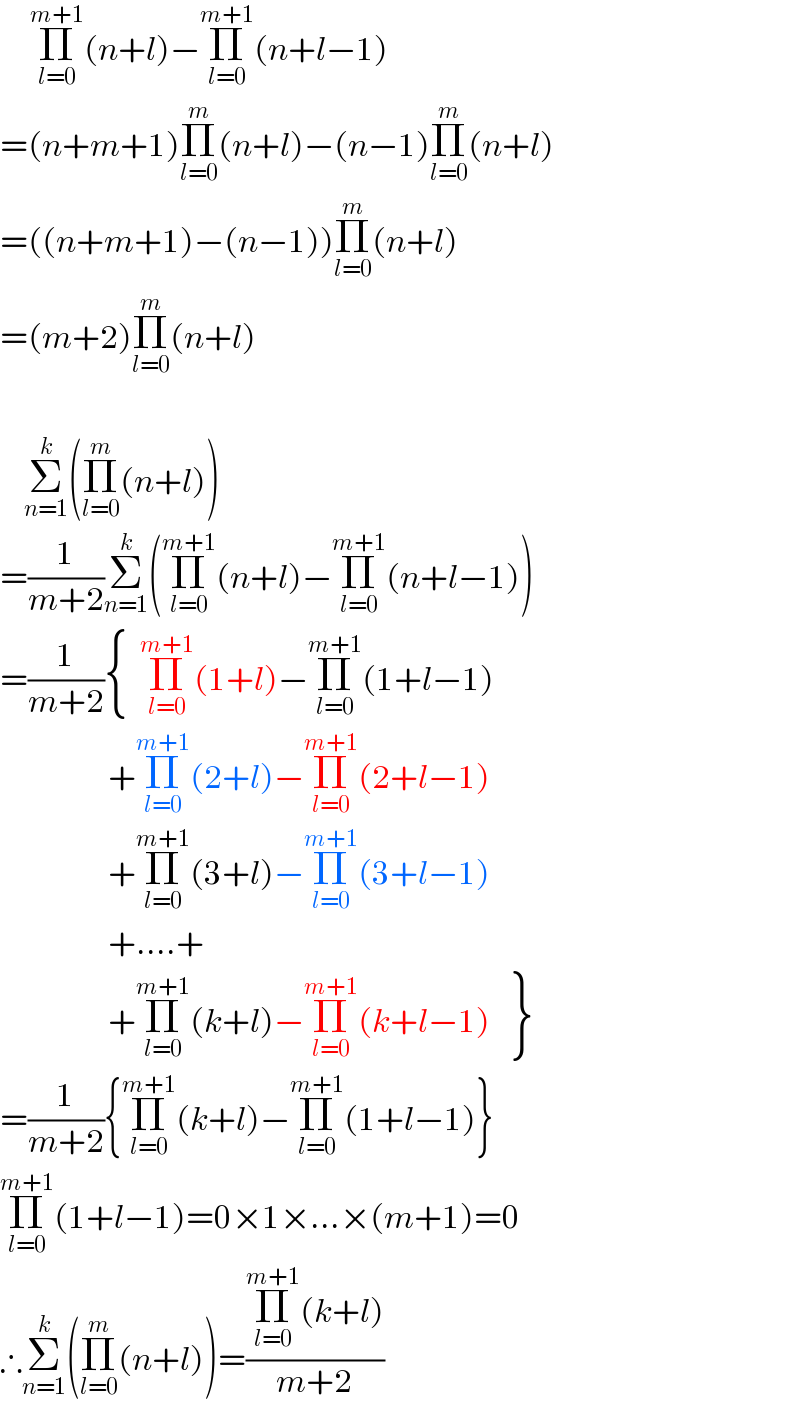
$$\:\:\:\:\:\underset{{l}=\mathrm{0}} {\overset{{m}+\mathrm{1}} {\prod}}\left({n}+{l}\right)−\underset{{l}=\mathrm{0}} {\overset{{m}+\mathrm{1}} {\prod}}\left({n}+{l}−\mathrm{1}\right) \\ $$$$=\left({n}+{m}+\mathrm{1}\right)\underset{{l}=\mathrm{0}} {\overset{{m}} {\prod}}\left({n}+{l}\right)−\left({n}−\mathrm{1}\right)\underset{{l}=\mathrm{0}} {\overset{{m}} {\prod}}\left({n}+{l}\right) \\ $$$$=\left(\left({n}+{m}+\mathrm{1}\right)−\left({n}−\mathrm{1}\right)\right)\underset{{l}=\mathrm{0}} {\overset{{m}} {\prod}}\left({n}+{l}\right) \\ $$$$=\left({m}+\mathrm{2}\right)\underset{{l}=\mathrm{0}} {\overset{{m}} {\prod}}\left({n}+{l}\right) \\ $$$$ \\ $$$$\:\:\:\:\underset{{n}=\mathrm{1}} {\overset{{k}} {\sum}}\left(\underset{{l}=\mathrm{0}} {\overset{{m}} {\prod}}\left({n}+{l}\right)\right) \\ $$$$=\frac{\mathrm{1}}{{m}+\mathrm{2}}\underset{{n}=\mathrm{1}} {\overset{{k}} {\sum}}\left(\underset{{l}=\mathrm{0}} {\overset{{m}+\mathrm{1}} {\prod}}\left({n}+{l}\right)−\underset{{l}=\mathrm{0}} {\overset{{m}+\mathrm{1}} {\prod}}\left({n}+{l}−\mathrm{1}\right)\right) \\ $$$$=\frac{\mathrm{1}}{{m}+\mathrm{2}}\begin{cases}{}\\{}\end{cases}\underset{{l}=\mathrm{0}} {\overset{{m}+\mathrm{1}} {\prod}}\left(\mathrm{1}+{l}\right)−\underset{{l}=\mathrm{0}} {\overset{{m}+\mathrm{1}} {\prod}}\left(\mathrm{1}+{l}−\mathrm{1}\right) \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:+\underset{{l}=\mathrm{0}} {\overset{{m}+\mathrm{1}} {\prod}}\left(\mathrm{2}+{l}\right)−\underset{{l}=\mathrm{0}} {\overset{{m}+\mathrm{1}} {\prod}}\left(\mathrm{2}+{l}−\mathrm{1}\right) \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:+\underset{{l}=\mathrm{0}} {\overset{{m}+\mathrm{1}} {\prod}}\left(\mathrm{3}+{l}\right)−\underset{{l}=\mathrm{0}} {\overset{{m}+\mathrm{1}} {\prod}}\left(\mathrm{3}+{l}−\mathrm{1}\right) \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:+....+ \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:+\underset{{l}=\mathrm{0}} {\overset{{m}+\mathrm{1}} {\prod}}\left({k}+{l}\right)−\underset{{l}=\mathrm{0}} {\overset{{m}+\mathrm{1}} {\prod}}\left({k}+{l}−\mathrm{1}\right)\left.\begin{matrix}{}\\{}\end{matrix}\right\} \\ $$$$=\frac{\mathrm{1}}{{m}+\mathrm{2}}\left\{\underset{{l}=\mathrm{0}} {\overset{{m}+\mathrm{1}} {\prod}}\left({k}+{l}\right)−\underset{{l}=\mathrm{0}} {\overset{{m}+\mathrm{1}} {\prod}}\left(\mathrm{1}+{l}−\mathrm{1}\right)\right\} \\ $$$$\underset{{l}=\mathrm{0}} {\overset{{m}+\mathrm{1}} {\prod}}\left(\mathrm{1}+{l}−\mathrm{1}\right)=\mathrm{0}×\mathrm{1}×...×\left({m}+\mathrm{1}\right)=\mathrm{0} \\ $$$$\therefore\underset{{n}=\mathrm{1}} {\overset{{k}} {\sum}}\left(\underset{{l}=\mathrm{0}} {\overset{{m}} {\prod}}\left({n}+{l}\right)\right)=\frac{\underset{{l}=\mathrm{0}} {\overset{{m}+\mathrm{1}} {\prod}}\left({k}+{l}\right)}{{m}+\mathrm{2}} \\ $$
Commented by hassentimol last updated on 19/Jan/19

$$\mathrm{Oh}...\:\mathrm{Thank}\:\mathrm{you}\:\mathrm{so}\:\mathrm{much}\:\mathrm{sir}... \\ $$$$\mathrm{I}\:\mathrm{am}\:\mathrm{so}\:\mathrm{grateful}...\:\mathrm{God}\:\mathrm{Bless}\:\mathrm{You}\:!!! \\ $$
