
Question Number 52360 by ajfour last updated on 06/Jan/19
Commented by ajfour last updated on 06/Jan/19
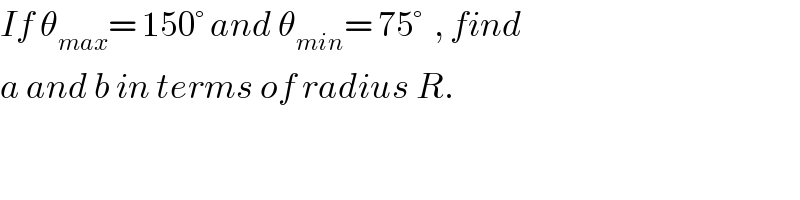
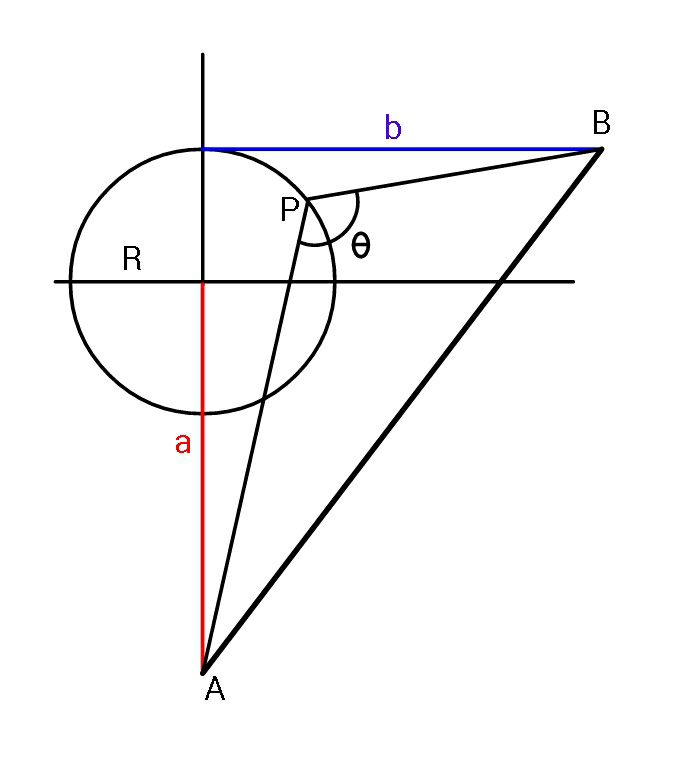
$${If}\:\theta_{{max}} =\:\mathrm{150}°\:{and}\:\theta_{{min}} =\:\mathrm{75}°\:\:,\:{find} \\ $$$${a}\:{and}\:{b}\:{in}\:{terms}\:{of}\:{radius}\:{R}. \\ $$
Answered by mr W last updated on 06/Jan/19

Commented by ajfour last updated on 06/Jan/19

$${how}\:{come}\:{the}\:{perpendicular} \\ $$$${bisector}\:{Sir}?\:{for}\:{certain}?! \\ $$
Commented by mr W last updated on 06/Jan/19
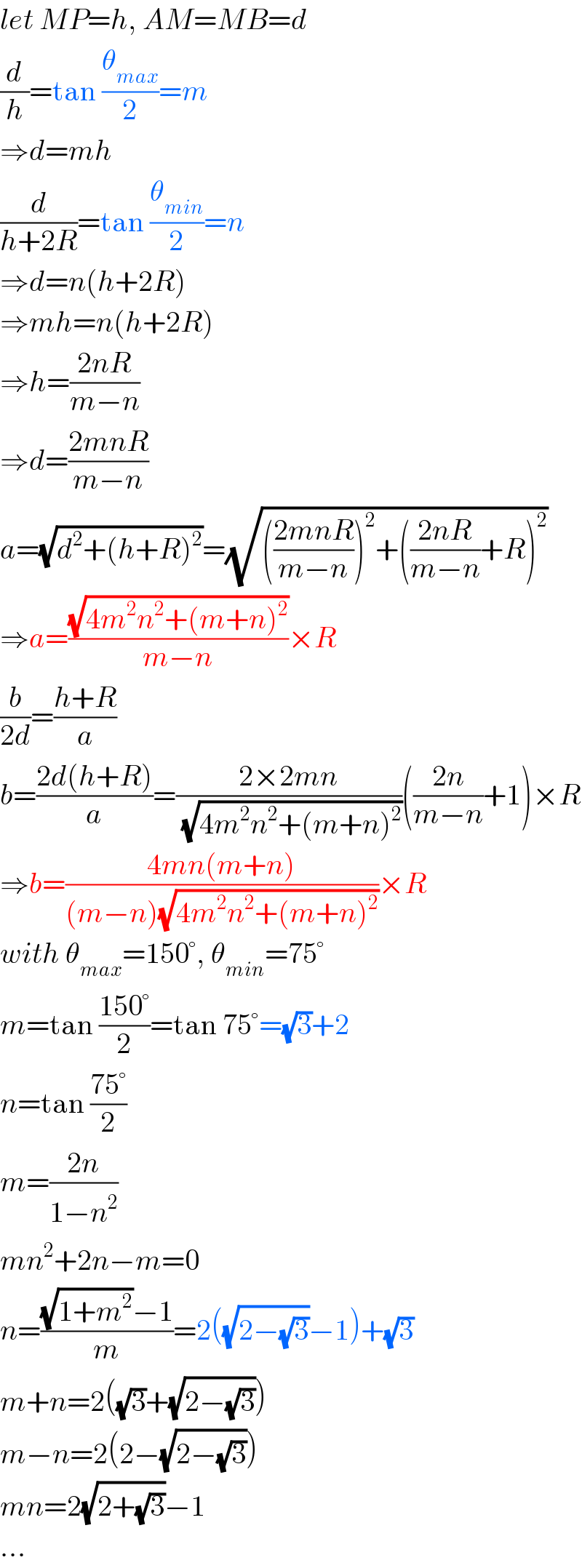
$${let}\:{MP}={h},\:{AM}={MB}={d} \\ $$$$\frac{{d}}{{h}}=\mathrm{tan}\:\frac{\theta_{{max}} }{\mathrm{2}}={m} \\ $$$$\Rightarrow{d}={mh} \\ $$$$\frac{{d}}{{h}+\mathrm{2}{R}}=\mathrm{tan}\:\frac{\theta_{{min}} }{\mathrm{2}}={n} \\ $$$$\Rightarrow{d}={n}\left({h}+\mathrm{2}{R}\right) \\ $$$$\Rightarrow{mh}={n}\left({h}+\mathrm{2}{R}\right) \\ $$$$\Rightarrow{h}=\frac{\mathrm{2}{nR}}{{m}−{n}} \\ $$$$\Rightarrow{d}=\frac{\mathrm{2}{mnR}}{{m}−{n}} \\ $$$${a}=\sqrt{{d}^{\mathrm{2}} +\left({h}+{R}\right)^{\mathrm{2}} }=\sqrt{\left(\frac{\mathrm{2}{mnR}}{{m}−{n}}\right)^{\mathrm{2}} +\left(\frac{\mathrm{2}{nR}}{{m}−{n}}+{R}\right)^{\mathrm{2}} } \\ $$$$\Rightarrow{a}=\frac{\sqrt{\mathrm{4}{m}^{\mathrm{2}} {n}^{\mathrm{2}} +\left({m}+{n}\right)^{\mathrm{2}} }}{{m}−{n}}×{R} \\ $$$$\frac{{b}}{\mathrm{2}{d}}=\frac{{h}+{R}}{{a}} \\ $$$${b}=\frac{\mathrm{2}{d}\left({h}+{R}\right)}{{a}}=\frac{\mathrm{2}×\mathrm{2}{mn}}{\sqrt{\mathrm{4}{m}^{\mathrm{2}} {n}^{\mathrm{2}} +\left({m}+{n}\right)^{\mathrm{2}} }}\left(\frac{\mathrm{2}{n}}{{m}−{n}}+\mathrm{1}\right)×{R} \\ $$$$\Rightarrow{b}=\frac{\mathrm{4}{mn}\left({m}+{n}\right)}{\left({m}−{n}\right)\sqrt{\mathrm{4}{m}^{\mathrm{2}} {n}^{\mathrm{2}} +\left({m}+{n}\right)^{\mathrm{2}} }}×{R} \\ $$$${with}\:\theta_{{max}} =\mathrm{150}°,\:\theta_{{min}} =\mathrm{75}° \\ $$$${m}=\mathrm{tan}\:\frac{\mathrm{150}°}{\mathrm{2}}=\mathrm{tan}\:\mathrm{75}°=\sqrt{\mathrm{3}}+\mathrm{2} \\ $$$${n}=\mathrm{tan}\:\frac{\mathrm{75}°}{\mathrm{2}} \\ $$$${m}=\frac{\mathrm{2}{n}}{\mathrm{1}−{n}^{\mathrm{2}} } \\ $$$${mn}^{\mathrm{2}} +\mathrm{2}{n}−{m}=\mathrm{0} \\ $$$${n}=\frac{\sqrt{\mathrm{1}+{m}^{\mathrm{2}} }−\mathrm{1}}{{m}}=\mathrm{2}\left(\sqrt{\mathrm{2}−\sqrt{\mathrm{3}}}−\mathrm{1}\right)+\sqrt{\mathrm{3}} \\ $$$${m}+{n}=\mathrm{2}\left(\sqrt{\mathrm{3}}+\sqrt{\mathrm{2}−\sqrt{\mathrm{3}}}\right) \\ $$$${m}−{n}=\mathrm{2}\left(\mathrm{2}−\sqrt{\mathrm{2}−\sqrt{\mathrm{3}}}\right) \\ $$$${mn}=\mathrm{2}\sqrt{\mathrm{2}+\sqrt{\mathrm{3}}}−\mathrm{1} \\ $$$$... \\ $$
Commented by mr W last updated on 06/Jan/19

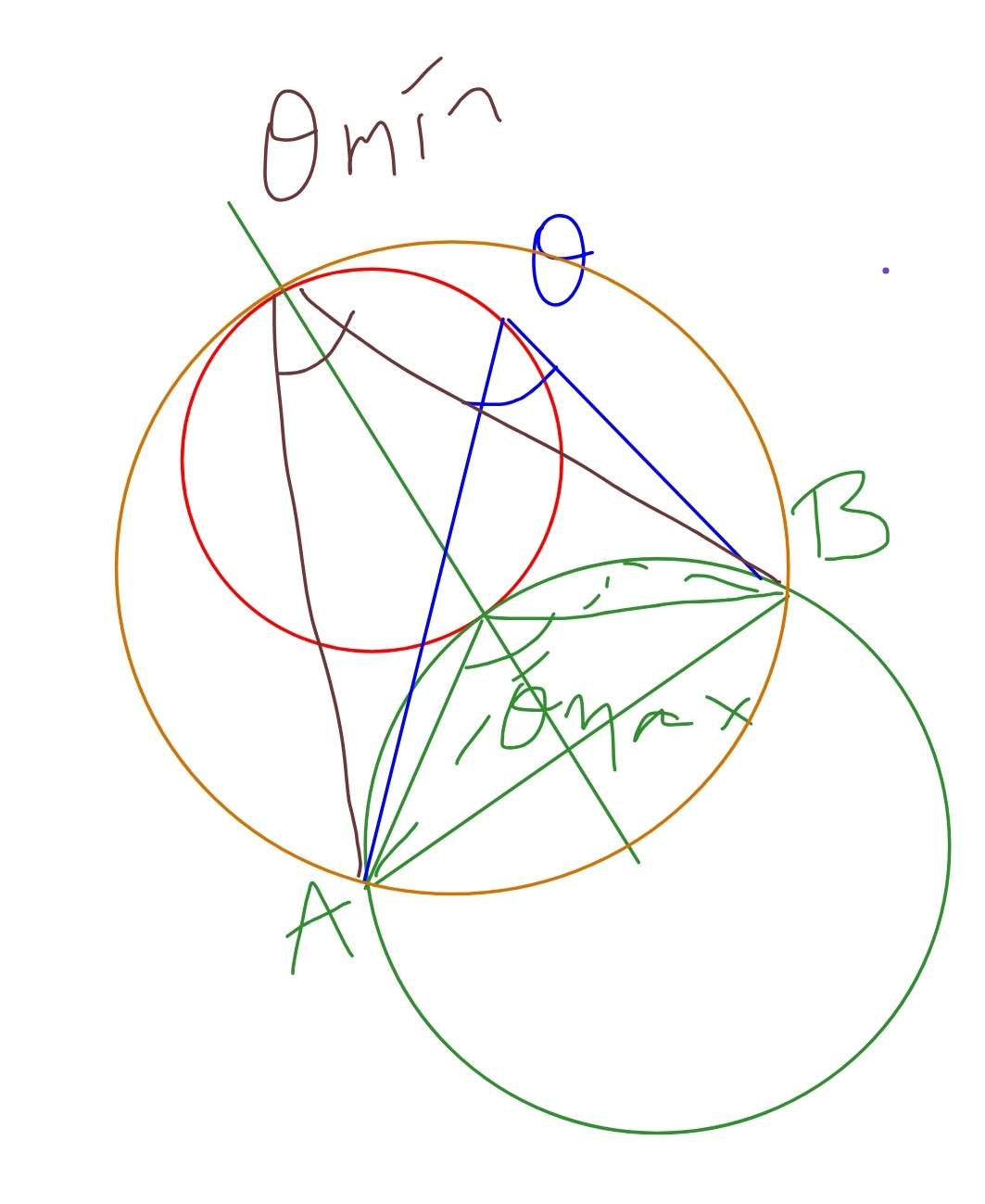
$${for}\:\theta={constant},\:{the}\:{locus}\:{of}\:{point}\:{P} \\ $$$${is}\:{a}\:{circle}\:{through}\:{points}\:{A}\:{and}\:{B}. \\ $$$${AB}\:{is}\:{a}\:{chord}\:{of}\:{this}\:{circle}.\:{such}\:{that} \\ $$$$\theta_{{max}} \:{happens},\:{this}\:{circle}\:{must}\:{tangent} \\ $$$${the}\:{circle}\:{with}\:{radius}\:{R}. \\ $$
Commented by mr W last updated on 06/Jan/19
Commented by ajfour last updated on 06/Jan/19
$${fine}\:{Sir},\:{let}\:{me}\:{check}\:{with}\:{b}=\mathrm{0}, \\ $$$${in}\:{a}\:{couple}\:{of}\:{minutes},\:{please}.. \\ $$
Commented by mr W last updated on 06/Jan/19

$${i}\:{think}\:{i}\:{made}\:{a}\:{mistake}.\:{it}\:{is}\:{correct} \\ $$$${that}\:{the}\:{circle}\:{through}\:{AB}\:{should} \\ $$$${tangent}\:{the}\:{circle}\:{with}\:{radius}\:{R},\:{but} \\ $$$${AB}\:{is}\:{not}\:{always}\:{centric}\:{to}\:{the}\:{circle} \\ $$$${with}\:{radius}\:{R}.\:{but}\:{i}\:{have}\:{assumed} \\ $$$${they}\:{are}\:{centric}\:{to}\:{each}\:{other}. \\ $$
Commented by mr W last updated on 06/Jan/19

$${i}\:{want}\:{to}\:{say},\:{my}\:{solution}\:{is}\:{correct},\: \\ $$$${but}\:{there}\:{are}\:{also}\:{other}\:{possibilities}. \\ $$
Commented by mr W last updated on 06/Jan/19

$${since}\:{both}\:{a}\:{and}\:{b}\:{are}\:{to}\:{be}\:{determined}, \\ $$$${it}\:{is}\:{ok}\:{to}\:{assume}\:{that}\:{AB}\:{is}\:{centric}\:{to} \\ $$$${the}\:{circle}\:{and}\:{we}\:{get}\:{the}\:{solution}\:{as} \\ $$$${i}\:{did}.\:{but}\:{if}\:{one}\:{of}\:{a}\:{and}\:{b}\:{is}\:{fixed},\:{then}\:{it} \\ $$$${is}\:{wrong}\:{to}\:{assume}\:{that}\:{AB}\:{is}\:{centric} \\ $$$${to}\:{the}\:{circle}. \\ $$
Commented by ajfour last updated on 06/Jan/19
$${yes}\:{Sir},\:{i}\:{tried}\:{to}\:{agree}\:{but}\:{my} \\ $$$${sketching}\:{did}\:{not}\:{let}\:{me}\:{agree}\:{totally} \\ $$$${about}\:{the}\:{perpendicular}\:{bisector} \\ $$$${line}\:{you}\:{drew},\:{not}\:{generally}. \\ $$
Commented by mr W last updated on 07/Jan/19

$${the}\:{situation}\:{is}\:{following}: \\ $$$${when}\:{a}\:{and}\:{b}\:{are}\:{given},\:{we}\:{get}\:{unique} \\ $$$$\theta_{{max}} \:{and}\:\theta_{{min}} . \\ $$$${but}\:{when}\:\theta_{{max}} \:{and}\:\theta_{{min}} \:{are}\:{given},\:{we} \\ $$$${can}\:{not}\:{get}\:{unique}\:{a}\:{and}\:{b}.\:{the}\:{solution} \\ $$$${i}\:{gave}\:{is}\:{a}\:{correct}\:{solution},\:{but}\:{not}\:{the} \\ $$$${only}\:{solution}. \\ $$
Commented by ajfour last updated on 07/Jan/19
$${oh}\:{yes},\:{Sir}.{Thank}\:{you}\:{so}\:{much}! \\ $$$${dint}\:{occur}\:{to}\:{me},\:{without}\:{your}\:{words}. \\ $$
