Question and Answers Forum

All Questions      Topic List

Others Questions

Previous in All Question      Next in All Question      

Previous in Others      Next in Others      

Question Number 49283 by Pk1167156@gmail.com last updated on 05/Dec/18

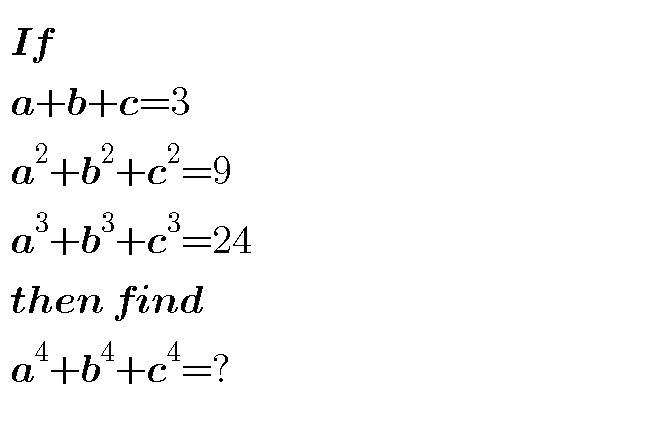
Answered by afachri last updated on 05/Dec/18

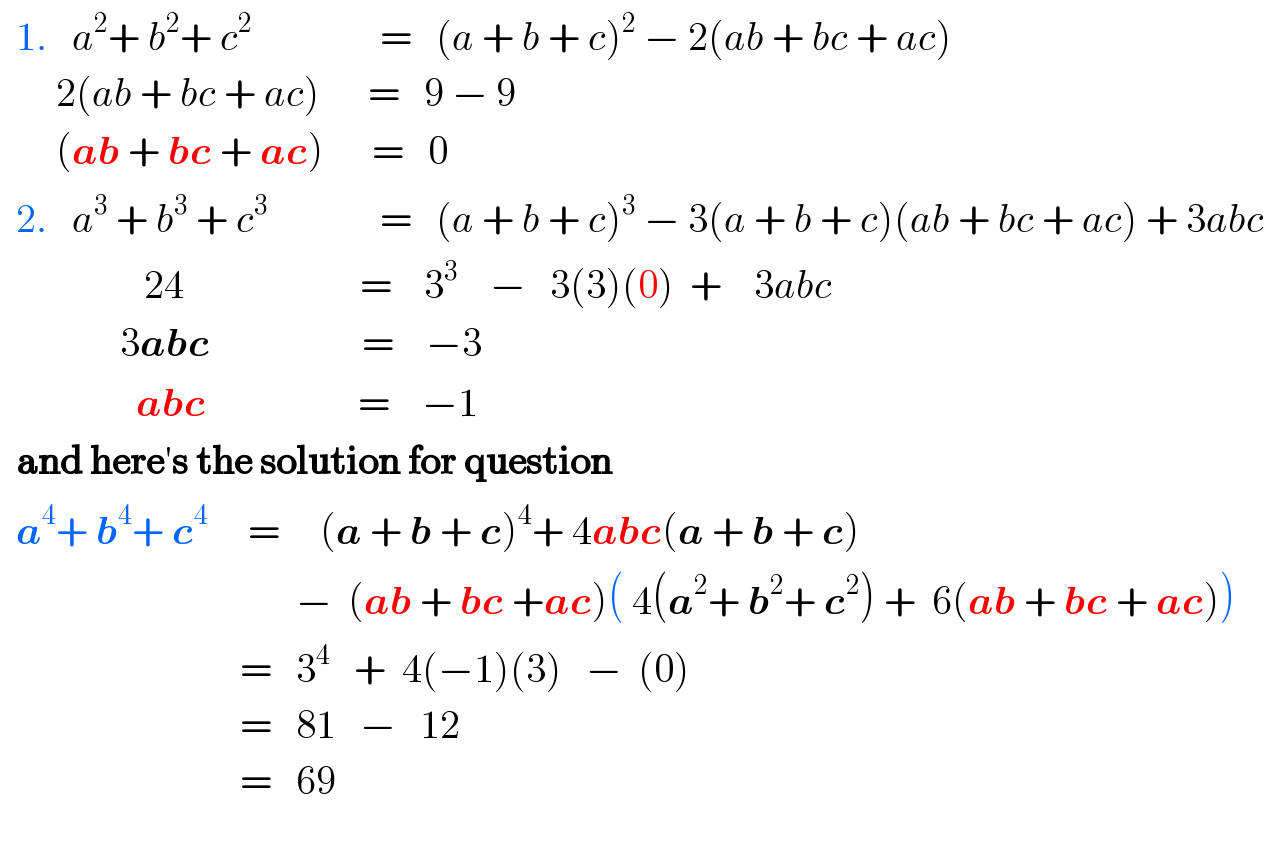
1. a^2 + b^2 + c^2 = (a + b + c)^2 − 2(ab + bc + ac) 2(ab + bc + ac) = 9 − 9 (ab + bc + ac) = 0 2. a^3 + b^3 + c^3 = (a + b + c)^3 − 3(a + b + c)(ab + bc + ac) + 3abc 24 = 3^3 − 3(3)(0) + 3abc 3abc = −3 abc = −1 and here′s the solution for question a^4 + b^4 + c^4 = (a + b + c)^4 + 4abc(a + b + c) − (ab + bc +ac)( 4(a^2 + b^2 + c^2 ) + 6(ab + bc + ac)) = 3^4 + 4(−1)(3) − (0) = 81 − 12 = 69

$$\:\:\mathrm{1}.\:\:\:{a}^{\mathrm{2}} +\:{b}^{\mathrm{2}} +\:{c}^{\mathrm{2}} \:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\:\:\:\left({a}\:+\:{b}\:+\:{c}\right)^{\mathrm{2}} \:−\:\mathrm{2}\left({ab}\:+\:{bc}\:+\:{ac}\right) \\ $$$$\:\:\:\:\:\:\:\mathrm{2}\left({ab}\:+\:{bc}\:+\:{ac}\right)\:\:\:\:\:\:=\:\:\:\mathrm{9}\:−\:\mathrm{9} \\ $$$$\:\:\:\:\:\:\:\left(\boldsymbol{{ab}}\:+\:\boldsymbol{{bc}}\:+\:\boldsymbol{{ac}}\right)\:\:\:\:\:\:=\:\:\:\mathrm{0} \\ $$$$\:\:\mathrm{2}.\:\:\:{a}^{\mathrm{3}} \:+\:{b}^{\mathrm{3}} \:+\:{c}^{\mathrm{3}} \:\:\:\:\:\:\:\:\:\:\:\:\:\:=\:\:\:\left({a}\:+\:{b}\:+\:{c}\right)^{\mathrm{3}} \:−\:\mathrm{3}\left({a}\:+\:{b}\:+\:{c}\right)\left({ab}\:+\:{bc}\:+\:{ac}\right)\:+\:\mathrm{3}{abc} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{24}\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\:\:\:\:\mathrm{3}^{\mathrm{3}} \:\:\:\:−\:\:\:\mathrm{3}\left(\mathrm{3}\right)\left(\mathrm{0}\right)\:\:+\:\:\:\:\mathrm{3}{abc}\: \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{3}\boldsymbol{{abc}}\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\:\:\:\:−\mathrm{3} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\boldsymbol{{abc}}\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\:\:\:\:−\mathrm{1} \\ $$$$\:\:\boldsymbol{\mathrm{and}}\:\boldsymbol{\mathrm{here}}'\boldsymbol{\mathrm{s}}\:\boldsymbol{\mathrm{the}}\:\boldsymbol{\mathrm{solution}}\:\boldsymbol{\mathrm{for}}\:\boldsymbol{\mathrm{question}} \\ $$$$\:\:\boldsymbol{{a}}^{\mathrm{4}} +\:\boldsymbol{{b}}^{\mathrm{4}} +\:\boldsymbol{{c}}^{\mathrm{4}} \:\:\:\:\:=\:\:\:\:\:\left(\boldsymbol{{a}}\:+\:\boldsymbol{{b}}\:+\:\boldsymbol{{c}}\right)^{\mathrm{4}} +\:\mathrm{4}\boldsymbol{{abc}}\left(\boldsymbol{{a}}\:+\:\boldsymbol{{b}}\:+\:\boldsymbol{{c}}\right)\:\: \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:−\:\:\left(\boldsymbol{{ab}}\:+\:\boldsymbol{{bc}}\:+\boldsymbol{{ac}}\right)\left(\:\mathrm{4}\left(\boldsymbol{{a}}^{\mathrm{2}} +\:\boldsymbol{{b}}^{\mathrm{2}} +\:\boldsymbol{{c}}^{\mathrm{2}} \right)\:+\:\:\mathrm{6}\left(\boldsymbol{{ab}}\:+\:\boldsymbol{{bc}}\:+\:\boldsymbol{{ac}}\right)\right) \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\:\:\:\mathrm{3}^{\mathrm{4}} \:\:\:+\:\:\mathrm{4}\left(−\mathrm{1}\right)\left(\mathrm{3}\right)\:\:\:−\:\:\left(\mathrm{0}\right) \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\:\:\:\mathrm{81}\:\:\:−\:\:\:\mathrm{12} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\:\:\:\mathrm{69} \\ $$$$ \\ $$

Commented by Pk1167156@gmail.com last updated on 05/Dec/18

Thank you very much sir

Commented by afachri last updated on 05/Dec/18

ur welcome Sir

$$\mathrm{ur}\:\mathrm{welcome}\:\mathrm{Sir} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com