
Question Number 49183 by ajfour last updated on 04/Dec/18

Commented by ajfour last updated on 04/Dec/18

$${Find}\:\:\:\:\:\:\:\frac{{Area}\:\bigtriangleup{PQR}}{{Area}\:\bigtriangleup{ABC}}\:\:,\:{if}\:{centroid}\:{G} \\ $$$${of}\:\bigtriangleup{ABC}\:{lies}\:{on}\:{z}\:{axis}\:{and}\:{each} \\ $$$${vertex}\:{on}\:{surface}\:{of}\:{paraboloid}. \\ $$
Commented by mr W last updated on 04/Dec/18
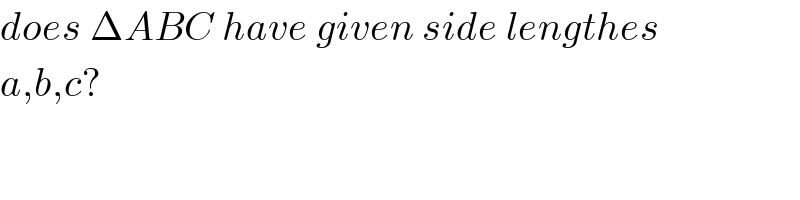
$${does}\:\Delta{ABC}\:{have}\:{given}\:{side}\:{lengthes} \\ $$$${a},{b},{c}? \\ $$
Commented by ajfour last updated on 04/Dec/18

$${yes}\:{sir}! \\ $$
Answered by mr W last updated on 05/Dec/18
![z=kr^2 with k=1 lengthes of medians of ΔABC: AG=(2/3)m_a =g_a =((√(2(b^2 +c^2 )−a^2 ))/3) BG=(2/3)m_b =g_b =((√(2(c^2 +a^2 )−b^2 ))/3) CG=(2/3)m_c =g_c =((√(2(a^2 +b^2 )−c^2 ))/3) let z_G =h let z_A =z_G +AG sin α=h+g_a sin α let z_B =z_G +BG sin β=h+g_b sin β we have three unknowns: h,α,β. r_A =AG cos α=g_a cos α r_B =BG cos β=g_b cos β z_A =kr_A ^2 ⇒h+g_a sin α=kg_a ^2 cos^2 α ⇒sin^2 α+(1/(kg_a )) sin α+(h/(kg_a ^2 ))−1=0 ...(i) similarly ⇒sin^2 β+(1/(kg_b )) sin β+(h/(kg_b ^2 ))−1=0 ...(ii) ⇒sin^2 γ+(1/(kg_c )) sin γ+(h/(kg_c ^2 ))−1=0 z_(B′) =z_G −B′G sin β=h−(1/3)m_b sin β z_(B′) =(1/2)(z_A +z_C ) z_C =2z_(B′) −z_A =2h−(2/3)m_b sin β−h−(2/3)m_a sin α ⇒z_C =h−(2/3)(m_a sin α+m_b sin β) z_C =h+(2/3)m_c sin γ ⇒(2/3)m_c sin γ=−(2/3)(m_a sin α+m_b sin β) ⇒ sin γ=−(1/g_c )(g_a sin α+g_b sin β) ⇒k(g_a sin α+g_b sin β)^2 −(g_a sin α+g_b sin β)+h−kg_c ^2 =0 ...(iii) from (i) and (ii): k(g_a ^2 sin^2 α−g_b ^2 sin^2 β)+(g_a sin α−g_b sin β)−k(g_a ^2 −g_b ^2 )=0 (1+kg_a sin α+kg_b sin β)(g_a sin α−g_b sin β)=k(g_a ^2 −g_b ^2 ) from (i) and (iii): k[g_a ^2 sin^2 α−(g_a sin α+g_b sin β)^2 ]+[g_a sin α+(g_a sin α+g_b sin β)]−k(g_a ^2 −g_c ^2 )=0 (1−kg_b sin β)(2g_a sin α+g_b sin β)=k(g_a ^2 −g_c ^2 ) we can solve (nummerically) { ((((1/k)+g_a sin α+g_b sin β)(g_a sin α−g_b sin β)=g_a ^2 −g_b ^2 )),((((1/k)−g_b sin β)(2g_a sin α+g_b sin β)=g_a ^2 −g_c ^2 )) :} for sin α and sin β. note: there can be more than one solution! with sin α and sin β we get sin γ from g_a sin α+g_b sin β+g_c sin γ=0 let e_a ,e_b ,e_c =projection of AG,BG,CG e_a =g_a cos α=g_a (√(1−sin^2 α)) e_b =g_b cos β=g_b (√(1−sin^2 β)) e_c =g_c cos γ=g_c (√(1−sin^2 γ)) Δ_(PQR) =((3(√((e_a +e_b +e_c )(−e_a +e_b +e_c )(e_a −e_b +e_c )(e_a +e_b −e_c ))))/4) Δ_(ABC) =((√((a+b+c)(−a+b+c)(a−b+c)(a+b−c)))/4) (Δ_(PQR) /Δ_(ABC) )=((3(√((e_a +e_b +e_c )(−e_a +e_b +e_c )(e_a −e_b +e_c )(e_a +e_b −e_c ))))/(√((a+b+c)(−a+b+c)(a−b+c)(a+b−c))))](Q49228.png)
$${z}={kr}^{\mathrm{2}} \:{with}\:{k}=\mathrm{1} \\ $$$$ \\ $$$${lengthes}\:{of}\:{medians}\:{of}\:\Delta{ABC}: \\ $$$${AG}=\frac{\mathrm{2}}{\mathrm{3}}{m}_{{a}} ={g}_{{a}} =\frac{\sqrt{\mathrm{2}\left({b}^{\mathrm{2}} +{c}^{\mathrm{2}} \right)−{a}^{\mathrm{2}} }}{\mathrm{3}} \\ $$$${BG}=\frac{\mathrm{2}}{\mathrm{3}}{m}_{{b}} ={g}_{{b}} =\frac{\sqrt{\mathrm{2}\left({c}^{\mathrm{2}} +{a}^{\mathrm{2}} \right)−{b}^{\mathrm{2}} }}{\mathrm{3}} \\ $$$${CG}=\frac{\mathrm{2}}{\mathrm{3}}{m}_{{c}} ={g}_{{c}} =\frac{\sqrt{\mathrm{2}\left({a}^{\mathrm{2}} +{b}^{\mathrm{2}} \right)−{c}^{\mathrm{2}} }}{\mathrm{3}} \\ $$$${let}\:{z}_{{G}} ={h} \\ $$$${let}\:{z}_{{A}} ={z}_{{G}} +{AG}\:\mathrm{sin}\:\alpha={h}+{g}_{{a}} \:\mathrm{sin}\:\alpha \\ $$$${let}\:{z}_{{B}} ={z}_{{G}} +{BG}\:\mathrm{sin}\:\beta={h}+{g}_{{b}} \:\mathrm{sin}\:\beta \\ $$$${we}\:{have}\:{three}\:{unknowns}:\:{h},\alpha,\beta. \\ $$$${r}_{{A}} ={AG}\:\mathrm{cos}\:\alpha={g}_{{a}} \:\mathrm{cos}\:\alpha \\ $$$${r}_{{B}} ={BG}\:\mathrm{cos}\:\beta={g}_{{b}} \:\mathrm{cos}\:\beta \\ $$$${z}_{{A}} ={kr}_{{A}} ^{\mathrm{2}} \\ $$$$\Rightarrow{h}+{g}_{{a}} \:\mathrm{sin}\:\alpha={kg}_{{a}} ^{\mathrm{2}} \:\mathrm{cos}^{\mathrm{2}} \:\alpha \\ $$$$\Rightarrow\mathrm{sin}^{\mathrm{2}} \:\alpha+\frac{\mathrm{1}}{{kg}_{{a}} }\:\mathrm{sin}\:\alpha+\frac{{h}}{{kg}_{{a}} ^{\mathrm{2}} }−\mathrm{1}=\mathrm{0}\:\:...\left({i}\right) \\ $$$${similarly} \\ $$$$\Rightarrow\mathrm{sin}^{\mathrm{2}} \:\beta+\frac{\mathrm{1}}{{kg}_{{b}} }\:\mathrm{sin}\:\beta+\frac{{h}}{{kg}_{{b}} ^{\mathrm{2}} }−\mathrm{1}=\mathrm{0}\:\:...\left({ii}\right) \\ $$$$\Rightarrow\mathrm{sin}^{\mathrm{2}} \:\gamma+\frac{\mathrm{1}}{{kg}_{{c}} }\:\mathrm{sin}\:\gamma+\frac{{h}}{{kg}_{{c}} ^{\mathrm{2}} }−\mathrm{1}=\mathrm{0} \\ $$$${z}_{{B}'} ={z}_{{G}} −{B}'{G}\:\mathrm{sin}\:\beta={h}−\frac{\mathrm{1}}{\mathrm{3}}{m}_{{b}} \:\mathrm{sin}\:\beta \\ $$$${z}_{{B}'} =\frac{\mathrm{1}}{\mathrm{2}}\left({z}_{{A}} +{z}_{{C}} \right) \\ $$$${z}_{{C}} =\mathrm{2}{z}_{{B}'} −{z}_{{A}} =\mathrm{2}{h}−\frac{\mathrm{2}}{\mathrm{3}}{m}_{{b}} \:\mathrm{sin}\:\beta−{h}−\frac{\mathrm{2}}{\mathrm{3}}{m}_{{a}} \:\mathrm{sin}\:\alpha \\ $$$$\Rightarrow{z}_{{C}} ={h}−\frac{\mathrm{2}}{\mathrm{3}}\left({m}_{{a}} \:\mathrm{sin}\:\alpha+{m}_{{b}} \:\mathrm{sin}\:\beta\right) \\ $$$${z}_{{C}} ={h}+\frac{\mathrm{2}}{\mathrm{3}}{m}_{{c}} \:\mathrm{sin}\:\gamma \\ $$$$\Rightarrow\frac{\mathrm{2}}{\mathrm{3}}{m}_{{c}} \:\mathrm{sin}\:\gamma=−\frac{\mathrm{2}}{\mathrm{3}}\left({m}_{{a}} \:\mathrm{sin}\:\alpha+{m}_{{b}} \:\mathrm{sin}\:\beta\right) \\ $$$$\Rightarrow\:\mathrm{sin}\:\gamma=−\frac{\mathrm{1}}{{g}_{{c}} }\left({g}_{{a}} \:\mathrm{sin}\:\alpha+{g}_{{b}} \:\mathrm{sin}\:\beta\right) \\ $$$$\Rightarrow{k}\left({g}_{{a}} \:\mathrm{sin}\:\alpha+{g}_{{b}} \:\mathrm{sin}\:\beta\right)^{\mathrm{2}} −\left({g}_{{a}} \:\mathrm{sin}\:\alpha+{g}_{{b}} \:\mathrm{sin}\:\beta\right)+{h}−{kg}_{{c}} ^{\mathrm{2}} =\mathrm{0}\:\:\:...\left({iii}\right) \\ $$$$ \\ $$$${from}\:\left({i}\right)\:{and}\:\left({ii}\right): \\ $$$${k}\left({g}_{{a}} ^{\mathrm{2}} \mathrm{sin}^{\mathrm{2}} \:\alpha−{g}_{{b}} ^{\mathrm{2}} \mathrm{sin}^{\mathrm{2}} \:\beta\right)+\left({g}_{{a}} \mathrm{sin}\:\alpha−{g}_{{b}} \mathrm{sin}\:\beta\right)−{k}\left({g}_{{a}} ^{\mathrm{2}} −{g}_{{b}} ^{\mathrm{2}} \right)=\mathrm{0} \\ $$$$\left(\mathrm{1}+{kg}_{{a}} \mathrm{sin}\:\alpha+{kg}_{{b}} \mathrm{sin}\:\beta\right)\left({g}_{{a}} \mathrm{sin}\:\alpha−{g}_{{b}} \mathrm{sin}\:\beta\right)={k}\left({g}_{{a}} ^{\mathrm{2}} −{g}_{{b}} ^{\mathrm{2}} \right) \\ $$$${from}\:\left({i}\right)\:{and}\:\left({iii}\right): \\ $$$${k}\left[{g}_{{a}} ^{\mathrm{2}} \mathrm{sin}^{\mathrm{2}} \:\alpha−\left({g}_{{a}} \:\mathrm{sin}\:\alpha+{g}_{{b}} \:\mathrm{sin}\:\beta\right)^{\mathrm{2}} \right]+\left[{g}_{{a}} \mathrm{sin}\:\alpha+\left({g}_{{a}} \:\mathrm{sin}\:\alpha+{g}_{{b}} \:\mathrm{sin}\:\beta\right)\right]−{k}\left({g}_{{a}} ^{\mathrm{2}} −{g}_{{c}} ^{\mathrm{2}} \right)=\mathrm{0} \\ $$$$\left(\mathrm{1}−{kg}_{{b}} \:\mathrm{sin}\:\beta\right)\left(\mathrm{2}{g}_{{a}} \mathrm{sin}\:\alpha+{g}_{{b}} \:\mathrm{sin}\:\beta\right)={k}\left({g}_{{a}} ^{\mathrm{2}} −{g}_{{c}} ^{\mathrm{2}} \right) \\ $$$${we}\:{can}\:{solve}\:\left({nummerically}\right) \\ $$$$\begin{cases}{\left(\frac{\mathrm{1}}{{k}}+{g}_{{a}} \mathrm{sin}\:\alpha+{g}_{{b}} \mathrm{sin}\:\beta\right)\left({g}_{{a}} \mathrm{sin}\:\alpha−{g}_{{b}} \mathrm{sin}\:\beta\right)={g}_{{a}} ^{\mathrm{2}} −{g}_{{b}} ^{\mathrm{2}} }\\{\left(\frac{\mathrm{1}}{{k}}−{g}_{{b}} \:\mathrm{sin}\:\beta\right)\left(\mathrm{2}{g}_{{a}} \mathrm{sin}\:\alpha+{g}_{{b}} \:\mathrm{sin}\:\beta\right)={g}_{{a}} ^{\mathrm{2}} −{g}_{{c}} ^{\mathrm{2}} }\end{cases} \\ $$$${for}\:\mathrm{sin}\:\alpha\:{and}\:\mathrm{sin}\:\beta. \\ $$$${note}:\:{there}\:{can}\:{be}\:{more}\:{than}\:{one} \\ $$$${solution}! \\ $$$${with}\:\mathrm{sin}\:\alpha\:{and}\:\mathrm{sin}\:\beta\:{we}\:{get}\:\mathrm{sin}\:\gamma\:{from} \\ $$$${g}_{{a}} \:\mathrm{sin}\:\alpha+{g}_{{b}} \:\mathrm{sin}\:\beta+{g}_{{c}} \mathrm{sin}\:\gamma=\mathrm{0} \\ $$$$ \\ $$$${let}\:{e}_{{a}} ,{e}_{{b}} ,{e}_{{c}} ={projection}\:{of}\:{AG},{BG},{CG} \\ $$$${e}_{{a}} ={g}_{{a}} \:\mathrm{cos}\:\alpha={g}_{{a}} \sqrt{\mathrm{1}−\mathrm{sin}^{\mathrm{2}} \:\alpha} \\ $$$${e}_{{b}} ={g}_{{b}} \:\mathrm{cos}\:\beta={g}_{{b}} \sqrt{\mathrm{1}−\mathrm{sin}^{\mathrm{2}} \:\beta} \\ $$$${e}_{{c}} ={g}_{{c}} \:\mathrm{cos}\:\gamma={g}_{{c}} \sqrt{\mathrm{1}−\mathrm{sin}^{\mathrm{2}} \:\gamma} \\ $$$$\Delta_{{PQR}} =\frac{\mathrm{3}\sqrt{\left({e}_{{a}} +{e}_{{b}} +{e}_{{c}} \right)\left(−{e}_{{a}} +{e}_{{b}} +{e}_{{c}} \right)\left({e}_{{a}} −{e}_{{b}} +{e}_{{c}} \right)\left({e}_{{a}} +{e}_{{b}} −{e}_{{c}} \right)}}{\mathrm{4}} \\ $$$$\Delta_{{ABC}} =\frac{\sqrt{\left({a}+{b}+{c}\right)\left(−{a}+{b}+{c}\right)\left({a}−{b}+{c}\right)\left({a}+{b}−{c}\right)}}{\mathrm{4}} \\ $$$$\frac{\Delta_{{PQR}} }{\Delta_{{ABC}} }=\frac{\mathrm{3}\sqrt{\left({e}_{{a}} +{e}_{{b}} +{e}_{{c}} \right)\left(−{e}_{{a}} +{e}_{{b}} +{e}_{{c}} \right)\left({e}_{{a}} −{e}_{{b}} +{e}_{{c}} \right)\left({e}_{{a}} +{e}_{{b}} −{e}_{{c}} \right)}}{\sqrt{\left({a}+{b}+{c}\right)\left(−{a}+{b}+{c}\right)\left({a}−{b}+{c}\right)\left({a}+{b}−{c}\right)}} \\ $$
Commented by mr W last updated on 04/Dec/18

Commented by ajfour last updated on 06/Dec/18

$${Thanks}\:{sir}!\:{and}\:{sorry}\:{for}\:{such} \\ $$$${not}\:{so}\:{interesting}\:{question}.. \\ $$
Commented by mr W last updated on 06/Dec/18

$${but}\:{it}\:{is}\:{an}\:{interesting}\:{question}.\:{what} \\ $$$${is}\:{the}\:{reason}\:{for}\:{the}\:{restriction}\:{that} \\ $$$${the}\:{centroid}\:{should}\:{lie}\:{on}\:{z}−{axix}?\: \\ $$$${with}\:{this}\:{restriction}\:{not}\:{every}\:{triangle} \\ $$$${can}\:{be}\:{placed}\:{inside}\:{the}\:{cup},\:{for} \\ $$$${example}\:{a}\:{triangle}\:{with}\:{sides}\:\mathrm{3},\mathrm{4},\mathrm{5}. \\ $$
