
Question Number 48871 by peter frank last updated on 29/Nov/18

Commented by maxmathsup by imad last updated on 01/Jan/19
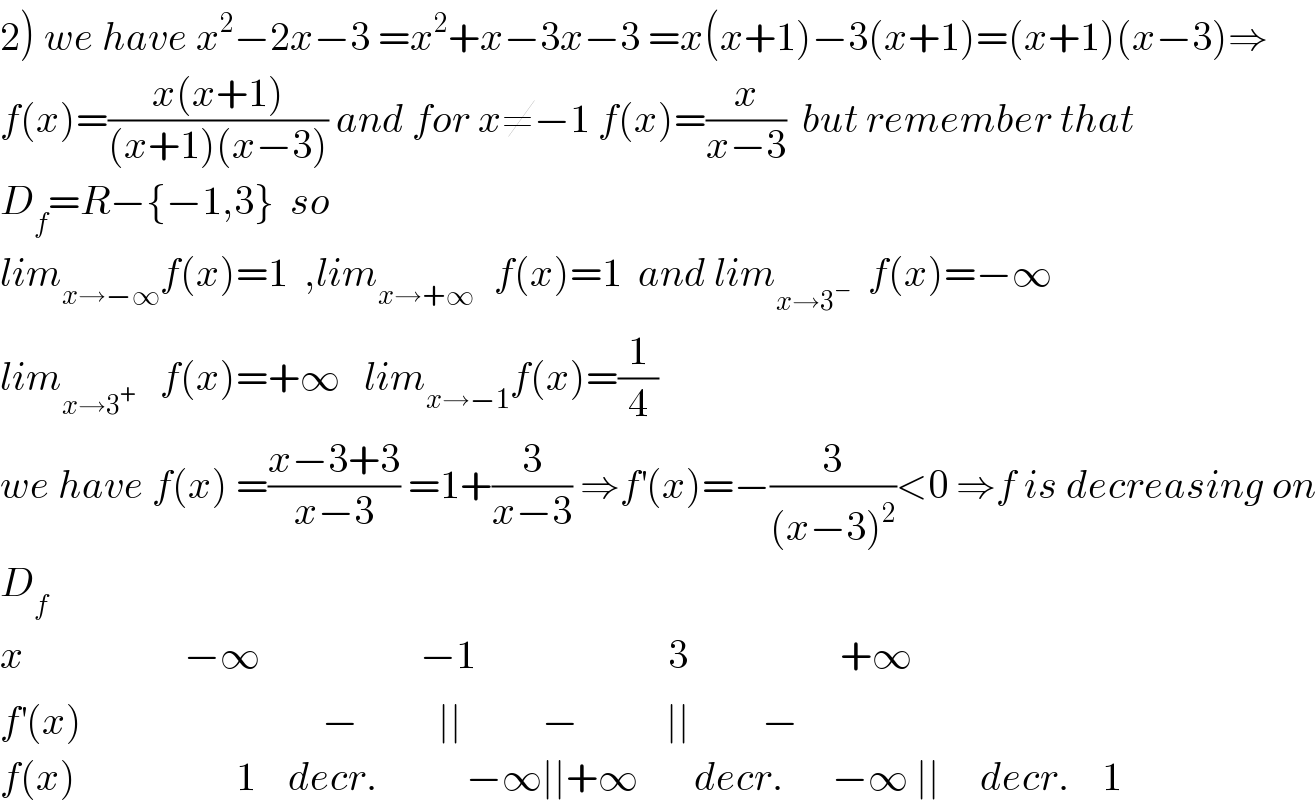
$$\left.\mathrm{2}\right)\:{we}\:{have}\:{x}^{\mathrm{2}} −\mathrm{2}{x}−\mathrm{3}\:={x}^{\mathrm{2}} +{x}−\mathrm{3}{x}−\mathrm{3}\:={x}\left({x}+\mathrm{1}\right)−\mathrm{3}\left({x}+\mathrm{1}\right)=\left({x}+\mathrm{1}\right)\left({x}−\mathrm{3}\right)\Rightarrow \\ $$$${f}\left({x}\right)=\frac{{x}\left({x}+\mathrm{1}\right)}{\left({x}+\mathrm{1}\right)\left({x}−\mathrm{3}\right)}\:{and}\:{for}\:{x}\neq−\mathrm{1}\:{f}\left({x}\right)=\frac{{x}}{{x}−\mathrm{3}}\:\:{but}\:{remember}\:{that} \\ $$$${D}_{{f}} ={R}−\left\{−\mathrm{1},\mathrm{3}\right\}\:\:{so} \\ $$$${lim}_{{x}\rightarrow−\infty} {f}\left({x}\right)=\mathrm{1}\:\:,{lim}_{{x}\rightarrow+\infty\:} \:\:{f}\left({x}\right)=\mathrm{1}\:\:{and}\:{lim}_{{x}\rightarrow\mathrm{3}^{−} } \:\:{f}\left({x}\right)=−\infty\: \\ $$$${lim}_{{x}\rightarrow\mathrm{3}^{+} } \:\:\:{f}\left({x}\right)=+\infty\:\:\:{lim}_{{x}\rightarrow−\mathrm{1}} {f}\left({x}\right)=\frac{\mathrm{1}}{\mathrm{4}} \\ $$$${we}\:{have}\:{f}\left({x}\right)\:=\frac{{x}−\mathrm{3}+\mathrm{3}}{{x}−\mathrm{3}}\:=\mathrm{1}+\frac{\mathrm{3}}{{x}−\mathrm{3}}\:\Rightarrow{f}^{'} \left({x}\right)=−\frac{\mathrm{3}}{\left({x}−\mathrm{3}\right)^{\mathrm{2}} }<\mathrm{0}\:\Rightarrow{f}\:{is}\:{decreasing}\:{on} \\ $$$${D}_{{f}} \\ $$$${x}\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:−\infty\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:−\mathrm{1}\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{3}\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:+\infty \\ $$$${f}^{'} \left({x}\right)\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:−\:\:\:\:\:\:\:\:\:\:\mid\mid\:\:\:\:\:\:\:\:\:\:−\:\:\:\:\:\:\:\:\:\:\:\mid\mid\:\:\:\:\:\:\:\:\:− \\ $$$${f}\left({x}\right)\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{1}\:\:\:\:{decr}.\:\:\:\:\:\:\:\:\:\:\:−\infty\mid\mid+\infty\:\:\:\:\:\:\:{decr}.\:\:\:\:\:\:−\infty\:\mid\mid\:\:\:\:\:{decr}.\:\:\:\:\mathrm{1} \\ $$
Answered by kaivan.ahmadi last updated on 30/Nov/18
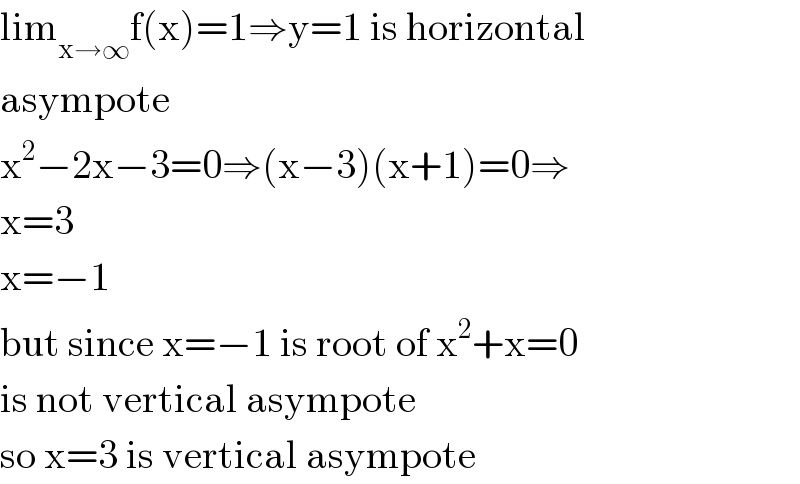
$$\mathrm{lim}_{\mathrm{x}\rightarrow\infty} \mathrm{f}\left(\mathrm{x}\right)=\mathrm{1}\Rightarrow\mathrm{y}=\mathrm{1}\:\mathrm{is}\:\mathrm{horizontal} \\ $$$$\mathrm{asympote} \\ $$$$\mathrm{x}^{\mathrm{2}} −\mathrm{2x}−\mathrm{3}=\mathrm{0}\Rightarrow\left(\mathrm{x}−\mathrm{3}\right)\left(\mathrm{x}+\mathrm{1}\right)=\mathrm{0}\Rightarrow \\ $$$$\mathrm{x}=\mathrm{3} \\ $$$$\mathrm{x}=−\mathrm{1} \\ $$$$\mathrm{but}\:\mathrm{since}\:\mathrm{x}=−\mathrm{1}\:\mathrm{is}\:\mathrm{root}\:\mathrm{of}\:\mathrm{x}^{\mathrm{2}} +\mathrm{x}=\mathrm{0} \\ $$$$\mathrm{is}\:\mathrm{not}\:\mathrm{vertical}\:\mathrm{asympote} \\ $$$$\mathrm{so}\:\mathrm{x}=\mathrm{3}\:\mathrm{is}\:\mathrm{vertical}\:\mathrm{asympote} \\ $$
Answered by kaivan.ahmadi last updated on 30/Nov/18

$$\mathrm{D}_{\mathrm{f}} =\mathrm{R}−\left\{−\mathrm{1},\mathrm{3}\right\} \\ $$$$\mathrm{R}_{\mathrm{f}} =\mathrm{R}−\left\{\mathrm{1}\right\} \\ $$
Answered by kaivan.ahmadi last updated on 30/Nov/18
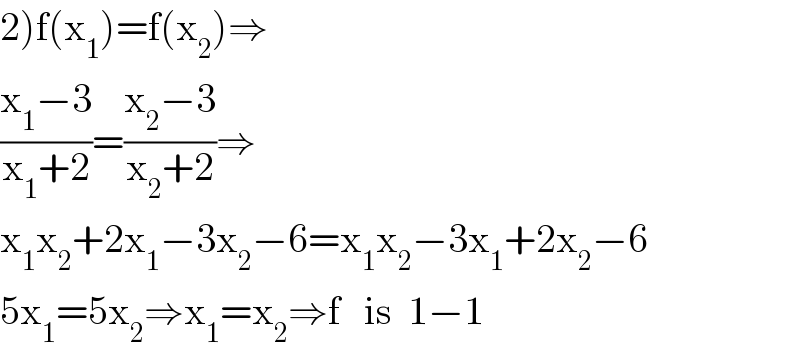
$$\left.\mathrm{2}\right)\mathrm{f}\left(\mathrm{x}_{\mathrm{1}} \right)=\mathrm{f}\left(\mathrm{x}_{\mathrm{2}} \right)\Rightarrow \\ $$$$\frac{\mathrm{x}_{\mathrm{1}} −\mathrm{3}}{\mathrm{x}_{\mathrm{1}} +\mathrm{2}}=\frac{\mathrm{x}_{\mathrm{2}} −\mathrm{3}}{\mathrm{x}_{\mathrm{2}} +\mathrm{2}}\Rightarrow \\ $$$$\mathrm{x}_{\mathrm{1}} \mathrm{x}_{\mathrm{2}} +\mathrm{2x}_{\mathrm{1}} −\mathrm{3x}_{\mathrm{2}} −\mathrm{6}=\mathrm{x}_{\mathrm{1}} \mathrm{x}_{\mathrm{2}} −\mathrm{3x}_{\mathrm{1}} +\mathrm{2x}_{\mathrm{2}} −\mathrm{6} \\ $$$$\mathrm{5x}_{\mathrm{1}} =\mathrm{5x}_{\mathrm{2}} \Rightarrow\mathrm{x}_{\mathrm{1}} =\mathrm{x}_{\mathrm{2}} \Rightarrow\mathrm{f}\:\:\:\mathrm{is}\:\:\mathrm{1}−\mathrm{1} \\ $$
