
Question Number 47939 by rahul 19 last updated on 17/Nov/18

Commented by rahul 19 last updated on 17/Nov/18
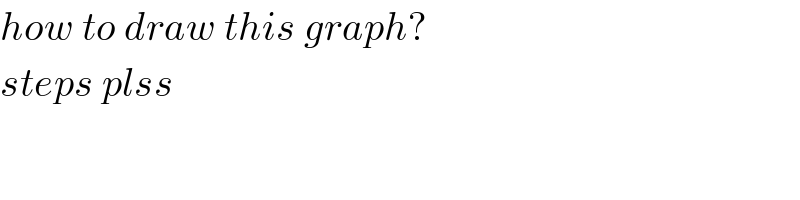
$${how}\:{to}\:{draw}\:{this}\:{graph}? \\ $$$${steps}\:{plss} \\ $$
Commented by rahul 19 last updated on 17/Nov/18
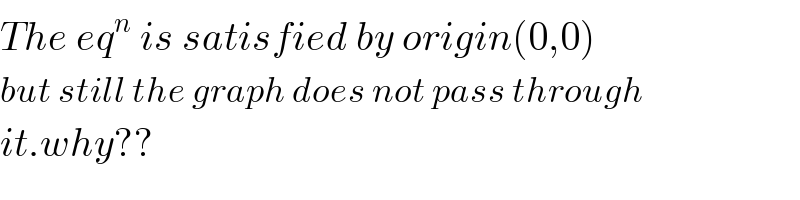
$${The}\:{eq}^{{n}} \:{is}\:{satisfied}\:{by}\:{origin}\left(\mathrm{0},\mathrm{0}\right) \\ $$$${but}\:{still}\:{the}\:{graph}\:{does}\:{not}\:{pass}\:{through} \\ $$$${it}.{why}?? \\ $$
Answered by mr W last updated on 17/Nov/18
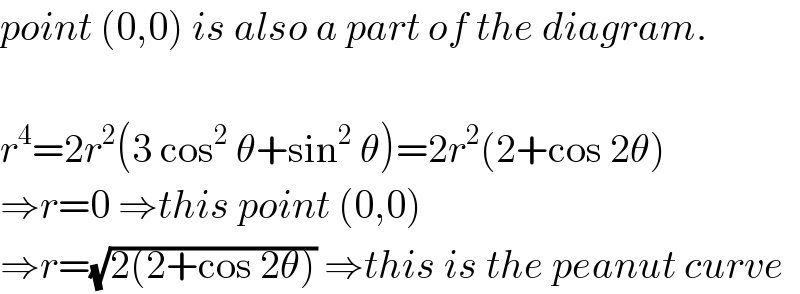
$${point}\:\left(\mathrm{0},\mathrm{0}\right)\:{is}\:{also}\:{a}\:{part}\:{of}\:{the}\:{diagram}. \\ $$$$ \\ $$$${r}^{\mathrm{4}} =\mathrm{2}{r}^{\mathrm{2}} \left(\mathrm{3}\:\mathrm{cos}^{\mathrm{2}} \:\theta+\mathrm{sin}^{\mathrm{2}} \:\theta\right)=\mathrm{2}{r}^{\mathrm{2}} \left(\mathrm{2}+\mathrm{cos}\:\mathrm{2}\theta\right) \\ $$$$\Rightarrow{r}=\mathrm{0}\:\Rightarrow{this}\:{point}\:\left(\mathrm{0},\mathrm{0}\right) \\ $$$$\Rightarrow{r}=\sqrt{\mathrm{2}\left(\mathrm{2}+\mathrm{cos}\:\mathrm{2}\theta\right)}\:\Rightarrow{this}\:{is}\:{the}\:{peanut}\:{curve} \\ $$
Commented by rahul 19 last updated on 17/Nov/18
thanks sir.
