
Question Number 47150 by somil last updated on 05/Nov/18
Commented by maxmathsup by imad last updated on 05/Nov/18
![we see that cosθ must be ≥0⇒θ ∈[−(π/2),(π/2)][2π] changement =x =sinθ give 3(√(1−x^2 ))=2x^2 ⇒9(1−x^2 )=4x^4 ⇒4x^4 +9x^2 −9=0 x^2 =u ⇒4u^2 +9u−9=0 ⇒Δ=9^2 −4.4.(−9) =9(9 +16)=9.25 ⇒(√Δ)=15 u_1 =((−9+15)/8) =(6/8) =(3/4) and u_2 =((−9−15)/8) =−3<0(to eliminate) ⇒ x^2 =(3/4) ⇒x=+^− ((√3)/2) case 1 x=((√3)/2) ⇒sinθ =sin((π/3)) ⇒ θ =(π/3) +2kπ or θ =((2π)/3) +2kπ −(π/2)≤(π/3)+2kπ≤(π/2) ⇒−(1/2)≤(1/3) +2k≤(1/2) ⇒−(5/3) ≤2k≤(1/6) ⇒−(5/6)≤k≤(1/(12)) ⇒k=0 ⇒θ =(π/3) −(π/2)≤((2π)/3) +2kπ≤(π/2) ⇒−(1/2) ≤(2/3) +2k≤(1/2) ⇒((−7)/3) ≤2k≤−(1/6) ⇒ −(7/6)≤k≤−(1/(12)) ⇒k=−1 ⇒x=((2π)/3) −2π =−((4π)/3) and cos(((4π)/3))<0 (toeliminate) case 2 x =−((√3)/2) ⇒sinθ =sin(−(π/3)) ⇒θ =−(π/3) +2kπ or θ=((4π)/3) +2kπ we follow the same manner to find x ...](Q47156.png)
$${we}\:{see}\:{that}\:{cos}\theta\:{must}\:{be}\:\geqslant\mathrm{0}\Rightarrow\theta\:\in\left[−\frac{\pi}{\mathrm{2}},\frac{\pi}{\mathrm{2}}\right]\left[\mathrm{2}\pi\right]\:\:{changement}\:={x}\:={sin}\theta\:{give} \\ $$$$\mathrm{3}\sqrt{\mathrm{1}−{x}^{\mathrm{2}} }=\mathrm{2}{x}^{\mathrm{2}} \:\Rightarrow\mathrm{9}\left(\mathrm{1}−{x}^{\mathrm{2}} \right)=\mathrm{4}{x}^{\mathrm{4}} \:\Rightarrow\mathrm{4}{x}^{\mathrm{4}} +\mathrm{9}{x}^{\mathrm{2}} \:−\mathrm{9}=\mathrm{0} \\ $$$${x}^{\mathrm{2}} ={u}\:\Rightarrow\mathrm{4}{u}^{\mathrm{2}} \:+\mathrm{9}{u}−\mathrm{9}=\mathrm{0}\:\Rightarrow\Delta=\mathrm{9}^{\mathrm{2}} −\mathrm{4}.\mathrm{4}.\left(−\mathrm{9}\right)\:=\mathrm{9}\left(\mathrm{9}\:+\mathrm{16}\right)=\mathrm{9}.\mathrm{25}\:\Rightarrow\sqrt{\Delta}=\mathrm{15} \\ $$$${u}_{\mathrm{1}} =\frac{−\mathrm{9}+\mathrm{15}}{\mathrm{8}}\:=\frac{\mathrm{6}}{\mathrm{8}}\:=\frac{\mathrm{3}}{\mathrm{4}}\:{and}\:{u}_{\mathrm{2}} =\frac{−\mathrm{9}−\mathrm{15}}{\mathrm{8}}\:=−\mathrm{3}<\mathrm{0}\left({to}\:{eliminate}\right)\:\Rightarrow \\ $$$${x}^{\mathrm{2}} =\frac{\mathrm{3}}{\mathrm{4}}\:\Rightarrow{x}=\overset{−} {+}\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\:\:\: \\ $$$${case}\:\mathrm{1}\:\:\:{x}=\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\:\Rightarrow{sin}\theta\:={sin}\left(\frac{\pi}{\mathrm{3}}\right)\:\Rightarrow\:\theta\:=\frac{\pi}{\mathrm{3}}\:+\mathrm{2}{k}\pi\:{or}\:\theta\:=\frac{\mathrm{2}\pi}{\mathrm{3}}\:+\mathrm{2}{k}\pi \\ $$$$−\frac{\pi}{\mathrm{2}}\leqslant\frac{\pi}{\mathrm{3}}+\mathrm{2}{k}\pi\leqslant\frac{\pi}{\mathrm{2}}\:\Rightarrow−\frac{\mathrm{1}}{\mathrm{2}}\leqslant\frac{\mathrm{1}}{\mathrm{3}}\:+\mathrm{2}{k}\leqslant\frac{\mathrm{1}}{\mathrm{2}}\:\Rightarrow−\frac{\mathrm{5}}{\mathrm{3}}\:\leqslant\mathrm{2}{k}\leqslant\frac{\mathrm{1}}{\mathrm{6}}\:\Rightarrow−\frac{\mathrm{5}}{\mathrm{6}}\leqslant{k}\leqslant\frac{\mathrm{1}}{\mathrm{12}} \\ $$$$\Rightarrow{k}=\mathrm{0}\:\Rightarrow\theta\:=\frac{\pi}{\mathrm{3}} \\ $$$$−\frac{\pi}{\mathrm{2}}\leqslant\frac{\mathrm{2}\pi}{\mathrm{3}}\:+\mathrm{2}{k}\pi\leqslant\frac{\pi}{\mathrm{2}}\:\Rightarrow−\frac{\mathrm{1}}{\mathrm{2}}\:\leqslant\frac{\mathrm{2}}{\mathrm{3}}\:+\mathrm{2}{k}\leqslant\frac{\mathrm{1}}{\mathrm{2}}\:\Rightarrow\frac{−\mathrm{7}}{\mathrm{3}}\:\leqslant\mathrm{2}{k}\leqslant−\frac{\mathrm{1}}{\mathrm{6}}\:\Rightarrow \\ $$$$−\frac{\mathrm{7}}{\mathrm{6}}\leqslant{k}\leqslant−\frac{\mathrm{1}}{\mathrm{12}}\:\Rightarrow{k}=−\mathrm{1}\:\Rightarrow{x}=\frac{\mathrm{2}\pi}{\mathrm{3}}\:−\mathrm{2}\pi\:=−\frac{\mathrm{4}\pi}{\mathrm{3}}\:\:{and}\:{cos}\left(\frac{\mathrm{4}\pi}{\mathrm{3}}\right)<\mathrm{0}\:\left({toeliminate}\right) \\ $$$${case}\:\mathrm{2}\:\:{x}\:=−\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\:\Rightarrow{sin}\theta\:={sin}\left(−\frac{\pi}{\mathrm{3}}\right)\:\Rightarrow\theta\:=−\frac{\pi}{\mathrm{3}}\:+\mathrm{2}{k}\pi\:{or}\:\theta=\frac{\mathrm{4}\pi}{\mathrm{3}}\:+\mathrm{2}{k}\pi \\ $$$${we}\:{follow}\:{the}\:{same}\:{manner}\:{to}\:{find}\:{x}\:... \\ $$$$ \\ $$
Commented by maxmathsup by imad last updated on 05/Nov/18
![another method but easy (e) ⇔ 3cosθ =2(1−cos^2 θ) ⇒ 3cosθ−2 +2cos^2 θ =0 ⇒2 cos^2 θ +3cosθ −2 =0 with condition cosθ≥0 ⇒ θ ∈[−(π/2),(π/2)] changement cosθ =x drive to 2 x^2 +3x−2 =0 Δ =9−4.2(−2) =9+16 =25 ⇒x_1 =((−3+5)/4) =(1/2) and x_2 =((−3−5)/4) <0(to eliminate) after we solve the equation cosθ =(1/2) ....](Q47158.png)
$${another}\:{method}\:{but}\:{easy}\:\:\left({e}\right)\:\Leftrightarrow\:\mathrm{3}{cos}\theta\:=\mathrm{2}\left(\mathrm{1}−{cos}^{\mathrm{2}} \theta\right)\:\Rightarrow \\ $$$$\mathrm{3}{cos}\theta−\mathrm{2}\:+\mathrm{2}{cos}^{\mathrm{2}} \theta\:=\mathrm{0}\:\Rightarrow\mathrm{2}\:{cos}^{\mathrm{2}} \theta\:+\mathrm{3}{cos}\theta\:−\mathrm{2}\:=\mathrm{0}\:\:{with}\:{condition}\:{cos}\theta\geqslant\mathrm{0}\:\Rightarrow \\ $$$$\theta\:\in\left[−\frac{\pi}{\mathrm{2}},\frac{\pi}{\mathrm{2}}\right]\:\:{changement}\:{cos}\theta\:={x}\:{drive}\:{to}\:\mathrm{2}\:{x}^{\mathrm{2}} \:+\mathrm{3}{x}−\mathrm{2}\:=\mathrm{0} \\ $$$$\Delta\:=\mathrm{9}−\mathrm{4}.\mathrm{2}\left(−\mathrm{2}\right)\:=\mathrm{9}+\mathrm{16}\:=\mathrm{25}\:\Rightarrow{x}_{\mathrm{1}} =\frac{−\mathrm{3}+\mathrm{5}}{\mathrm{4}}\:=\frac{\mathrm{1}}{\mathrm{2}}\:{and}\:{x}_{\mathrm{2}} =\frac{−\mathrm{3}−\mathrm{5}}{\mathrm{4}}\:<\mathrm{0}\left({to}\:{eliminate}\right) \\ $$$${after}\:{we}\:{solve}\:{the}\:{equation}\:{cos}\theta\:=\frac{\mathrm{1}}{\mathrm{2}}\:.... \\ $$
Answered by tanmay.chaudhury50@gmail.com last updated on 05/Nov/18
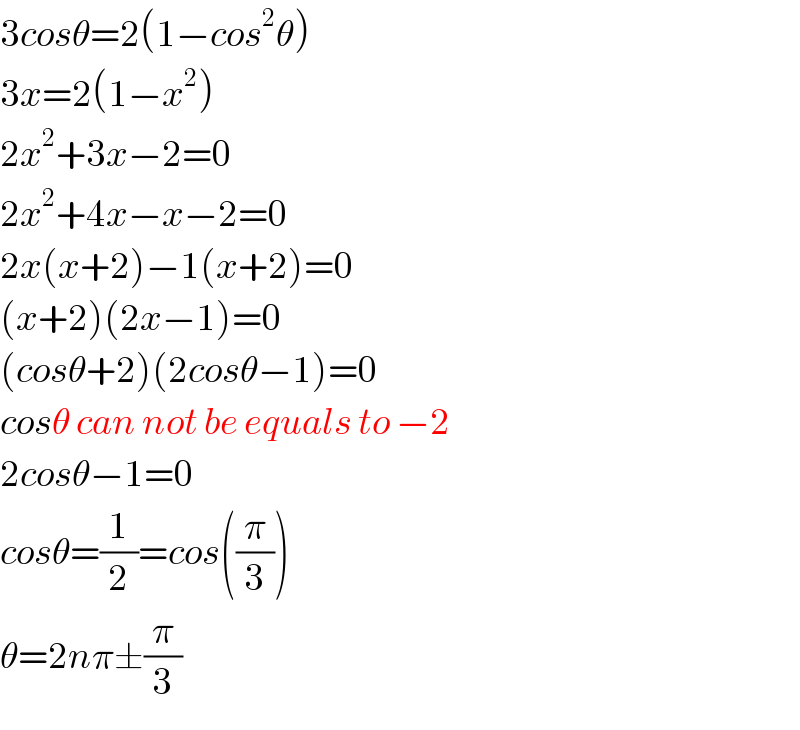
$$\mathrm{3}{cos}\theta=\mathrm{2}\left(\mathrm{1}−{cos}^{\mathrm{2}} \theta\right) \\ $$$$\mathrm{3}{x}=\mathrm{2}\left(\mathrm{1}−{x}^{\mathrm{2}} \right) \\ $$$$\mathrm{2}{x}^{\mathrm{2}} +\mathrm{3}{x}−\mathrm{2}=\mathrm{0} \\ $$$$\mathrm{2}{x}^{\mathrm{2}} +\mathrm{4}{x}−{x}−\mathrm{2}=\mathrm{0} \\ $$$$\mathrm{2}{x}\left({x}+\mathrm{2}\right)−\mathrm{1}\left({x}+\mathrm{2}\right)=\mathrm{0} \\ $$$$\left({x}+\mathrm{2}\right)\left(\mathrm{2}{x}−\mathrm{1}\right)=\mathrm{0} \\ $$$$\left({cos}\theta+\mathrm{2}\right)\left(\mathrm{2}{cos}\theta−\mathrm{1}\right)=\mathrm{0} \\ $$$${cos}\theta\:{can}\:{not}\:{be}\:{equals}\:{to}\:−\mathrm{2} \\ $$$$\mathrm{2}{cos}\theta−\mathrm{1}=\mathrm{0} \\ $$$${cos}\theta=\frac{\mathrm{1}}{\mathrm{2}}={cos}\left(\frac{\pi}{\mathrm{3}}\right) \\ $$$$\theta=\mathrm{2}{n}\pi\pm\frac{\pi}{\mathrm{3}} \\ $$
