
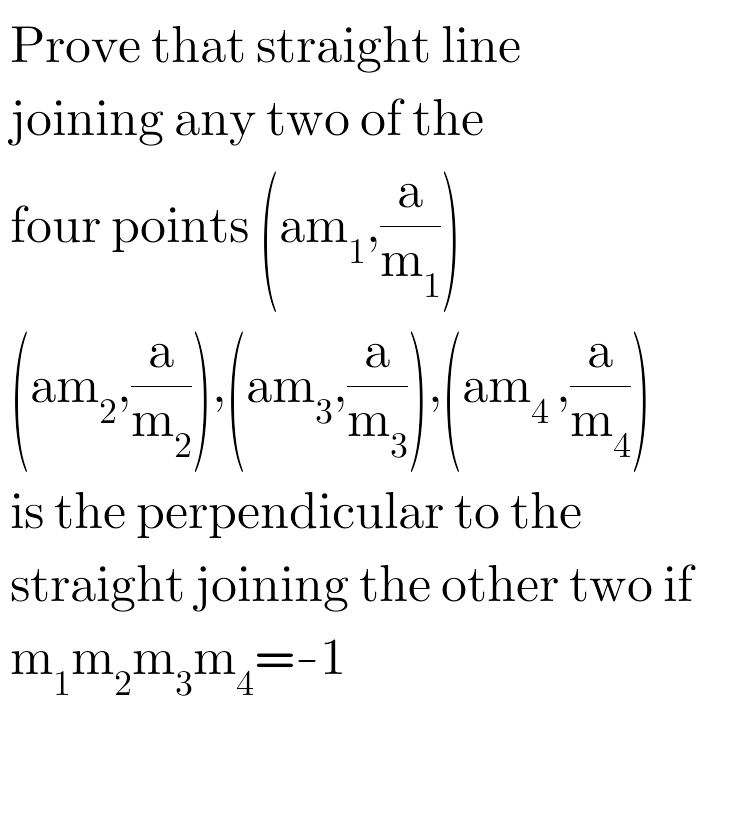
Question Number 46816 by peter frank last updated on 31/Oct/18
Answered by tanmay.chaudhury50@gmail.com last updated on 01/Nov/18
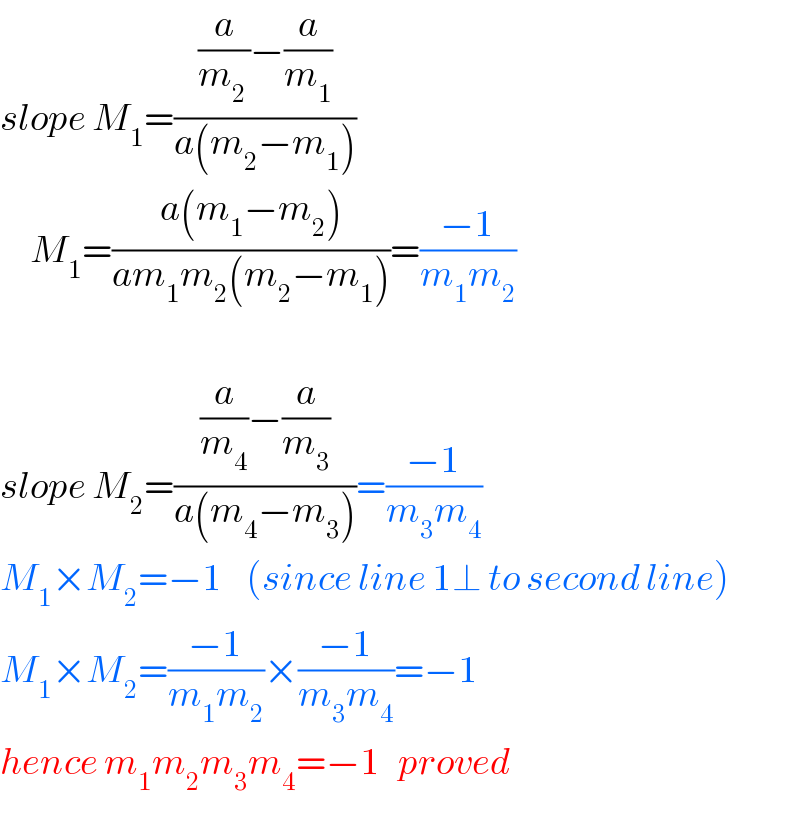
$${slope}\:{M}_{\mathrm{1}} =\frac{\frac{{a}}{{m}_{\mathrm{2}\:} }−\frac{{a}}{{m}_{\mathrm{1}} }}{{a}\left({m}_{\mathrm{2}} −{m}_{\mathrm{1}} \right)} \\ $$$$\:\:\:\:\:{M}_{\mathrm{1}} =\frac{{a}\left({m}_{\mathrm{1}} −{m}_{\mathrm{2}} \right)}{{am}_{\mathrm{1}} {m}_{\mathrm{2}} \left({m}_{\mathrm{2}} −{m}_{\mathrm{1}} \right)}=\frac{−\mathrm{1}}{{m}_{\mathrm{1}} {m}_{\mathrm{2}} } \\ $$$$ \\ $$$${slope}\:{M}_{\mathrm{2}} =\frac{\frac{{a}}{{m}_{\mathrm{4}} }−\frac{{a}}{{m}_{\mathrm{3}} }}{{a}\left({m}_{\mathrm{4}} −{m}_{\mathrm{3}} \right)}=\frac{−\mathrm{1}}{{m}_{\mathrm{3}} {m}_{\mathrm{4}} } \\ $$$${M}_{\mathrm{1}} ×{M}_{\mathrm{2}} =−\mathrm{1}\:\:\:\:\left({since}\:{line}\:\mathrm{1}\bot\:{to}\:{second}\:{line}\right) \\ $$$${M}_{\mathrm{1}} ×{M}_{\mathrm{2}} =\frac{−\mathrm{1}}{{m}_{\mathrm{1}} {m}_{\mathrm{2}} }×\frac{−\mathrm{1}}{{m}_{\mathrm{3}} {m}_{\mathrm{4}} }=−\mathrm{1} \\ $$$${hence}\:{m}_{\mathrm{1}} {m}_{\mathrm{2}} {m}_{\mathrm{3}} {m}_{\mathrm{4}} =−\mathrm{1}\:\:\:{proved} \\ $$
