Question and Answers Forum

All Questions      Topic List

Vector Questions

Previous in All Question      Next in All Question      

Previous in Vector      Next in Vector      

Question Number 45809 by rahul 19 last updated on 17/Oct/18

Commented by rahul 19 last updated on 17/Oct/18

Calculation error....

$${Calculation}\:{error}.... \\ $$

Commented by MJS last updated on 17/Oct/18

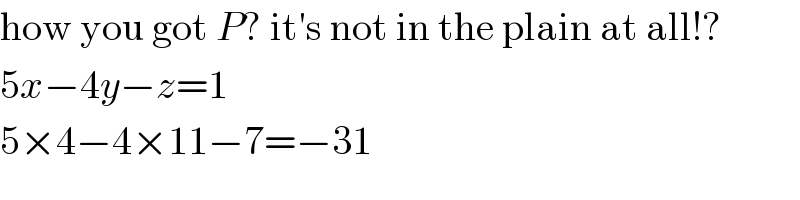
how you got P? it′s not in the plain at all!? 5x−4y−z=1 5×4−4×11−7=−31

$$\mathrm{how}\:\mathrm{you}\:\mathrm{got}\:{P}?\:\mathrm{it}'\mathrm{s}\:\mathrm{not}\:\mathrm{in}\:\mathrm{the}\:\mathrm{plain}\:\mathrm{at}\:\mathrm{all}!? \\ $$$$\mathrm{5}{x}−\mathrm{4}{y}−{z}=\mathrm{1} \\ $$$$\mathrm{5}×\mathrm{4}−\mathrm{4}×\mathrm{11}−\mathrm{7}=−\mathrm{31} \\ $$

Answered by tanmay.chaudhury50@gmail.com last updated on 17/Oct/18

The eqn of st line QR is ((x−2)/(1−2))=((y−3)/(−1−3))=((z−5)/(4−5)) ((x−2)/1)=((y−3)/4)=((z−5)/1)=r (r+2,4r+3,r+5) is the point P...interdection of st line and plane... 5(r+2)−4(4r+3)−1(r+5)=1 5r+10−16r−12−r−5−1=0 −12r−8=0 r=((−2)/3) point P(((−2)/3)+2 ,((−8)/3)+3 ,((−2)/3)+5) point P((4/3),(1/3),((13)/3)) let point S(α,β,γ) poinS lies on QR so ((α−2)/1)=((β−3)/4)=((γ−5)/1)=k (α,β,γ)=(k+2,4k+3,k+5) direction ratio of TS that means betwedn (k+2,4k+3,k+5)and (2,1,4) is (k,4k+2,k+1) TS⊥QR atS so 1(k)+4(4k+2)+1(k+1)=0 18k+8+1=0 k=((−1)/2) so point S is{((−1)/2)+2,4(((−1)/2))+3,((−1)/2)+5} ={(3/2),1,(9/2)}←it is point S {(4/3),(1/3),((13)/3)}←it is point P PS=(√(((4/3)−(3/2))^2 +((1/3)−1)^2 +(((13)/3)−(9/2))^2 )) =(√((((−1)/6))^2 +(((−2)/3))^2 +(((−1)/6))^2 )) =(√((1/(36))+(4/9)+(1/(36)))) =(√((1+16+1)/(36))) =(√((18)/(36))) =(1/((√2) ))ans

$${The}\:{eqn}\:{of}\:{st}\:{line}\:{QR}\:{is} \\ $$$$\frac{{x}−\mathrm{2}}{\mathrm{1}−\mathrm{2}}=\frac{{y}−\mathrm{3}}{−\mathrm{1}−\mathrm{3}}=\frac{{z}−\mathrm{5}}{\mathrm{4}−\mathrm{5}} \\ $$$$\frac{{x}−\mathrm{2}}{\mathrm{1}}=\frac{{y}−\mathrm{3}}{\mathrm{4}}=\frac{{z}−\mathrm{5}}{\mathrm{1}}={r} \\ $$$$\left({r}+\mathrm{2},\mathrm{4}{r}+\mathrm{3},{r}+\mathrm{5}\right)\:{is}\:{the}\:{point}\:{P}...{interdection} \\ $$$${of}\:{st}\:{line}\:{and}\:{plane}... \\ $$$$\mathrm{5}\left({r}+\mathrm{2}\right)−\mathrm{4}\left(\mathrm{4}{r}+\mathrm{3}\right)−\mathrm{1}\left({r}+\mathrm{5}\right)=\mathrm{1} \\ $$$$\mathrm{5}{r}+\mathrm{10}−\mathrm{16}{r}−\mathrm{12}−{r}−\mathrm{5}−\mathrm{1}=\mathrm{0} \\ $$$$−\mathrm{12}{r}−\mathrm{8}=\mathrm{0}\:\:\:{r}=\frac{−\mathrm{2}}{\mathrm{3}} \\ $$$$ \\ $$$${point}\:{P}\left(\frac{−\mathrm{2}}{\mathrm{3}}+\mathrm{2}\:\:,\frac{−\mathrm{8}}{\mathrm{3}}+\mathrm{3}\:\:,\frac{−\mathrm{2}}{\mathrm{3}}+\mathrm{5}\right) \\ $$$$\:\:\:{point}\:{P}\left(\frac{\mathrm{4}}{\mathrm{3}},\frac{\mathrm{1}}{\mathrm{3}},\frac{\mathrm{13}}{\mathrm{3}}\right) \\ $$$${let}\:{point}\:{S}\left(\alpha,\beta,\gamma\right) \\ $$$${poinS}\:{lies}\:{on}\:{QR}\:{so}\:\:\frac{\alpha−\mathrm{2}}{\mathrm{1}}=\frac{\beta−\mathrm{3}}{\mathrm{4}}=\frac{\gamma−\mathrm{5}}{\mathrm{1}}={k} \\ $$$$\left(\alpha,\beta,\gamma\right)=\left({k}+\mathrm{2},\mathrm{4}{k}+\mathrm{3},{k}+\mathrm{5}\right) \\ $$$${direction}\:{ratio}\:{of}\:{TS}\:{that}\:{means}\:{betwedn} \\ $$$$\left({k}+\mathrm{2},\mathrm{4}{k}+\mathrm{3},{k}+\mathrm{5}\right){and}\:\left(\mathrm{2},\mathrm{1},\mathrm{4}\right)\:{is} \\ $$$$\left({k},\mathrm{4}{k}+\mathrm{2},{k}+\mathrm{1}\right) \\ $$$${TS}\bot{QR}\:{atS}\:{so} \\ $$$$\mathrm{1}\left({k}\right)+\mathrm{4}\left(\mathrm{4}{k}+\mathrm{2}\right)+\mathrm{1}\left({k}+\mathrm{1}\right)=\mathrm{0} \\ $$$$\mathrm{18}{k}+\mathrm{8}+\mathrm{1}=\mathrm{0} \\ $$$${k}=\frac{−\mathrm{1}}{\mathrm{2}}\: \\ $$$${so}\:{point}\:{S}\:{is}\left\{\frac{−\mathrm{1}}{\mathrm{2}}+\mathrm{2},\mathrm{4}\left(\frac{−\mathrm{1}}{\mathrm{2}}\right)+\mathrm{3},\frac{−\mathrm{1}}{\mathrm{2}}+\mathrm{5}\right\} \\ $$$$=\left\{\frac{\mathrm{3}}{\mathrm{2}},\mathrm{1},\frac{\mathrm{9}}{\mathrm{2}}\right\}\leftarrow{it}\:{is}\:{point}\:{S} \\ $$$$\left\{\frac{\mathrm{4}}{\mathrm{3}},\frac{\mathrm{1}}{\mathrm{3}},\frac{\mathrm{13}}{\mathrm{3}}\right\}\leftarrow{it}\:{is}\:{point}\:{P} \\ $$$${PS}=\sqrt{\left(\frac{\mathrm{4}}{\mathrm{3}}−\frac{\mathrm{3}}{\mathrm{2}}\right)^{\mathrm{2}} +\left(\frac{\mathrm{1}}{\mathrm{3}}−\mathrm{1}\right)^{\mathrm{2}} +\left(\frac{\mathrm{13}}{\mathrm{3}}−\frac{\mathrm{9}}{\mathrm{2}}\right)^{\mathrm{2}} }\: \\ $$$$=\sqrt{\left(\frac{−\mathrm{1}}{\mathrm{6}}\right)^{\mathrm{2}} +\left(\frac{−\mathrm{2}}{\mathrm{3}}\right)^{\mathrm{2}} +\left(\frac{−\mathrm{1}}{\mathrm{6}}\right)^{\mathrm{2}} }\: \\ $$$$=\sqrt{\frac{\mathrm{1}}{\mathrm{36}}+\frac{\mathrm{4}}{\mathrm{9}}+\frac{\mathrm{1}}{\mathrm{36}}}\: \\ $$$$=\sqrt{\frac{\mathrm{1}+\mathrm{16}+\mathrm{1}}{\mathrm{36}}}\:=\sqrt{\frac{\mathrm{18}}{\mathrm{36}}}\:=\frac{\mathrm{1}}{\sqrt{\mathrm{2}}\:}{ans} \\ $$$$ \\ $$

Commented by rahul 19 last updated on 17/Oct/18

thanks sir!��☺️

Answered by MJS last updated on 17/Oct/18

line QR=l_1 : X=Q+t(R−Q) l_1 : ((x),(y),(z) ) = ((2),(3),(5) ) −t ((1),(4),(1) ) plane p_1 : 5x−4y−z=1 P=l_1 ∩p_1 5(2−t)−4(3−4t)−(5−t)=1 t=(2/3) ⇒ P= (((4/3)),((1/3)),(((13)/3)) ) plane⊥l_1 =p_2 : x+4y+z=c [the direction of l_1 is the perpendicular of p_2 ] T∈p_2 ⇒ c=10 p_2 : x+4y+z=10 S=l_1 ∩p_2 (2−t)+4(3−4t)+(5−t)=10 t=(1/2) ⇒ S= (((3/2)),(1),((9/2)) ) ∣PS∣=(√(((3/2)−(4/3))^2 +(1−(1/3))^2 +((9/2)−((13)/3))^2 ))=((√2)/2)

$$\mathrm{line}\:{QR}={l}_{\mathrm{1}} :\:{X}={Q}+{t}\left({R}−{Q}\right) \\ $$$${l}_{\mathrm{1}} :\:\begin{pmatrix}{{x}}\\{{y}}\\{{z}}\end{pmatrix}\:=\begin{pmatrix}{\mathrm{2}}\\{\mathrm{3}}\\{\mathrm{5}}\end{pmatrix}\:−{t}\begin{pmatrix}{\mathrm{1}}\\{\mathrm{4}}\\{\mathrm{1}}\end{pmatrix} \\ $$$$\mathrm{plane}\:{p}_{\mathrm{1}} :\:\mathrm{5}{x}−\mathrm{4}{y}−{z}=\mathrm{1} \\ $$$${P}={l}_{\mathrm{1}} \cap{p}_{\mathrm{1}} \\ $$$$\mathrm{5}\left(\mathrm{2}−{t}\right)−\mathrm{4}\left(\mathrm{3}−\mathrm{4}{t}\right)−\left(\mathrm{5}−{t}\right)=\mathrm{1} \\ $$$${t}=\frac{\mathrm{2}}{\mathrm{3}}\:\Rightarrow\:{P}=\begin{pmatrix}{\frac{\mathrm{4}}{\mathrm{3}}}\\{\frac{\mathrm{1}}{\mathrm{3}}}\\{\frac{\mathrm{13}}{\mathrm{3}}}\end{pmatrix} \\ $$$$\mathrm{plane}\bot{l}_{\mathrm{1}} ={p}_{\mathrm{2}} :\:{x}+\mathrm{4}{y}+{z}={c} \\ $$$$\:\:\:\:\:\left[\mathrm{the}\:\mathrm{direction}\:\mathrm{of}\:{l}_{\mathrm{1}} \:\mathrm{is}\:\mathrm{the}\:\mathrm{perpendicular}\:\mathrm{of}\:{p}_{\mathrm{2}} \right] \\ $$$${T}\in{p}_{\mathrm{2}} \:\Rightarrow\:{c}=\mathrm{10} \\ $$$${p}_{\mathrm{2}} :\:{x}+\mathrm{4}{y}+{z}=\mathrm{10} \\ $$$${S}={l}_{\mathrm{1}} \cap{p}_{\mathrm{2}} \\ $$$$\left(\mathrm{2}−{t}\right)+\mathrm{4}\left(\mathrm{3}−\mathrm{4}{t}\right)+\left(\mathrm{5}−{t}\right)=\mathrm{10} \\ $$$${t}=\frac{\mathrm{1}}{\mathrm{2}}\:\Rightarrow\:{S}=\begin{pmatrix}{\frac{\mathrm{3}}{\mathrm{2}}}\\{\mathrm{1}}\\{\frac{\mathrm{9}}{\mathrm{2}}}\end{pmatrix} \\ $$$$\mid{PS}\mid=\sqrt{\left(\frac{\mathrm{3}}{\mathrm{2}}−\frac{\mathrm{4}}{\mathrm{3}}\right)^{\mathrm{2}} +\left(\mathrm{1}−\frac{\mathrm{1}}{\mathrm{3}}\right)^{\mathrm{2}} +\left(\frac{\mathrm{9}}{\mathrm{2}}−\frac{\mathrm{13}}{\mathrm{3}}\right)^{\mathrm{2}} }=\frac{\sqrt{\mathrm{2}}}{\mathrm{2}} \\ $$

Commented by rahul 19 last updated on 17/Oct/18

thanks sir!��☺️

Commented by MJS last updated on 17/Oct/18

((√2)/2)=(1/(√2))

$$\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}=\frac{\mathrm{1}}{\sqrt{\mathrm{2}}} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com