Question and Answers Forum

All Questions      Topic List

None Questions

Previous in All Question      Next in All Question      

Previous in None      Next in None      

Question Number 31571 by mondodotto@gmail.com last updated on 10/Mar/18

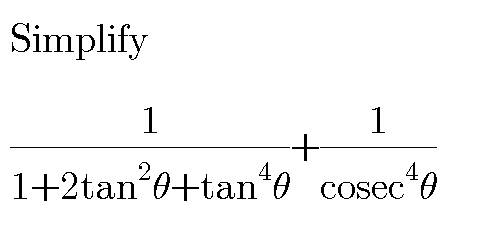
Answered by Joel578 last updated on 10/Mar/18

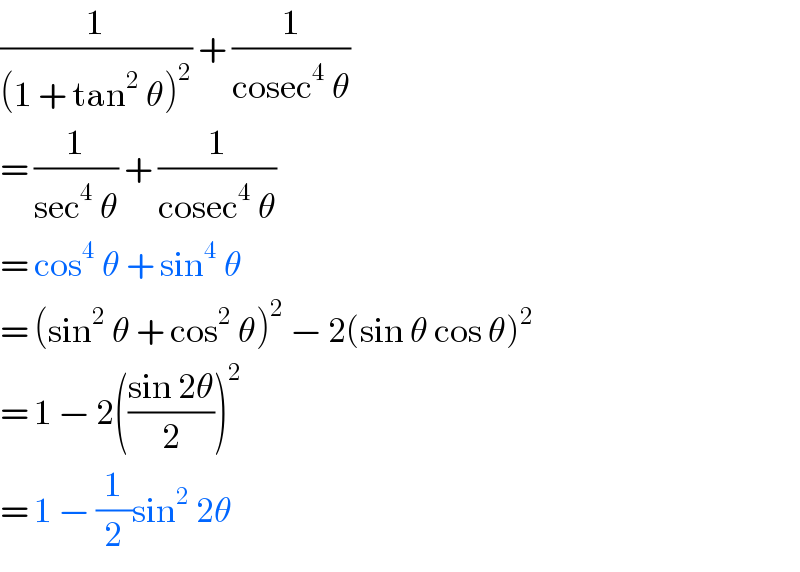
(1/((1 + tan^2 θ)^2 )) + (1/(cosec^4 θ)) = (1/(sec^4 θ)) + (1/(cosec^4 θ)) = cos^4 θ + sin^4 θ = (sin^2 θ + cos^2 θ)^2 − 2(sin θ cos θ)^2 = 1 − 2(((sin 2θ)/2))^2 = 1 − (1/2)sin^2 2θ

$$\frac{\mathrm{1}}{\left(\mathrm{1}\:+\:\mathrm{tan}^{\mathrm{2}} \:\theta\right)^{\mathrm{2}} }\:+\:\frac{\mathrm{1}}{\mathrm{cosec}^{\mathrm{4}} \:\theta} \\ $$$$=\:\frac{\mathrm{1}}{\mathrm{sec}^{\mathrm{4}} \:\theta}\:+\:\frac{\mathrm{1}}{\mathrm{cosec}^{\mathrm{4}} \:\theta} \\ $$$$=\:\mathrm{cos}^{\mathrm{4}} \:\theta\:+\:\mathrm{sin}^{\mathrm{4}} \:\theta \\ $$$$=\:\left(\mathrm{sin}^{\mathrm{2}} \:\theta\:+\:\mathrm{cos}^{\mathrm{2}} \:\theta\right)^{\mathrm{2}} \:−\:\mathrm{2}\left(\mathrm{sin}\:\theta\:\mathrm{cos}\:\theta\right)^{\mathrm{2}} \\ $$$$=\:\mathrm{1}\:−\:\mathrm{2}\left(\frac{\mathrm{sin}\:\mathrm{2}\theta}{\mathrm{2}}\right)^{\mathrm{2}} \\ $$$$=\:\mathrm{1}\:−\:\frac{\mathrm{1}}{\mathrm{2}}\mathrm{sin}^{\mathrm{2}} \:\mathrm{2}\theta \\ $$

Answered by rahul 19 last updated on 10/Mar/18

(1/(1+2tan^2 θ+tan^4 θ))= (1/((tan^2 θ+1)^2 )) = (1/(sec^4 θ))= cos^4 θ. and (1/(cosec^4 θ))=sin^4 θ. cos^4 θ+sin^4 θ.

$$\frac{\mathrm{1}}{\mathrm{1}+\mathrm{2tan}\:^{\mathrm{2}} \theta+\mathrm{tan}\:^{\mathrm{4}} \theta}=\:\frac{\mathrm{1}}{\left(\mathrm{tan}\:^{\mathrm{2}} \theta+\mathrm{1}\right)^{\mathrm{2}} } \\ $$$$=\:\frac{\mathrm{1}}{\mathrm{sec}^{\mathrm{4}} \theta}=\:\mathrm{cos}\:^{\mathrm{4}} \theta. \\ $$$${and}\:\frac{\mathrm{1}}{\mathrm{cosec}\:^{\mathrm{4}} \theta}={sin}^{\mathrm{4}} \theta. \\ $$$${cos}^{\mathrm{4}} \theta+{sin}^{\mathrm{4}} \theta. \\ $$$$ \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com