
Question Number 24753 by math solver last updated on 25/Nov/17
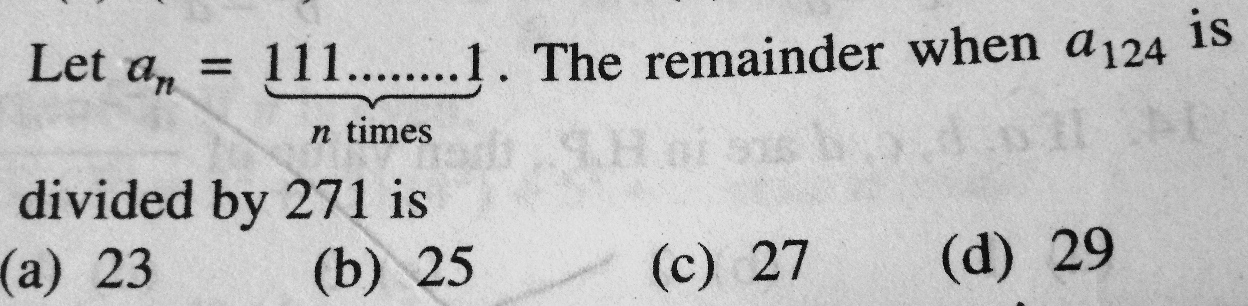
Answered by iv@0uja last updated on 27/Nov/17
![[11111=41×271] a_(124) =1111+10^4 ×11111+10^9 ×11111+... ...+10^(114) ×11111+10^(119) ×11111 ≡1111 (mod 271) =4×271+27 ≡27 (mod 271) ⇒ (c)](Q24851.png)
$$\left[\mathrm{11111}=\mathrm{41}×\mathrm{271}\right] \\ $$$${a}_{\mathrm{124}} =\mathrm{1111}+\mathrm{10}^{\mathrm{4}} ×\mathrm{11111}+\mathrm{10}^{\mathrm{9}} ×\mathrm{11111}+... \\ $$$$\:\:\:\:\:\:\:\:\:\:\:...+\mathrm{10}^{\mathrm{114}} ×\mathrm{11111}+\mathrm{10}^{\mathrm{119}} ×\mathrm{11111} \\ $$$$\:\:\:\:\:\:\:\:\equiv\mathrm{1111}\:\left({mod}\:\mathrm{271}\right) \\ $$$$\:\:\:\:\:\:\:\:=\mathrm{4}×\mathrm{271}+\mathrm{27} \\ $$$$\:\:\:\:\:\:\:\:\equiv\mathrm{27}\:\left({mod}\:\mathrm{271}\right)\:\Rightarrow\:\left({c}\right) \\ $$
Commented by Rasheed.Sindhi last updated on 06/Dec/17

$${g}\underset{\smile} {\overset{\frown} {\mathcal{O}}}\overset{\frown} {\mathcal{O}}{d}\:{strategy}! \\ $$
