
Question Number 22407 by mondodotto@gmail.com last updated on 17/Oct/17

Answered by $@ty@m last updated on 18/Oct/17
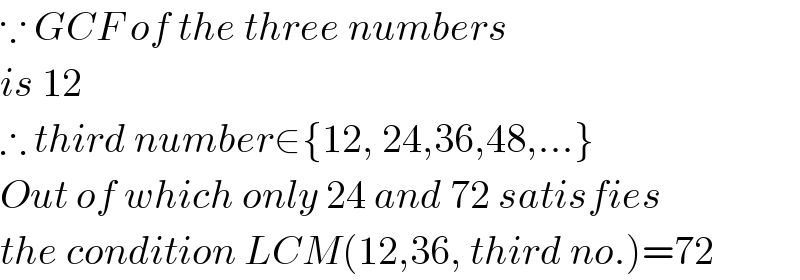
$$\because\:{GCF}\:{of}\:{the}\:{three}\:{numbers}\: \\ $$$${is}\:\mathrm{12} \\ $$$$\therefore\:{third}\:{number}\in\left\{\mathrm{12},\:\mathrm{24},\mathrm{36},\mathrm{48},...\right\} \\ $$$${Out}\:{of}\:{which}\:{only}\:\mathrm{24}\:{and}\:\mathrm{72}\:{satisfies} \\ $$$${the}\:{condition}\:{LCM}\left(\mathrm{12},\mathrm{36},\:{third}\:{no}.\right)=\mathrm{72} \\ $$
Commented by NECx last updated on 17/Oct/17

$${workings}\:{please} \\ $$$$ \\ $$
Answered by Rasheed.Sindhi last updated on 17/Oct/17

$$\mathrm{72}=\mathrm{2}^{\mathrm{3}} .\mathrm{3}^{\mathrm{2}\:} ,\mathrm{12}=\mathrm{2}^{\mathrm{2}} .\mathrm{3}\:,\mathrm{36}=\mathrm{2}^{\mathrm{2}} .\mathrm{3}^{\mathrm{2}} \\ $$$$\bar {\mathrm{L}et}\:\mathrm{the}\:\mathrm{third}\:\mathrm{number}\:\mathrm{is}\:\mathrm{2}^{\mathrm{m}} .\mathrm{3}^{\mathrm{n}} \\ $$$$\mathrm{lcm}\left(\mathrm{2}^{\mathrm{2}} .\mathrm{3},\:\mathrm{2}^{\mathrm{2}} .\mathrm{3}^{\mathrm{2}} ,\:\mathrm{2}^{\mathrm{m}} .\mathrm{3}^{\mathrm{n}} \right) \\ $$$$\:\:\:=\mathrm{2}^{\mathrm{max}\left(\mathrm{2},\mathrm{2},\mathrm{m}\right)} ×\mathrm{3}^{\mathrm{max}\left(\mathrm{1},\mathrm{2},\mathrm{n}\right)} =\mathrm{2}^{\mathrm{3}} .\mathrm{3}^{\mathrm{2}} \\ $$$$\:\:\mathrm{max}\left(\mathrm{2},\mathrm{2},\mathrm{m}\right)=\mathrm{3}\Rightarrow\mathrm{m}=\mathrm{3}....\left(\mathrm{i}\right) \\ $$$$\:\:\mathrm{max}\left(\mathrm{1},\mathrm{2},\mathrm{n}\right)=\mathrm{2}\Rightarrow\mathrm{n}\leqslant\mathrm{2}......\left(\mathrm{ii}\right) \\ $$$$\mathrm{gcd}\left(\mathrm{2}^{\mathrm{2}} .\mathrm{3},\:\mathrm{2}^{\mathrm{2}} .\mathrm{3}^{\mathrm{2}} ,\:\mathrm{2}^{\mathrm{m}} .\mathrm{3}^{\mathrm{n}} \right) \\ $$$$\:\:=\mathrm{2}^{\mathrm{min}\left(\mathrm{2},\mathrm{2},\mathrm{m}\right)} ×\mathrm{3}^{\mathrm{min}\left(\mathrm{1},\mathrm{2},\mathrm{n}\right)} =\mathrm{2}^{\mathrm{2}} ×\mathrm{3} \\ $$$$\:\:\:\mathrm{min}\left(\mathrm{2},\mathrm{2},\mathrm{m}\right)=\mathrm{2}\Rightarrow\mathrm{m}\geqslant\mathrm{2}...\left(\mathrm{iii}\right) \\ $$$$\:\:\:\:\mathrm{min}\left(\mathrm{1},\mathrm{2},\mathrm{n}\right)=\mathrm{1}\Rightarrow\mathrm{n}\geqslant\mathrm{1}....\left(\mathrm{iv}\right) \\ $$$$\left(\mathrm{i}\right)\:\&\:\left(\mathrm{iii}\right): \\ $$$$\mathrm{m}=\mathrm{3}\:\wedge\:\mathrm{m}\geqslant\mathrm{2}\Rightarrow\mathrm{m}=\mathrm{3} \\ $$$$\left(\mathrm{ii}\right)\:\&\:\left(\mathrm{iv}\right): \\ $$$$\mathrm{n}\leqslant\mathrm{2}\:\wedge\:\mathrm{n}\geqslant\mathrm{1}\Rightarrow\mathrm{n}=\mathrm{1},\mathrm{2} \\ $$$$\mathrm{The}\:\mathrm{third}\:\mathrm{number}: \\ $$$$\mathrm{2}^{\mathrm{m}} .\mathrm{3}^{\mathrm{n}} =\mathrm{2}^{\mathrm{3}} .\mathrm{3}\:\:\mathrm{or}\:\:\mathrm{2}^{\mathrm{3}} .\mathrm{3}^{\mathrm{2}} \\ $$$$\mathrm{2}^{\mathrm{m}} .\mathrm{3}^{\mathrm{n}} =\mathrm{24}\:\:\mathrm{or}\:\:\mathrm{72} \\ $$$$ \\ $$
Commented by $@ty@m last updated on 18/Oct/17

$${Nice}.. \\ $$$${In}\:{a}\:{systematic}\:{way}... \\ $$
