Question and Answers Forum

All Questions      Topic List

Geometry Questions

Previous in All Question      Next in All Question      

Previous in Geometry      Next in Geometry      

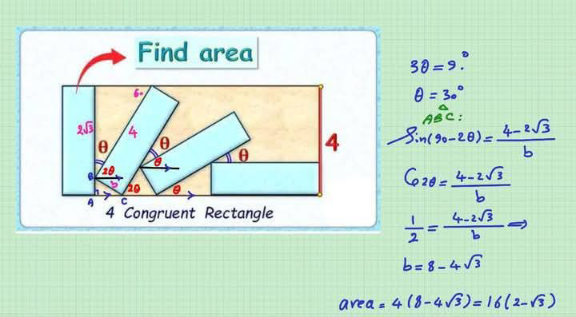
Question Number 218528 by Spillover last updated on 11/Apr/25

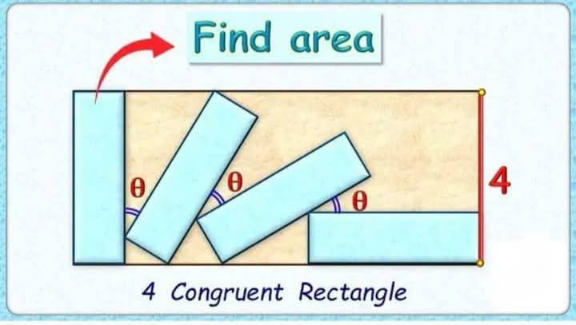
Answered by mr W last updated on 11/Apr/25

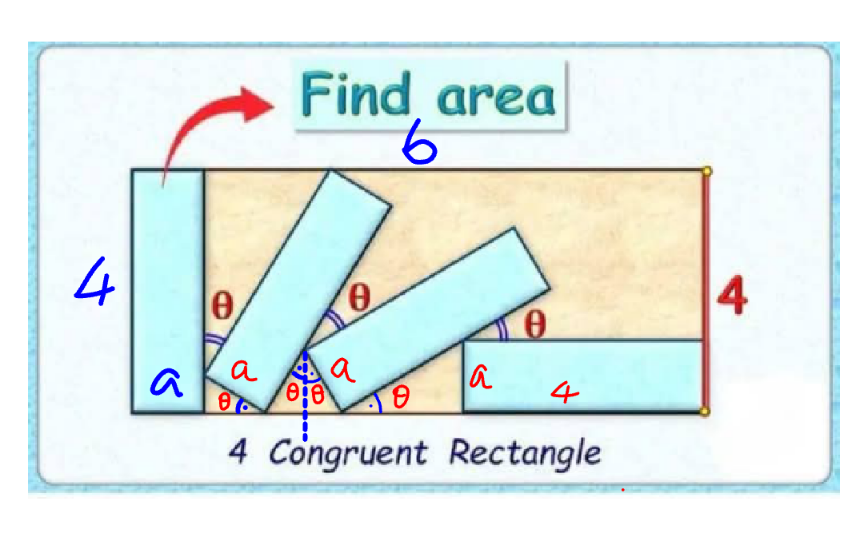
Commented by mr W last updated on 11/Apr/25

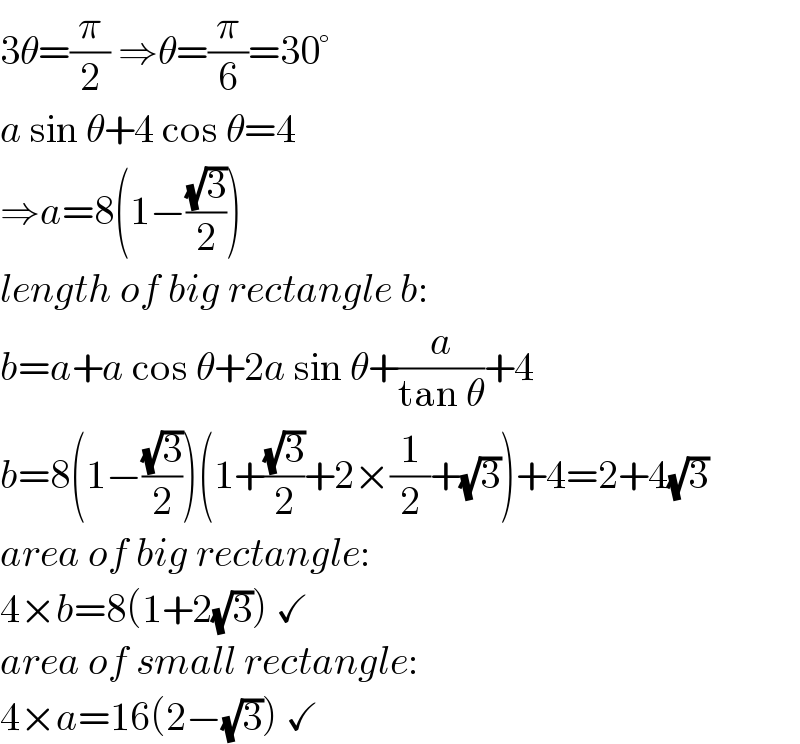
3θ=(π/2) ⇒θ=(π/6)=30° a sin θ+4 cos θ=4 ⇒a=8(1−((√3)/2)) length of big rectangle b: b=a+a cos θ+2a sin θ+(a/(tan θ))+4 b=8(1−((√3)/2))(1+((√3)/2)+2×(1/2)+(√3))+4=2+4(√3) area of big rectangle: 4×b=8(1+2(√3)) ✓ area of small rectangle: 4×a=16(2−(√3)) ✓

$$\mathrm{3}\theta=\frac{\pi}{\mathrm{2}}\:\Rightarrow\theta=\frac{\pi}{\mathrm{6}}=\mathrm{30}° \\ $$$${a}\:\mathrm{sin}\:\theta+\mathrm{4}\:\mathrm{cos}\:\theta=\mathrm{4} \\ $$$$\Rightarrow{a}=\mathrm{8}\left(\mathrm{1}−\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\right) \\ $$$${length}\:{of}\:{big}\:{rectangle}\:{b}: \\ $$$${b}={a}+{a}\:\mathrm{cos}\:\theta+\mathrm{2}{a}\:\mathrm{sin}\:\theta+\frac{{a}}{\mathrm{tan}\:\theta}+\mathrm{4} \\ $$$${b}=\mathrm{8}\left(\mathrm{1}−\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\right)\left(\mathrm{1}+\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}+\mathrm{2}×\frac{\mathrm{1}}{\mathrm{2}}+\sqrt{\mathrm{3}}\right)+\mathrm{4}=\mathrm{2}+\mathrm{4}\sqrt{\mathrm{3}} \\ $$$${area}\:{of}\:{big}\:{rectangle}: \\ $$$$\mathrm{4}×{b}=\mathrm{8}\left(\mathrm{1}+\mathrm{2}\sqrt{\mathrm{3}}\right)\:\checkmark \\ $$$${area}\:{of}\:{small}\:{rectangle}: \\ $$$$\mathrm{4}×{a}=\mathrm{16}\left(\mathrm{2}−\sqrt{\mathrm{3}}\right)\:\checkmark \\ $$

Answered by Spillover last updated on 11/Apr/25

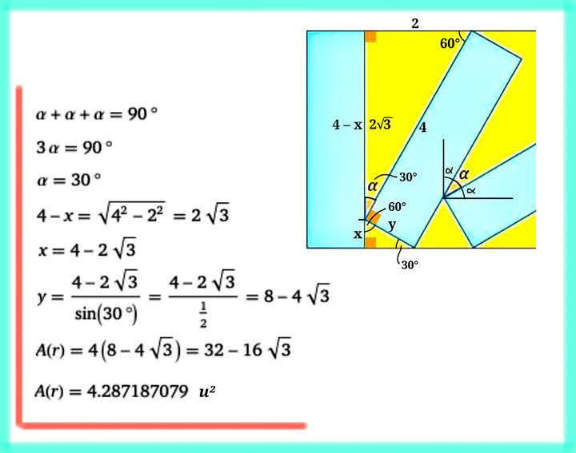
Answered by Spillover last updated on 11/Apr/25

Terms of Service

Privacy Policy

Contact: info@tinkutara.com