
Question Number 218527 by Spillover last updated on 11/Apr/25
Answered by A5T last updated on 12/Apr/25
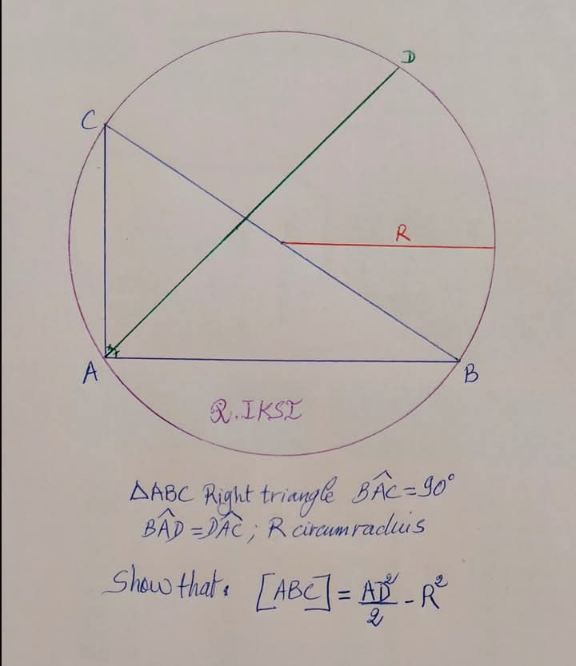
![CD=BD ∧ ∠CDB=90° ⇒ BC=CD(√2)=2R ⇒CD=R(√2) Ptolemy′s theorem: CD×AB+AC×BD=AD×BC ⇒(AB+AC)=AD(√2)...(i) AB^2 +AC^2 =BC^2 ⇒(AB+AC)^2 −2×AB×BC=4R^2 (i)⇒2AD^2 −4[ABC]=4R^2 ⇒[ABC]=((AD^2 )/2)−R^2](Q218597.png)
$$\mathrm{CD}=\mathrm{BD}\:\wedge\:\angle\mathrm{CDB}=\mathrm{90}°\:\Rightarrow\:\mathrm{BC}=\mathrm{CD}\sqrt{\mathrm{2}}=\mathrm{2R} \\ $$$$\Rightarrow\mathrm{CD}=\mathrm{R}\sqrt{\mathrm{2}} \\ $$$$\mathrm{Ptolemy}'\mathrm{s}\:\mathrm{theorem}:\:\mathrm{CD}×\mathrm{AB}+\mathrm{AC}×\mathrm{BD}=\mathrm{AD}×\mathrm{BC} \\ $$$$\Rightarrow\left(\mathrm{AB}+\mathrm{AC}\right)=\mathrm{AD}\sqrt{\mathrm{2}}...\left(\mathrm{i}\right) \\ $$$$\mathrm{AB}^{\mathrm{2}} +\mathrm{AC}^{\mathrm{2}} =\mathrm{BC}^{\mathrm{2}} \\ $$$$\Rightarrow\left(\mathrm{AB}+\mathrm{AC}\right)^{\mathrm{2}} −\mathrm{2}×\mathrm{AB}×\mathrm{BC}=\mathrm{4R}^{\mathrm{2}} \\ $$$$\left(\mathrm{i}\right)\Rightarrow\mathrm{2AD}^{\mathrm{2}} −\mathrm{4}\left[\mathrm{ABC}\right]=\mathrm{4R}^{\mathrm{2}} \\ $$$$\Rightarrow\left[\mathrm{ABC}\right]=\frac{\mathrm{AD}^{\mathrm{2}} }{\mathrm{2}}−\mathrm{R}^{\mathrm{2}} \\ $$
Commented by Spillover last updated on 17/Apr/25
$${thank}\:{you}. \\ $$
