Question and Answers Forum

All Questions      Topic List

Coordinate Geometry Questions

Previous in All Question      Next in All Question      

Previous in Coordinate Geometry      Next in Coordinate Geometry      

Question Number 218384 by Spillover last updated on 08/Apr/25

Answered by Nicholas666 last updated on 09/Apr/25

percentage of red area: ((15π(√3))/(32.3)).100%=((5π(√3))/(32))=100% =((5.π.1,732)/(32)).100%=((27,206)/(32)).100%=85,02%

$${percentage}\:{of}\:{red}\:{area}: \\ $$$$\frac{\mathrm{15}\pi\sqrt{\mathrm{3}}}{\mathrm{32}.\mathrm{3}}.\mathrm{100\%}=\frac{\mathrm{5}\pi\sqrt{\mathrm{3}}}{\mathrm{32}}=\mathrm{100\%} \\ $$$$=\frac{\mathrm{5}.\pi.\mathrm{1},\mathrm{732}}{\mathrm{32}}.\mathrm{100\%}=\frac{\mathrm{27},\mathrm{206}}{\mathrm{32}}.\mathrm{100\%}=\mathrm{85},\mathrm{02\%} \\ $$$$ \\ $$

Answered by Spillover last updated on 09/Apr/25

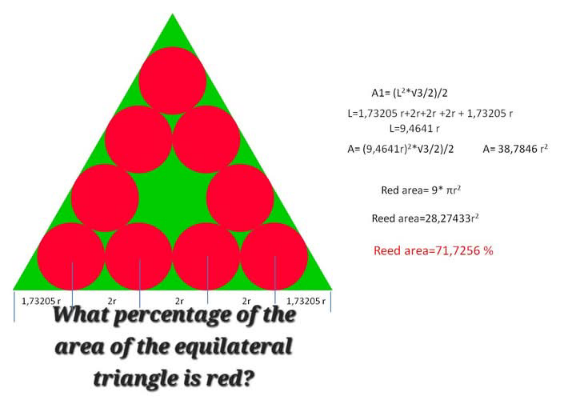
Commented by mr W last updated on 11/Apr/25

but this is not the same figure as in the question!

$${but}\:{this}\:{is}\:{not}\:{the}\:{same}\:{figure}\:{as}\: \\ $$$${in}\:{the}\:{question}! \\ $$

Answered by mr W last updated on 10/Apr/25

Commented by mr W last updated on 10/Apr/25

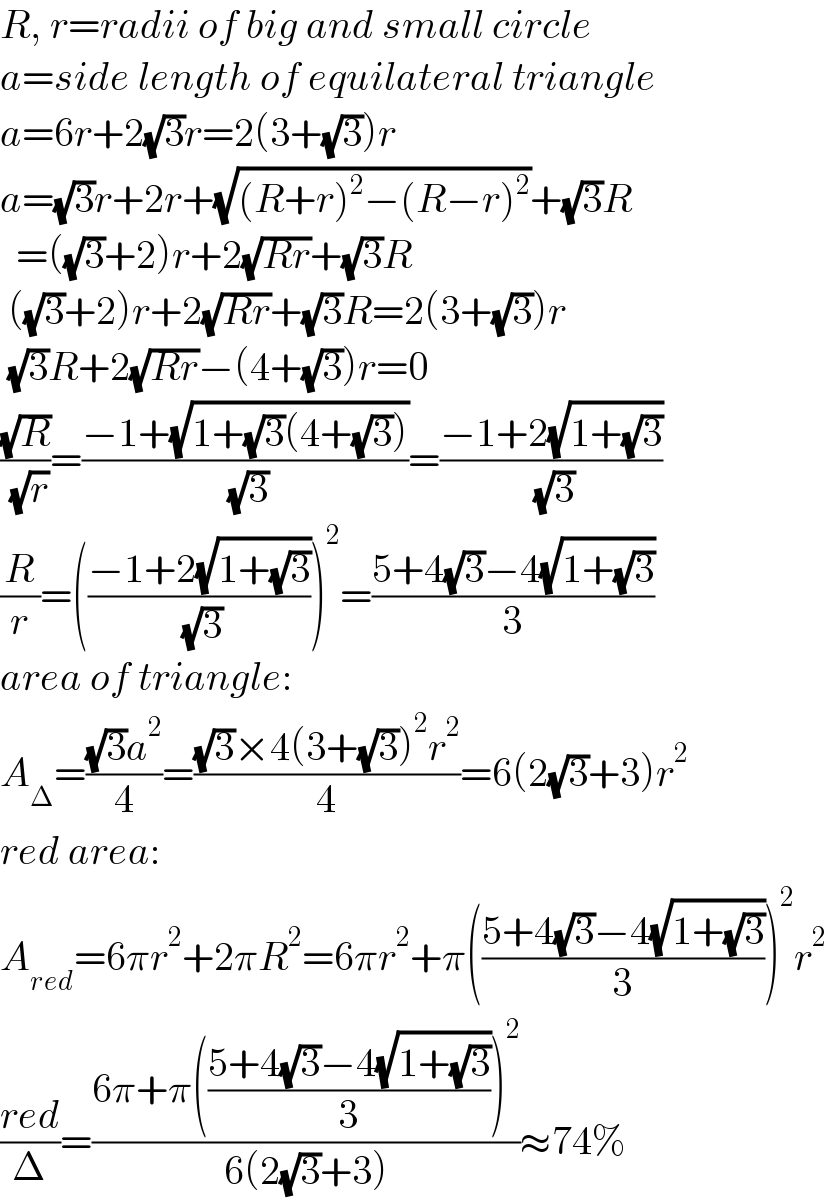
R, r=radii of big and small circle a=side length of equilateral triangle a=6r+2(√3)r=2(3+(√3))r a=(√3)r+2r+(√((R+r)^2 −(R−r)^2 ))+(√3)R =((√3)+2)r+2(√(Rr))+(√3)R ((√3)+2)r+2(√(Rr))+(√3)R=2(3+(√3))r (√3)R+2(√(Rr))−(4+(√3))r=0 ((√R)/( (√r)))=((−1+(√(1+(√3)(4+(√3)))))/( (√3)))=((−1+2(√(1+(√3))))/( (√3))) (R/r)=(((−1+2(√(1+(√3))))/( (√3))))^2 =((5+4(√3)−4(√(1+(√3))))/3) area of triangle: A_Δ =(((√3)a^2 )/4)=(((√3)×4(3+(√3))^2 r^2 )/4)=6(2(√3)+3)r^2 red area: A_(red) =6πr^2 +2πR^2 =6πr^2 +π(((5+4(√3)−4(√(1+(√3))))/3))^2 r^2 ((red)/Δ)=((6π+π(((5+4(√3)−4(√(1+(√3))))/3))^2 )/(6(2(√3)+3)))≈74%

$${R},\:{r}={radii}\:{of}\:{big}\:{and}\:{small}\:{circle} \\ $$$${a}={side}\:{length}\:{of}\:{equilateral}\:{triangle} \\ $$$${a}=\mathrm{6}{r}+\mathrm{2}\sqrt{\mathrm{3}}{r}=\mathrm{2}\left(\mathrm{3}+\sqrt{\mathrm{3}}\right){r} \\ $$$${a}=\sqrt{\mathrm{3}}{r}+\mathrm{2}{r}+\sqrt{\left({R}+{r}\right)^{\mathrm{2}} −\left({R}−{r}\right)^{\mathrm{2}} }+\sqrt{\mathrm{3}}{R} \\ $$$$\:\:=\left(\sqrt{\mathrm{3}}+\mathrm{2}\right){r}+\mathrm{2}\sqrt{{Rr}}+\sqrt{\mathrm{3}}{R} \\ $$$$\:\left(\sqrt{\mathrm{3}}+\mathrm{2}\right){r}+\mathrm{2}\sqrt{{Rr}}+\sqrt{\mathrm{3}}{R}=\mathrm{2}\left(\mathrm{3}+\sqrt{\mathrm{3}}\right){r} \\ $$$$\:\sqrt{\mathrm{3}}{R}+\mathrm{2}\sqrt{{Rr}}−\left(\mathrm{4}+\sqrt{\mathrm{3}}\right){r}=\mathrm{0} \\ $$$$\frac{\sqrt{{R}}}{\:\sqrt{{r}}}=\frac{−\mathrm{1}+\sqrt{\mathrm{1}+\sqrt{\mathrm{3}}\left(\mathrm{4}+\sqrt{\mathrm{3}}\right)}}{\:\sqrt{\mathrm{3}}}=\frac{−\mathrm{1}+\mathrm{2}\sqrt{\mathrm{1}+\sqrt{\mathrm{3}}}}{\:\sqrt{\mathrm{3}}} \\ $$$$\frac{{R}}{{r}}=\left(\frac{−\mathrm{1}+\mathrm{2}\sqrt{\mathrm{1}+\sqrt{\mathrm{3}}}}{\:\sqrt{\mathrm{3}}}\right)^{\mathrm{2}} =\frac{\mathrm{5}+\mathrm{4}\sqrt{\mathrm{3}}−\mathrm{4}\sqrt{\mathrm{1}+\sqrt{\mathrm{3}}}}{\mathrm{3}} \\ $$$${area}\:{of}\:{triangle}: \\ $$$${A}_{\Delta} =\frac{\sqrt{\mathrm{3}}{a}^{\mathrm{2}} }{\mathrm{4}}=\frac{\sqrt{\mathrm{3}}×\mathrm{4}\left(\mathrm{3}+\sqrt{\mathrm{3}}\right)^{\mathrm{2}} {r}^{\mathrm{2}} }{\mathrm{4}}=\mathrm{6}\left(\mathrm{2}\sqrt{\mathrm{3}}+\mathrm{3}\right){r}^{\mathrm{2}} \\ $$$${red}\:{area}: \\ $$$${A}_{{red}} =\mathrm{6}\pi{r}^{\mathrm{2}} +\mathrm{2}\pi{R}^{\mathrm{2}} =\mathrm{6}\pi{r}^{\mathrm{2}} +\pi\left(\frac{\mathrm{5}+\mathrm{4}\sqrt{\mathrm{3}}−\mathrm{4}\sqrt{\mathrm{1}+\sqrt{\mathrm{3}}}}{\mathrm{3}}\right)^{\mathrm{2}} {r}^{\mathrm{2}} \\ $$$$\frac{{red}}{\Delta}=\frac{\mathrm{6}\pi+\pi\left(\frac{\mathrm{5}+\mathrm{4}\sqrt{\mathrm{3}}−\mathrm{4}\sqrt{\mathrm{1}+\sqrt{\mathrm{3}}}}{\mathrm{3}}\right)^{\mathrm{2}} }{\mathrm{6}\left(\mathrm{2}\sqrt{\mathrm{3}}+\mathrm{3}\right)}\approx\mathrm{74\%} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com