Question and Answers Forum

All Questions      Topic List

Algebra Questions

Previous in All Question      Next in All Question      

Previous in Algebra      Next in Algebra      

Question Number 217690 by hardmath last updated on 18/Mar/25

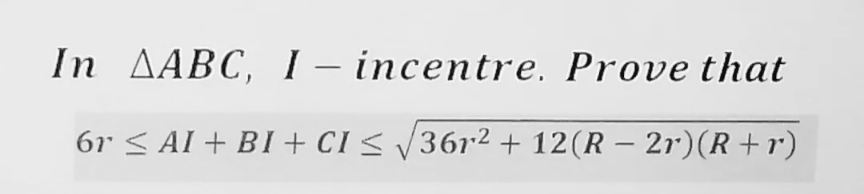
Commented by hardmath last updated on 18/Mar/25

★Hard★

$$\bigstar\mathrm{Hard}\bigstar \\ $$

Commented by mr W last updated on 19/Mar/25

★Hardmath★

$$\bigstar\mathrm{Hard}{math}\bigstar \\ $$

Commented by hardmath last updated on 19/Mar/25

yes dear professor))

$$\left.\mathrm{y}\left.\mathrm{es}\:\mathrm{dear}\:\mathrm{professor}\right)\right) \\ $$

Answered by mr W last updated on 19/Mar/25

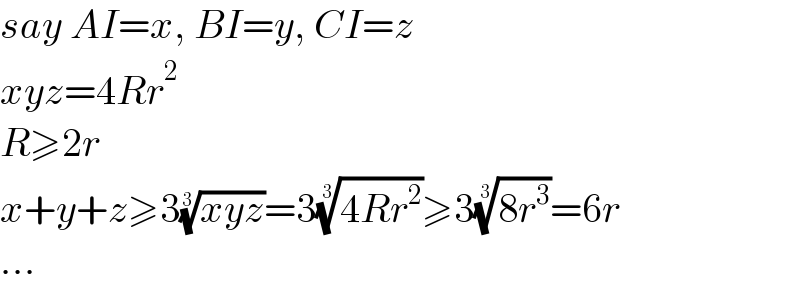
say AI=x, BI=y, CI=z xyz=4Rr^2 R≥2r x+y+z≥3((xyz))^(1/3) =3((4Rr^2 ))^(1/3) ≥3((8r^3 ))^(1/3) =6r ...

$${say}\:{AI}={x},\:{BI}={y},\:{CI}={z} \\ $$$${xyz}=\mathrm{4}{Rr}^{\mathrm{2}} \\ $$$${R}\geqslant\mathrm{2}{r} \\ $$$${x}+{y}+{z}\geqslant\mathrm{3}\sqrt[{\mathrm{3}}]{{xyz}}=\mathrm{3}\sqrt[{\mathrm{3}}]{\mathrm{4}{Rr}^{\mathrm{2}} }\geqslant\mathrm{3}\sqrt[{\mathrm{3}}]{\mathrm{8}{r}^{\mathrm{3}} }=\mathrm{6}{r} \\ $$$$... \\ $$

Commented by hardmath last updated on 19/Mar/25

dear professor, that′s it?)

$$\left.\mathrm{dear}\:\mathrm{professor},\:\mathrm{that}'\mathrm{s}\:\mathrm{it}?\right) \\ $$

Commented by mr W last updated on 19/Mar/25

what′s it what you mean?

$${what}'{s}\:{it}\:{what}\:{you}\:{mean}? \\ $$

Commented by hardmath last updated on 19/Mar/25

That is, the solution (proof) is this value

$$ \\ $$That is, the solution (proof) is this value

Commented by mr W last updated on 19/Mar/25

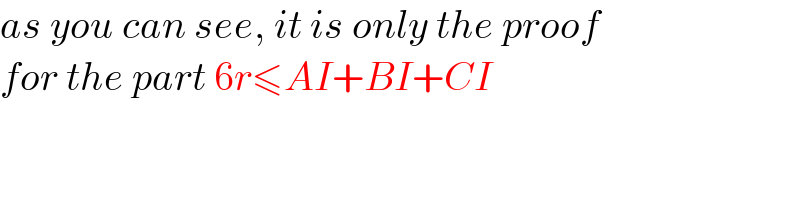
as you can see, it is only the proof for the part 6r≤AI+BI+CI

$${as}\:{you}\:{can}\:{see},\:{it}\:{is}\:{only}\:{the}\:{proof}\: \\ $$$${for}\:{the}\:{part}\:\mathrm{6}{r}\leqslant{AI}+{BI}+{CI} \\ $$

Commented by mr W last updated on 19/Mar/25

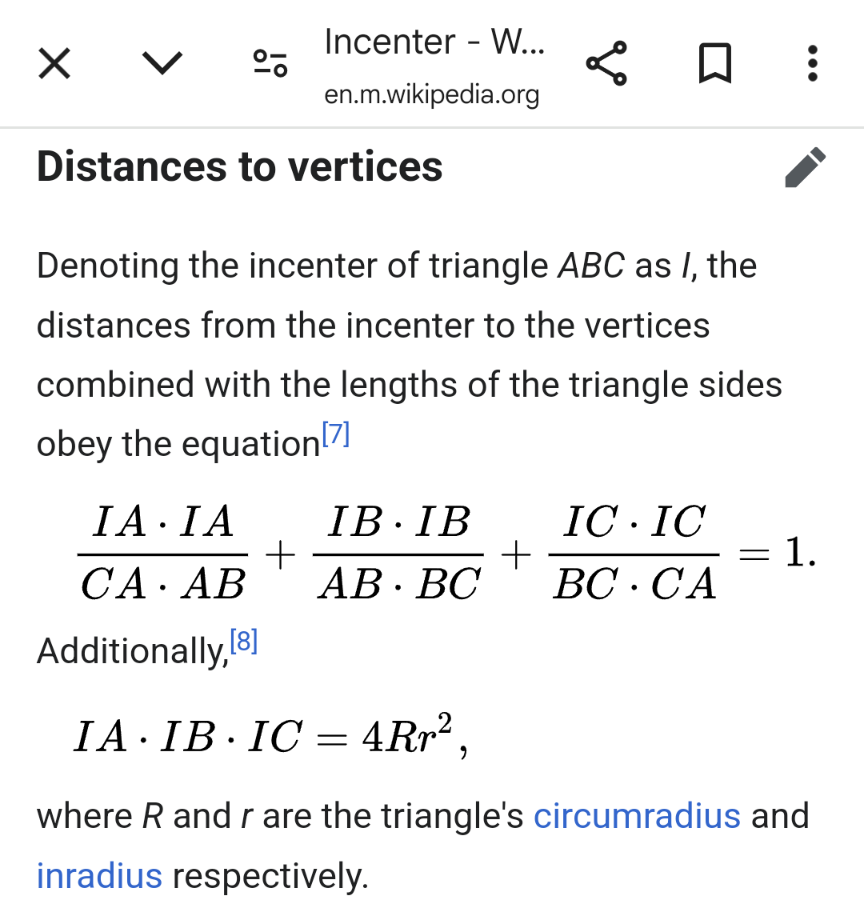
Commented by hardmath last updated on 21/Mar/25

thankyou dearprofessor

$$\mathrm{thankyou}\:\mathrm{dearprofessor} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com