
Question Number 217686 by mnjuly1970 last updated on 18/Mar/25
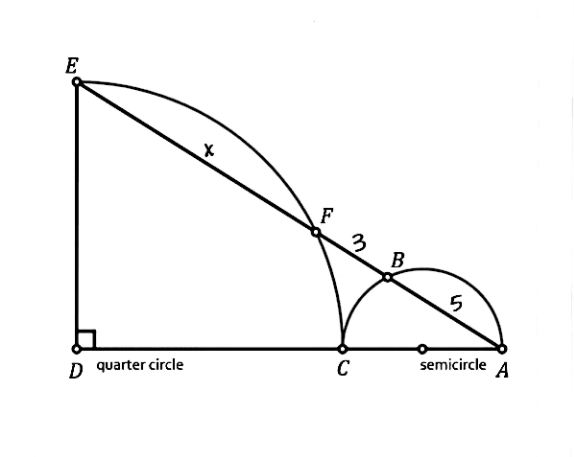
Commented by mnjuly1970 last updated on 18/Mar/25
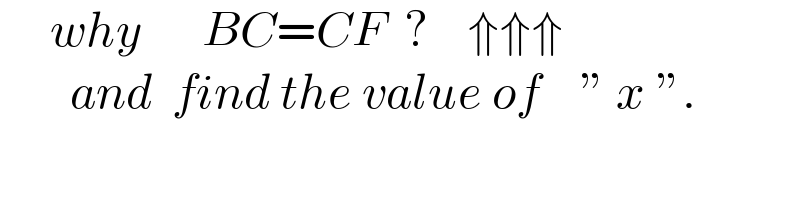
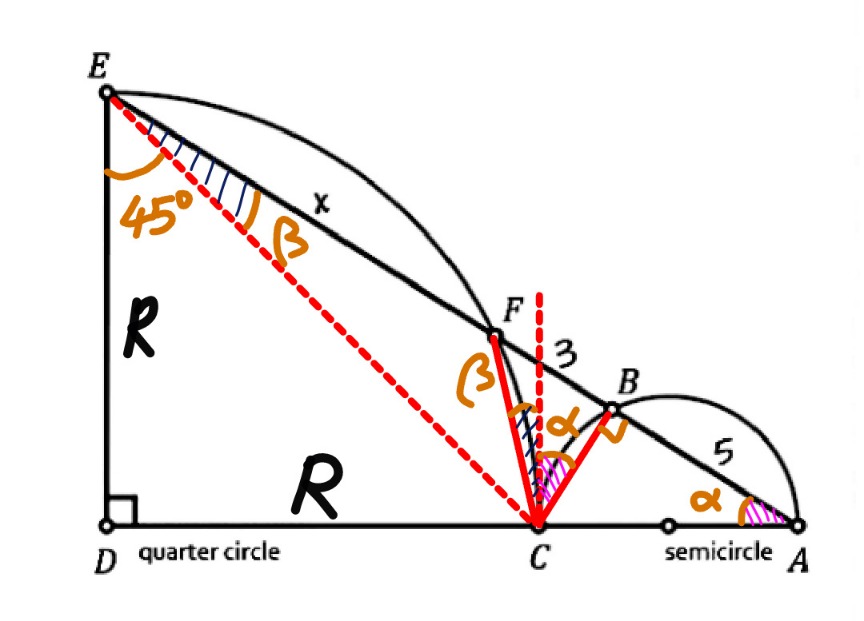
$$\:\:\:\:\:{why}\:\:\:\:\:\:{BC}={CF}\:\:?\:\:\:\:\Uparrow\Uparrow\Uparrow \\ $$$$\:\:\:\:\:\:\:{and}\:\:{find}\:{the}\:{value}\:{of}\:\:\:\:''\:{x}\:''. \\ $$
Commented by mr W last updated on 18/Mar/25
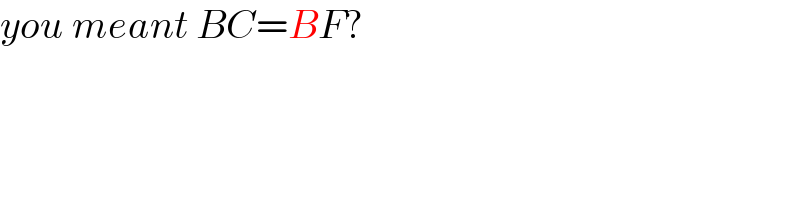
$${you}\:{meant}\:{BC}={BF}? \\ $$
Commented by mnjuly1970 last updated on 18/Mar/25
$${yes}\:{yes}\:{sir}\:.{my}\:{mistake}..\:\underline{\underbrace{\lesseqgtr}} \\ $$
Answered by mr W last updated on 19/Mar/25
Commented by mr W last updated on 19/Mar/25
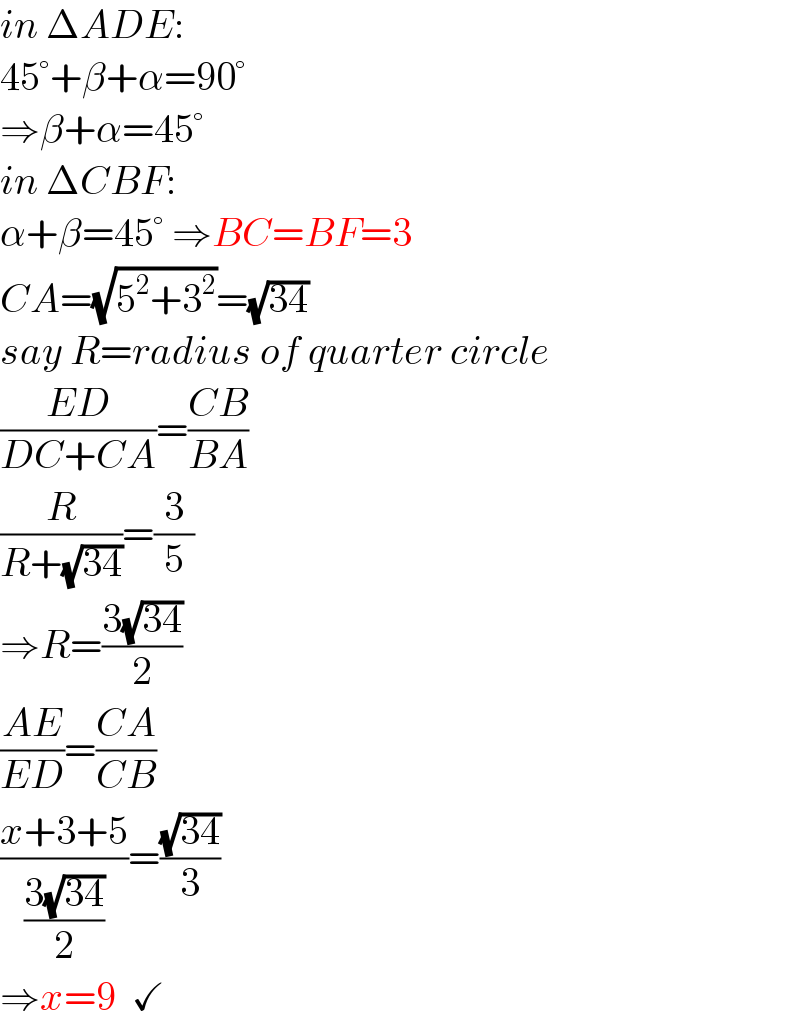
$${in}\:\Delta{ADE}: \\ $$$$\mathrm{45}°+\beta+\alpha=\mathrm{90}° \\ $$$$\Rightarrow\beta+\alpha=\mathrm{45}° \\ $$$${in}\:\Delta{CBF}: \\ $$$$\alpha+\beta=\mathrm{45}°\:\Rightarrow{BC}={BF}=\mathrm{3} \\ $$$${CA}=\sqrt{\mathrm{5}^{\mathrm{2}} +\mathrm{3}^{\mathrm{2}} }=\sqrt{\mathrm{34}} \\ $$$${say}\:{R}={radius}\:{of}\:{quarter}\:{circle} \\ $$$$\frac{{ED}}{{DC}+{CA}}=\frac{{CB}}{{BA}} \\ $$$$\frac{{R}}{{R}+\sqrt{\mathrm{34}}}=\frac{\mathrm{3}}{\mathrm{5}} \\ $$$$\Rightarrow{R}=\frac{\mathrm{3}\sqrt{\mathrm{34}}}{\mathrm{2}} \\ $$$$\frac{{AE}}{{ED}}=\frac{{CA}}{{CB}} \\ $$$$\frac{{x}+\mathrm{3}+\mathrm{5}}{\frac{\mathrm{3}\sqrt{\mathrm{34}}}{\mathrm{2}}}=\frac{\sqrt{\mathrm{34}}}{\mathrm{3}} \\ $$$$\Rightarrow{x}=\mathrm{9}\:\:\checkmark \\ $$
