
Question Number 216587 by Tawa11 last updated on 11/Feb/25
Commented by Tawa11 last updated on 11/Feb/25

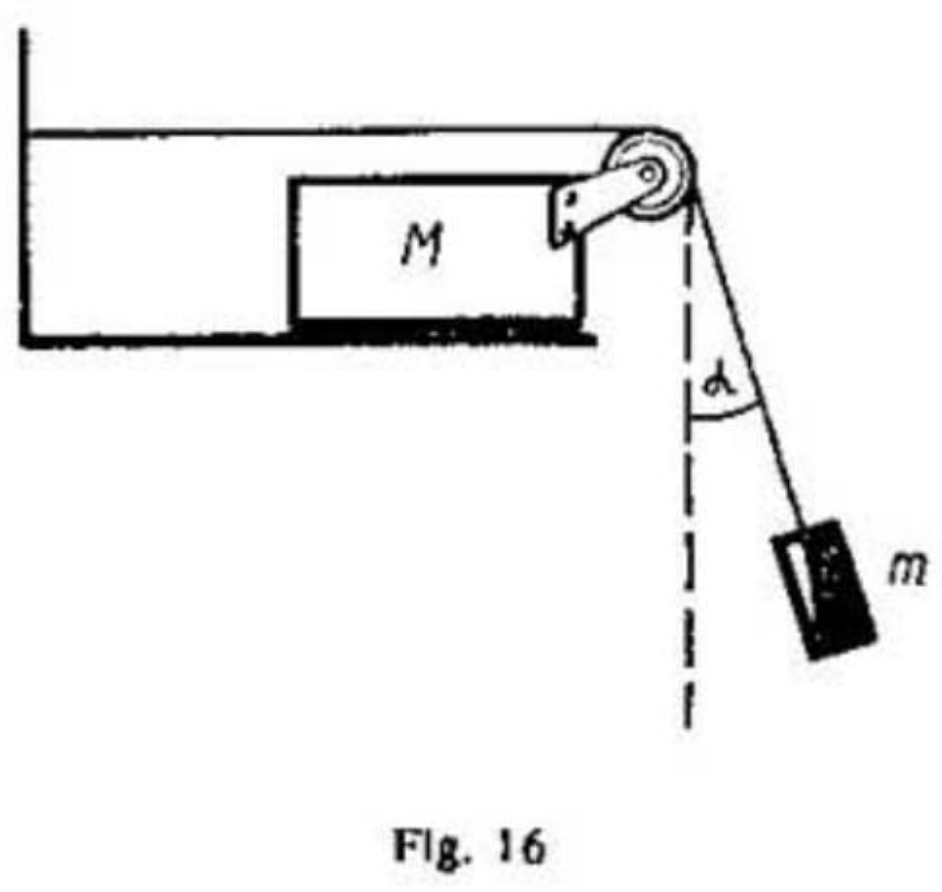
In the mechanical system represented in Figure 16, the bar, whose mass is "M", can slide along the rails without friction. At the initial moment the load suspended in wire deviates from the vertical to the angle "α" and is released. What is the mass "m" of this load, if the angle formed by the thread with the vertical does not vary during the movement of the system?*
Commented by mr W last updated on 12/Feb/25

$$\frac{{m}}{{M}}=\frac{\mathrm{sin}\:\alpha}{\left(\mathrm{1}−\mathrm{sin}\:\alpha\right)^{\mathrm{2}} } \\ $$$${T}=\frac{{Mg}\:\mathrm{tan}\:\alpha}{\mathrm{1}−\mathrm{sin}\:\alpha}={tension}\:{in}\:{wire} \\ $$$${do}\:{you}\:{know}\:{the}\:{correct}\:{answer}? \\ $$
Commented by Tawa11 last updated on 12/Feb/25
$$\mathrm{No}\:\mathrm{sir}. \\ $$$$\mathrm{I}\:\mathrm{don}'\mathrm{t}\:\mathrm{know}\:\mathrm{the}\:\mathrm{correct}\:\mathrm{answer}. \\ $$
Commented by Tawa11 last updated on 12/Feb/25
$$\mathrm{Please}\:\mathrm{sir},\:\mathrm{show}\:\mathrm{me}\:\mathrm{the}\:\mathrm{full}\:\mathrm{working}. \\ $$$$\mathrm{Thanks}\:\mathrm{sir}. \\ $$
Answered by mr W last updated on 12/Feb/25
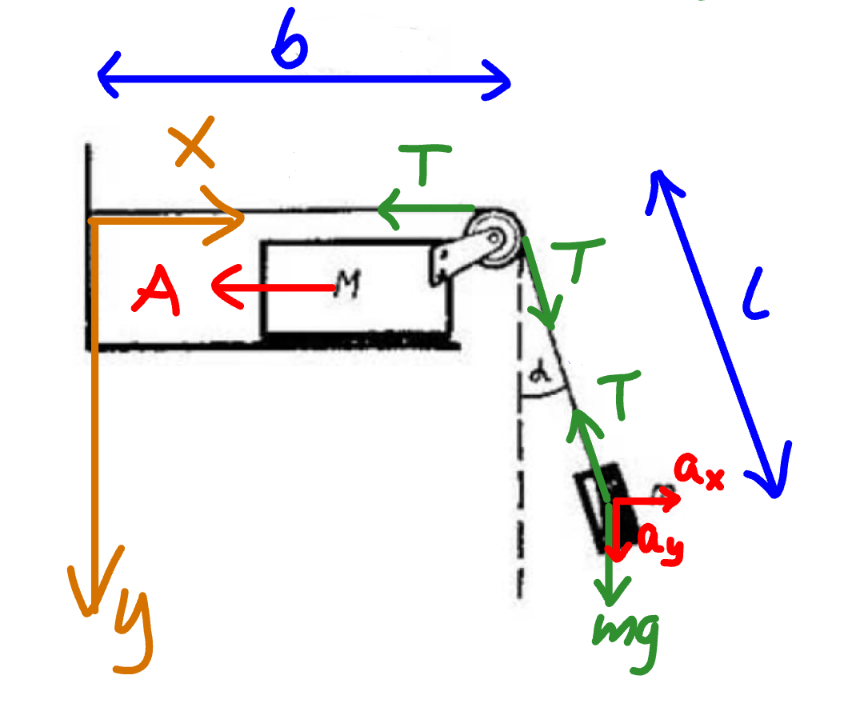
Commented by mr W last updated on 12/Feb/25
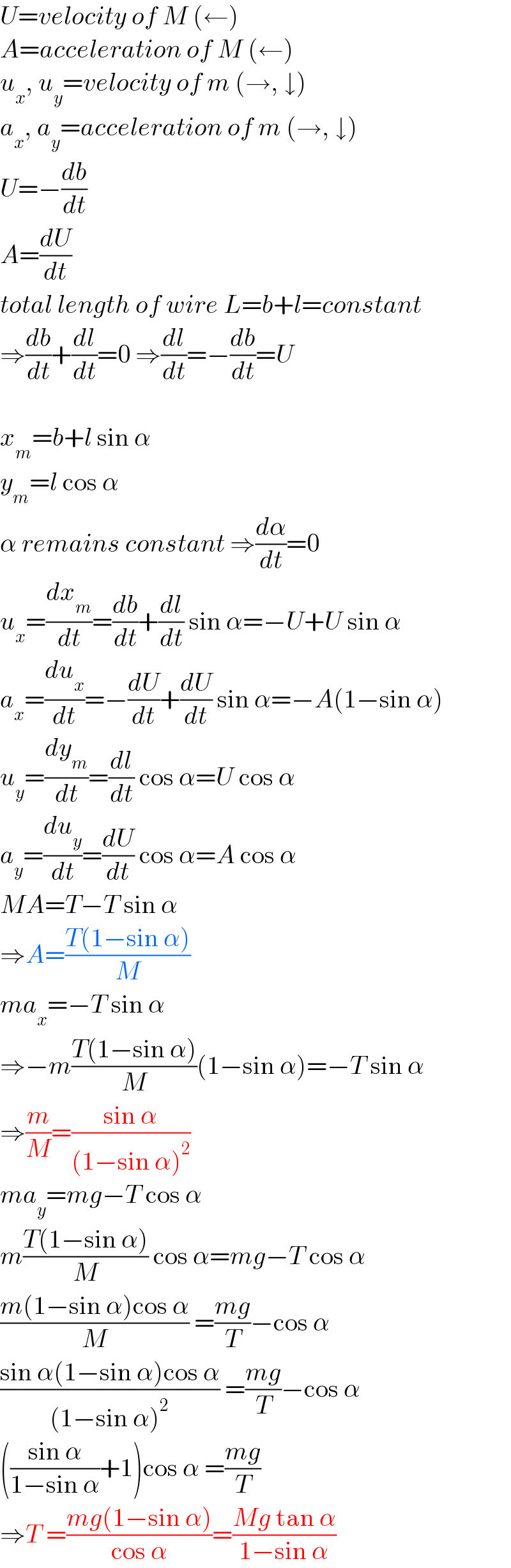
$${U}={velocity}\:{of}\:{M}\:\left(\leftarrow\right) \\ $$$${A}={acceleration}\:{of}\:{M}\:\left(\leftarrow\right) \\ $$$${u}_{{x}} ,\:{u}_{{y}} ={velocity}\:{of}\:{m}\:\left(\rightarrow,\:\downarrow\right) \\ $$$${a}_{{x}} ,\:{a}_{{y}} ={acceleration}\:{of}\:{m}\:\left(\rightarrow,\:\downarrow\right) \\ $$$${U}=−\frac{{db}}{{dt}} \\ $$$${A}=\frac{{dU}}{{dt}} \\ $$$${total}\:{length}\:{of}\:{wire}\:{L}={b}+{l}={constant} \\ $$$$\Rightarrow\frac{{db}}{{dt}}+\frac{{dl}}{{dt}}=\mathrm{0}\:\Rightarrow\frac{{dl}}{{dt}}=−\frac{{db}}{{dt}}={U} \\ $$$$ \\ $$$${x}_{{m}} ={b}+{l}\:\mathrm{sin}\:\alpha \\ $$$${y}_{{m}} ={l}\:\mathrm{cos}\:\alpha \\ $$$$\alpha\:{remains}\:{constant}\:\Rightarrow\frac{{d}\alpha}{{dt}}=\mathrm{0} \\ $$$${u}_{{x}} =\frac{{dx}_{{m}} }{{dt}}=\frac{{db}}{{dt}}+\frac{{dl}}{{dt}}\:\mathrm{sin}\:\alpha=−{U}+{U}\:\mathrm{sin}\:\alpha \\ $$$${a}_{{x}} =\frac{{du}_{{x}} }{{dt}}=−\frac{{dU}}{{dt}}+\frac{{dU}}{{dt}}\:\mathrm{sin}\:\alpha=−{A}\left(\mathrm{1}−\mathrm{sin}\:\alpha\right) \\ $$$${u}_{{y}} =\frac{{dy}_{{m}} }{{dt}}=\frac{{dl}}{{dt}}\:\mathrm{cos}\:\alpha={U}\:\mathrm{cos}\:\alpha \\ $$$${a}_{{y}} =\frac{{du}_{{y}} }{{dt}}=\frac{{dU}}{{dt}}\:\mathrm{cos}\:\alpha={A}\:\mathrm{cos}\:\alpha \\ $$$${MA}={T}−{T}\:\mathrm{sin}\:\alpha \\ $$$$\Rightarrow{A}=\frac{{T}\left(\mathrm{1}−\mathrm{sin}\:\alpha\right)}{{M}} \\ $$$${ma}_{{x}} =−{T}\:\mathrm{sin}\:\alpha \\ $$$$\Rightarrow−{m}\frac{{T}\left(\mathrm{1}−\mathrm{sin}\:\alpha\right)}{{M}}\left(\mathrm{1}−\mathrm{sin}\:\alpha\right)=−{T}\:\mathrm{sin}\:\alpha \\ $$$$\Rightarrow\frac{{m}}{{M}}=\frac{\mathrm{sin}\:\alpha}{\left(\mathrm{1}−\mathrm{sin}\:\alpha\right)^{\mathrm{2}} } \\ $$$${ma}_{{y}} ={mg}−{T}\:\mathrm{cos}\:\alpha \\ $$$${m}\frac{{T}\left(\mathrm{1}−\mathrm{sin}\:\alpha\right)}{{M}}\:\mathrm{cos}\:\alpha={mg}−{T}\:\mathrm{cos}\:\alpha \\ $$$$\frac{{m}\left(\mathrm{1}−\mathrm{sin}\:\alpha\right)\mathrm{cos}\:\alpha}{{M}}\:=\frac{{mg}}{{T}}−\mathrm{cos}\:\alpha \\ $$$$\frac{\mathrm{sin}\:\alpha\left(\mathrm{1}−\mathrm{sin}\:\alpha\right)\mathrm{cos}\:\alpha}{\left(\mathrm{1}−\mathrm{sin}\:\alpha\right)^{\mathrm{2}} }\:=\frac{{mg}}{{T}}−\mathrm{cos}\:\alpha \\ $$$$\left(\frac{\mathrm{sin}\:\alpha}{\mathrm{1}−\mathrm{sin}\:\alpha}+\mathrm{1}\right)\mathrm{cos}\:\alpha\:=\frac{{mg}}{{T}} \\ $$$$\Rightarrow{T}\:=\frac{{mg}\left(\mathrm{1}−\mathrm{sin}\:\alpha\right)}{\mathrm{cos}\:\alpha}=\frac{{Mg}\:\mathrm{tan}\:\alpha}{\mathrm{1}−\mathrm{sin}\:\alpha} \\ $$
Commented by Tawa11 last updated on 12/Feb/25
$$\mathrm{Thanks}\:\mathrm{for}\:\mathrm{your}\:\mathrm{time}\:\mathrm{sir},\:\mathrm{I}\:\mathrm{appreciate}. \\ $$
