
Previous in Matrices and Determinants Next in Matrices and Determinants
Question Number 216526 by Mingma last updated on 10/Feb/25
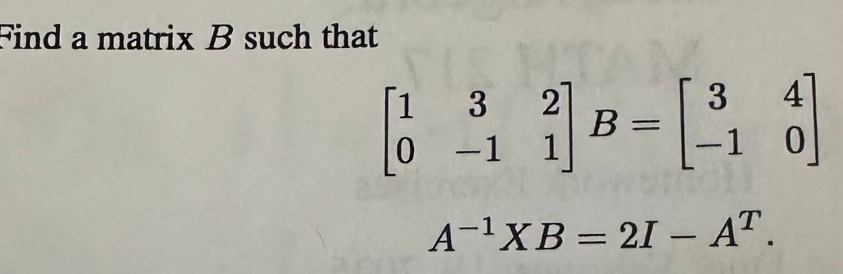
Answered by Wuji last updated on 10/Feb/25
![let B= [((a b)),((c d)),((e f)) ] , C= [((3 4)),((−1 0)) ] : AB= [((1 3 2)),((0 −1 1)) ] [((a b)),((c d)),((e f)) ]= [((a+3c+2e b+3d+2f)),((−c −e −d +f )) ] AB=C ⇒ a+3c+2e=3 ⇒ b+3d+2f=4 ⇒ −c +e=−1 ⇒e=c−1 ⇒ −d +f=0 ⇒ f=d a+3c+2(c−1)=3 ⇒ a+5c=5 ⇒a=5−5c from b+3d+2f=4 b+3d+2d ⇒b+5d=4 ⇒b=4−5d {c=0 , d=0 arbitrary} then, a=5 , b=4 ,c=0,e=−1 , f=d=0 B= [(( 5 4)),(( 0 0)),((−1 0)) ] Checking if AB=C [((1 3 2)),((0 −1 1)) ] [((5 4)),((0 0)),((−1 0)) ]= [((1(5)+3(0)+2(−1) 1(4)+3(0)+2(0))),((0(5)+(−1)(0)+1(−1) 0(4)+(−1)(0)+1(0))) ] [((5 +0−2 4+0+0)),((0 +0−1 0+0+0)) ]= [((3 4)),((−1 0)) ]](Q216528.png)
$$\mathrm{let}\:\mathrm{B}=\begin{bmatrix}{\mathrm{a}\:\:\:\:\:\:\:\mathrm{b}}\\{\mathrm{c}\:\:\:\:\:\:\:\:\mathrm{d}}\\{\mathrm{e}\:\:\:\:\:\:\:\:\mathrm{f}}\end{bmatrix}\:,\:\mathrm{C}=\begin{bmatrix}{\mathrm{3}\:\:\:\:\:\:\:\:\mathrm{4}}\\{−\mathrm{1}\:\:\:\:\mathrm{0}}\end{bmatrix}\:: \\ $$$$\mathrm{AB}=\begin{bmatrix}{\mathrm{1}\:\:\:\mathrm{3}\:\:\:\:\:\mathrm{2}}\\{\mathrm{0}\:\:−\mathrm{1}\:\:\:\mathrm{1}}\end{bmatrix}\begin{bmatrix}{\mathrm{a}\:\:\:\:\mathrm{b}}\\{\mathrm{c}\:\:\:\:\:\mathrm{d}}\\{\mathrm{e}\:\:\:\:\:\mathrm{f}}\end{bmatrix}=\begin{bmatrix}{\mathrm{a}+\mathrm{3c}+\mathrm{2e}\:\:\:\:\mathrm{b}+\mathrm{3d}+\mathrm{2f}}\\{−\mathrm{c}\:\:−\mathrm{e}\:\:\:\:\:\:\:\:\:−\mathrm{d}\:\:+\mathrm{f}\:\:\:\:}\end{bmatrix} \\ $$$$\mathrm{AB}=\mathrm{C}\:\:\Rightarrow\:\mathrm{a}+\mathrm{3c}+\mathrm{2e}=\mathrm{3} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\Rightarrow\:\mathrm{b}+\mathrm{3d}+\mathrm{2f}=\mathrm{4} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\Rightarrow\:−\mathrm{c}\:+\mathrm{e}=−\mathrm{1}\:\:\Rightarrow\mathrm{e}=\mathrm{c}−\mathrm{1} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\Rightarrow\:−\mathrm{d}\:+\mathrm{f}=\mathrm{0}\:\:\Rightarrow\:\mathrm{f}=\mathrm{d} \\ $$$$\mathrm{a}+\mathrm{3c}+\mathrm{2}\left(\mathrm{c}−\mathrm{1}\right)=\mathrm{3}\:\:\Rightarrow\:\mathrm{a}+\mathrm{5c}=\mathrm{5}\:\Rightarrow\mathrm{a}=\mathrm{5}−\mathrm{5c} \\ $$$$\mathrm{from}\:\:\mathrm{b}+\mathrm{3d}+\mathrm{2f}=\mathrm{4} \\ $$$$\mathrm{b}+\mathrm{3d}+\mathrm{2d}\:\Rightarrow\mathrm{b}+\mathrm{5d}=\mathrm{4}\:\Rightarrow\mathrm{b}=\mathrm{4}−\mathrm{5d} \\ $$$$\left\{\mathrm{c}=\mathrm{0}\:,\:\mathrm{d}=\mathrm{0}\:\:\:\:\mathrm{arbitrary}\right\} \\ $$$$\mathrm{then}, \\ $$$$\mathrm{a}=\mathrm{5}\:\:,\:\mathrm{b}=\mathrm{4}\:,\mathrm{c}=\mathrm{0},\mathrm{e}=−\mathrm{1}\:,\:\mathrm{f}=\mathrm{d}=\mathrm{0} \\ $$$$\mathrm{B}=\begin{bmatrix}{\:\:\:\mathrm{5}\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{4}}\\{\:\:\:\mathrm{0}\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{0}}\\{−\mathrm{1}\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{0}}\end{bmatrix} \\ $$$$\mathrm{Checking} \\ $$$$\mathrm{if}\:\mathrm{AB}=\mathrm{C} \\ $$$$\begin{bmatrix}{\mathrm{1}\:\:\:\mathrm{3}\:\:\:\:\mathrm{2}}\\{\mathrm{0}\:−\mathrm{1}\:\:\mathrm{1}}\end{bmatrix}\begin{bmatrix}{\mathrm{5}\:\:\:\:\:\:\:\:\:\:\mathrm{4}}\\{\mathrm{0}\:\:\:\:\:\:\:\:\:\mathrm{0}}\\{−\mathrm{1}\:\:\:\:\:\mathrm{0}}\end{bmatrix}=\begin{bmatrix}{\mathrm{1}\left(\mathrm{5}\right)+\mathrm{3}\left(\mathrm{0}\right)+\mathrm{2}\left(−\mathrm{1}\right)\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{1}\left(\mathrm{4}\right)+\mathrm{3}\left(\mathrm{0}\right)+\mathrm{2}\left(\mathrm{0}\right)}\\{\mathrm{0}\left(\mathrm{5}\right)+\left(−\mathrm{1}\right)\left(\mathrm{0}\right)+\mathrm{1}\left(−\mathrm{1}\right)\:\:\:\:\:\mathrm{0}\left(\mathrm{4}\right)+\left(−\mathrm{1}\right)\left(\mathrm{0}\right)+\mathrm{1}\left(\mathrm{0}\right)}\end{bmatrix} \\ $$$$\begin{bmatrix}{\mathrm{5}\:+\mathrm{0}−\mathrm{2}\:\:\:\:\:\:\:\mathrm{4}+\mathrm{0}+\mathrm{0}}\\{\mathrm{0}\:+\mathrm{0}−\mathrm{1}\:\:\:\:\:\:\:\mathrm{0}+\mathrm{0}+\mathrm{0}}\end{bmatrix}=\begin{bmatrix}{\mathrm{3}\:\:\:\:\:\:\:\mathrm{4}}\\{−\mathrm{1}\:\:\:\mathrm{0}}\end{bmatrix} \\ $$
