Question and Answers Forum

All Questions      Topic List

None Questions

Previous in All Question      Next in All Question      

Previous in None      Next in None      

Question Number 216437 by issac last updated on 08/Feb/25

Answered by issac last updated on 08/Feb/25

Q. Maxwells equations for electric and magntic fields are as follows. Choose one that can be described by the above equation. A) A continuous equation is established between charge density and current density B) A magnetic field is generated while the capacitor is charged. C) The strength of the electric field produced by a stationary point charge is inversely proportional to the square of the distance

$$\mathrm{Q}. \\ $$$$\mathrm{Maxwells}\:\mathrm{equations}\:\mathrm{for}\:\mathrm{electric}\:\mathrm{and} \\ $$$$\mathrm{magntic}\:\mathrm{fields}\:\mathrm{are}\:\mathrm{as}\:\mathrm{follows}. \\ $$$$\: \\ $$$$\mathrm{Choose}\:\mathrm{one}\:\mathrm{that}\:\mathrm{can}\:\mathrm{be}\:\mathrm{described}\:\mathrm{by}\:\mathrm{the} \\ $$$$\mathrm{above}\:\mathrm{equation}. \\ $$$$\: \\ $$$$\left.\boldsymbol{\mathrm{A}}\right) \\ $$$$\mathrm{A}\:\mathrm{continuous}\:\mathrm{equation}\:\mathrm{is}\:\mathrm{established} \\ $$$$\mathrm{between}\:\mathrm{charge}\:\mathrm{density}\:\mathrm{and}\:\mathrm{current} \\ $$$$\mathrm{density} \\ $$$$\: \\ $$$$\left.\boldsymbol{\mathrm{B}}\right) \\ $$$$\mathrm{A}\:\mathrm{magnetic}\:\mathrm{field}\:\mathrm{is}\:\mathrm{generated}\:\mathrm{while}\:\mathrm{the} \\ $$$$\mathrm{capacitor}\:\mathrm{is}\:\mathrm{charged}. \\ $$$$\: \\ $$$$\left.\boldsymbol{\mathrm{C}}\right) \\ $$$$\mathrm{The}\:\mathrm{strength}\:\mathrm{of}\:\mathrm{the}\:\mathrm{electric}\:\mathrm{field}\: \\ $$$$\mathrm{produced}\:\mathrm{by}\:\mathrm{a}\:\mathrm{stationary}\:\mathrm{point}\:\mathrm{charge}\:\mathrm{is} \\ $$$$\mathrm{inversely}\:\mathrm{proportional}\:\mathrm{to}\:\mathrm{the}\:\mathrm{square}\:\mathrm{of}\:\mathrm{the} \\ $$$$\mathrm{distance} \\ $$

Answered by MrGaster last updated on 08/Feb/25

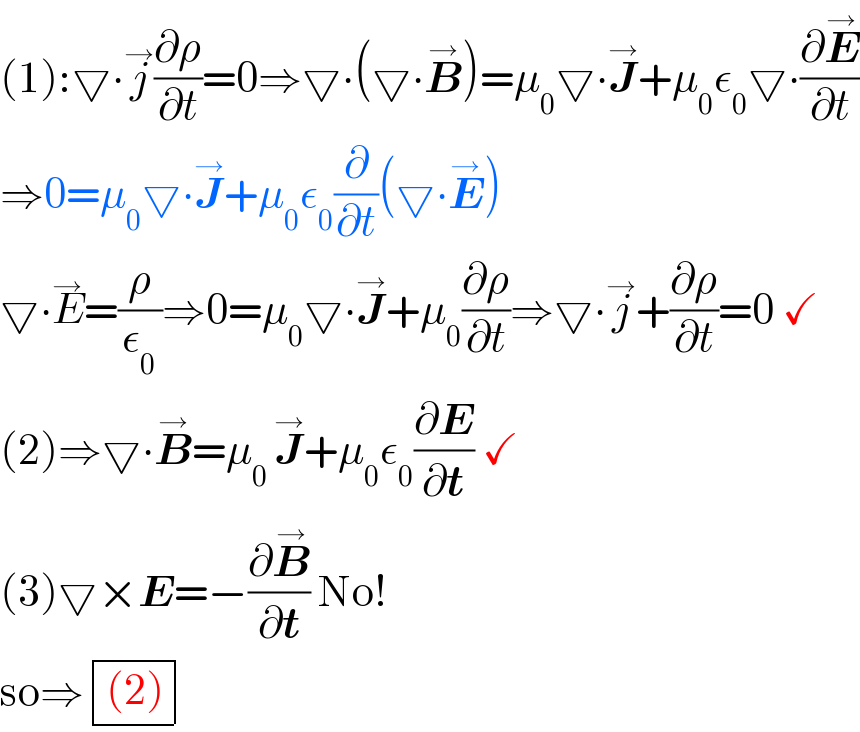
(1):▽∙j^→ (∂ρ/∂t)=0⇒▽∙(▽∙B^→ )=μ_0 ▽∙J^→ +μ_0 ε_0 ▽∙(∂E^→ /∂t) ⇒0=μ_0 ▽∙J^→ +μ_0 ε_0 (∂/∂t)(▽∙E^→ ) ▽∙E^→ =(ρ/ε_0 )⇒0=μ_0 ▽∙J^→ +μ_0 (∂ρ/∂t)⇒▽∙j^→ +(∂ρ/∂t)=0 ✓ (2)⇒▽∙B^→ =μ_(0 ) J^→ +μ_0 ε_0 (∂E/∂t) ✓ (3)▽×E=−(∂B^→ /∂t) No! so⇒ determinant ((((2))))

$$\left(\mathrm{1}\right):\bigtriangledown\centerdot\overset{\rightarrow} {{j}}\frac{\partial\rho}{\partial{t}}=\mathrm{0}\Rightarrow\bigtriangledown\centerdot\left(\bigtriangledown\centerdot\overset{\rightarrow} {\boldsymbol{{B}}}\right)=\mu_{\mathrm{0}} \bigtriangledown\centerdot\overset{\rightarrow} {\boldsymbol{{J}}}+\mu_{\mathrm{0}} \epsilon_{\mathrm{0}} \bigtriangledown\centerdot\frac{\partial\overset{\rightarrow} {\boldsymbol{{E}}}}{\partial{t}} \\ $$$$\Rightarrow\mathrm{0}=\mu_{\mathrm{0}} \bigtriangledown\centerdot\overset{\rightarrow} {\boldsymbol{{J}}}+\mu_{\mathrm{0}} \epsilon_{\mathrm{0}} \frac{\partial}{\partial{t}}\left(\bigtriangledown\centerdot\overset{\rightarrow} {\boldsymbol{{E}}}\right) \\ $$$$\bigtriangledown\centerdot\overset{\rightarrow} {{E}}=\frac{\rho}{\epsilon_{\mathrm{0}} }\Rightarrow\mathrm{0}=\mu_{\mathrm{0}} \bigtriangledown\centerdot\overset{\rightarrow} {\boldsymbol{{J}}}+\mu_{\mathrm{0}} \frac{\partial\rho}{\partial{t}}\Rightarrow\bigtriangledown\centerdot\overset{\rightarrow} {{j}}+\frac{\partial\rho}{\partial{t}}=\mathrm{0}\:\checkmark \\ $$$$\left(\mathrm{2}\right)\Rightarrow\bigtriangledown\centerdot\overset{\rightarrow} {\boldsymbol{{B}}}=\mu_{\mathrm{0}\:} \overset{\rightarrow} {\boldsymbol{{J}}}+\mu_{\mathrm{0}} \epsilon_{\mathrm{0}} \frac{\partial\boldsymbol{{E}}}{\partial\boldsymbol{{t}}}\:\checkmark \\ $$$$\left(\mathrm{3}\right)\bigtriangledown×\boldsymbol{{E}}=−\frac{\partial\overset{\rightarrow} {\boldsymbol{{B}}}}{\partial\boldsymbol{{t}}}\:\mathrm{No}! \\ $$$$\mathrm{so}\Rightarrow\begin{array}{|c|}{\left(\mathrm{2}\right)}\\\hline\end{array} \\ $$

Commented by issac last updated on 08/Feb/25

yeah∼∼ Good

$$\mathrm{yeah}\sim\sim\:\mathrm{Good} \\ $$

Answered by MathematicalUser2357 last updated on 25/Feb/25

Got

$$\mathrm{Got}\:\: \\ $$

Commented by MathematicalUser2357 last updated on 27/Apr/25

Commented by MathematicalUser2357 last updated on 27/Apr/25

"How's the new profile?" BRUH, MATHEMATICIANS ARE NOT RATING THAT. AND, DO NOT BE AN IMAGE

Terms of Service

Privacy Policy

Contact: info@tinkutara.com