
Question Number 215641 by cherokeesay last updated on 12/Jan/25

Answered by mr W last updated on 12/Jan/25
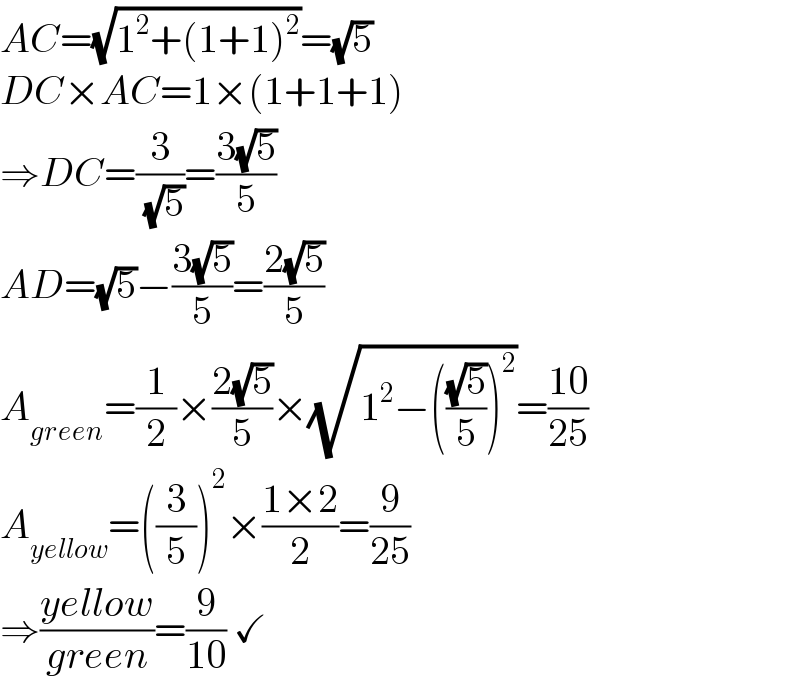
$${AC}=\sqrt{\mathrm{1}^{\mathrm{2}} +\left(\mathrm{1}+\mathrm{1}\right)^{\mathrm{2}} }=\sqrt{\mathrm{5}} \\ $$$${DC}×{AC}=\mathrm{1}×\left(\mathrm{1}+\mathrm{1}+\mathrm{1}\right)\: \\ $$$$\Rightarrow{DC}=\frac{\mathrm{3}}{\:\sqrt{\mathrm{5}}}=\frac{\mathrm{3}\sqrt{\mathrm{5}}}{\mathrm{5}} \\ $$$${AD}=\sqrt{\mathrm{5}}−\frac{\mathrm{3}\sqrt{\mathrm{5}}}{\mathrm{5}}=\frac{\mathrm{2}\sqrt{\mathrm{5}}}{\mathrm{5}} \\ $$$${A}_{{green}} =\frac{\mathrm{1}}{\mathrm{2}}×\frac{\mathrm{2}\sqrt{\mathrm{5}}}{\mathrm{5}}×\sqrt{\mathrm{1}^{\mathrm{2}} −\left(\frac{\sqrt{\mathrm{5}}}{\mathrm{5}}\right)^{\mathrm{2}} }=\frac{\mathrm{10}}{\mathrm{25}} \\ $$$${A}_{{yellow}} =\left(\frac{\mathrm{3}}{\mathrm{5}}\right)^{\mathrm{2}} ×\frac{\mathrm{1}×\mathrm{2}}{\mathrm{2}}=\frac{\mathrm{9}}{\mathrm{25}} \\ $$$$\Rightarrow\frac{{yellow}}{{green}}=\frac{\mathrm{9}}{\mathrm{10}}\:\checkmark \\ $$
Commented by cherokeesay last updated on 12/Jan/25

$${so}\:{nice}\:! \\ $$$${thank}\:{you}\:{master}\:! \\ $$$$\:\underline{\underbrace{\lesseqgtr}} \\ $$
