
Question Number 211455 by BaliramKumar last updated on 09/Sep/24
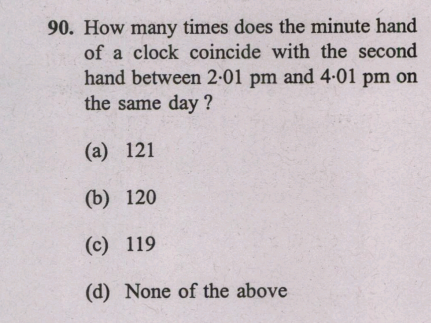
Answered by BHOOPENDRA last updated on 10/Sep/24
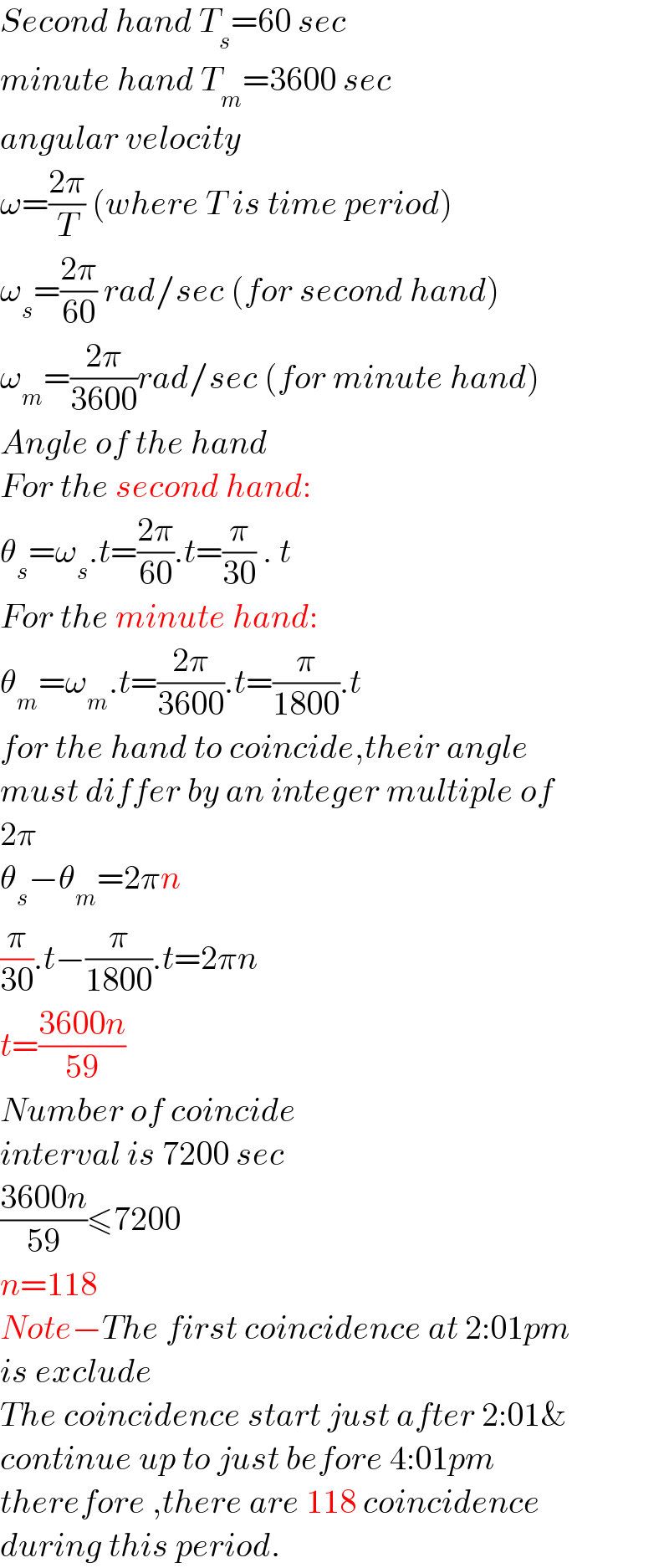
$${Second}\:{hand}\:{T}_{{s}} =\mathrm{60}\:{sec} \\ $$$${minute}\:{hand}\:{T}_{{m}} =\mathrm{3600}\:{sec} \\ $$$${angular}\:{velocity} \\ $$$$\omega=\frac{\mathrm{2}\pi}{{T}}\:\left({where}\:{T}\:{is}\:{time}\:{period}\right) \\ $$$$\omega_{{s}} =\frac{\mathrm{2}\pi}{\mathrm{60}}\:{rad}/{sec}\:\left({for}\:{second}\:{hand}\right) \\ $$$$\omega_{{m}} =\frac{\mathrm{2}\pi}{\mathrm{3600}}{rad}/{sec}\:\left({for}\:{minute}\:{hand}\right) \\ $$$${Angle}\:{of}\:{the}\:{hand}\: \\ $$$${For}\:{the}\:{second}\:{hand}: \\ $$$$\theta_{{s}} =\omega_{{s}} .{t}=\frac{\mathrm{2}\pi}{\mathrm{60}}.{t}=\frac{\pi}{\mathrm{30}}\:.\:{t} \\ $$$${For}\:{the}\:{minute}\:{hand}: \\ $$$$\theta_{{m}} =\omega_{{m}} .{t}=\frac{\mathrm{2}\pi}{\mathrm{3600}}.{t}=\frac{\pi}{\mathrm{1800}}.{t} \\ $$$${for}\:{the}\:{hand}\:{to}\:{coincide},{their}\:{angle} \\ $$$${must}\:{differ}\:{by}\:{an}\:{integer}\:{multiple}\:{of} \\ $$$$\mathrm{2}\pi \\ $$$$\theta_{{s}} −\theta_{{m}} =\mathrm{2}\pi{n} \\ $$$$\frac{\pi}{\mathrm{30}}.{t}−\frac{\pi}{\mathrm{1800}}.{t}=\mathrm{2}\pi{n} \\ $$$${t}=\frac{\mathrm{3600}{n}}{\mathrm{59}} \\ $$$${Number}\:{of}\:{coincide} \\ $$$${interval}\:{is}\:\mathrm{7200}\:{sec} \\ $$$$\frac{\mathrm{3600}{n}}{\mathrm{59}}\leqslant\mathrm{7200} \\ $$$${n}=\mathrm{118} \\ $$$${Note}−{The}\:{first}\:{coincidence}\:{at}\:\mathrm{2}:\mathrm{01}{pm} \\ $$$${is}\:{exclude} \\ $$$${The}\:{coincidence}\:{start}\:{just}\:{after}\:\mathrm{2}:\mathrm{01\&} \\ $$$${continue}\:{up}\:{to}\:{just}\:{before}\:\mathrm{4}:\mathrm{01}{pm} \\ $$$${therefore}\:,{there}\:{are}\:\mathrm{118}\:{coincidence} \\ $$$${during}\:{this}\:{period}. \\ $$
Answered by mr W last updated on 10/Sep/24

$${at}\:{h}:\mathrm{0}:\mathrm{0}\:{the}\:{minute}\:{hand}\:{and}\:{second} \\ $$$${hand}\:{coincide}. \\ $$$${say}\:{at}\:{h}:{m}:{s}\:{they}\:{coincide}\:{again}. \\ $$$$\mathrm{0}\leqslant{m}\leqslant\mathrm{59} \\ $$$$\mathrm{0}\leqslant{s}<\mathrm{60} \\ $$$$\mathrm{6}\left({m}+\frac{{s}}{\mathrm{60}}\right)=\mathrm{6}{s}\:\Rightarrow{m}=\frac{\mathrm{59}{s}}{\mathrm{60}}\:\Rightarrow{s}=\frac{\mathrm{60}{m}}{\mathrm{59}} \\ $$$$\Rightarrow\mathrm{0}\leqslant{s}=\frac{\mathrm{60}{m}}{\mathrm{59}}<\mathrm{60}\:\Rightarrow\mathrm{0}\leqslant{m}\leqslant\mathrm{58} \\ $$$${m}=\mathrm{0},\mathrm{1},...,\mathrm{58}\:\Rightarrow\mathrm{59}\:{times}\:{in}\:{a}\:{hour} \\ $$$$\mathrm{2}:\mathrm{01}\:\Rightarrow\mathrm{58} \\ $$$$\mathrm{3}:\mathrm{00}\:\Rightarrow\mathrm{59} \\ $$$$\mathrm{4}:\mathrm{01}\:\Rightarrow\mathrm{1} \\ $$$$\Sigma:\:\mathrm{118}\:{times}\:\checkmark \\ $$
Commented by mr W last updated on 11/Sep/24

$${for}\:{a}\:{mechanic}\:{clock}\:{the}\:{minute} \\ $$$${hand}\:{coincides}\:{with}\:{the}\:{second}\:{hand} \\ $$$${at}\:{following}\:{moments}\:{in}\:{a}\:{hour}: \\ $$$${h}:\mathrm{00}:\mathrm{00} \\ $$$${h}:\mathrm{01}:\mathrm{01} \\ $$$${h}:\mathrm{02}:\mathrm{02} \\ $$$$... \\ $$$${h}:\mathrm{59}:\mathrm{59} \\ $$$${totally}\:\mathrm{60}\:{times}\:{in}\:{a}\:{hours}. \\ $$$${in}\:{the}\:{period}\:\mathrm{2}:\mathrm{01}\:{till}\:\mathrm{4}:\mathrm{01}\:{they} \\ $$$${coincide}\:\mathrm{120}\:{times}. \\ $$
