
Question Number 210767 by lmcp1203 last updated on 18/Aug/24
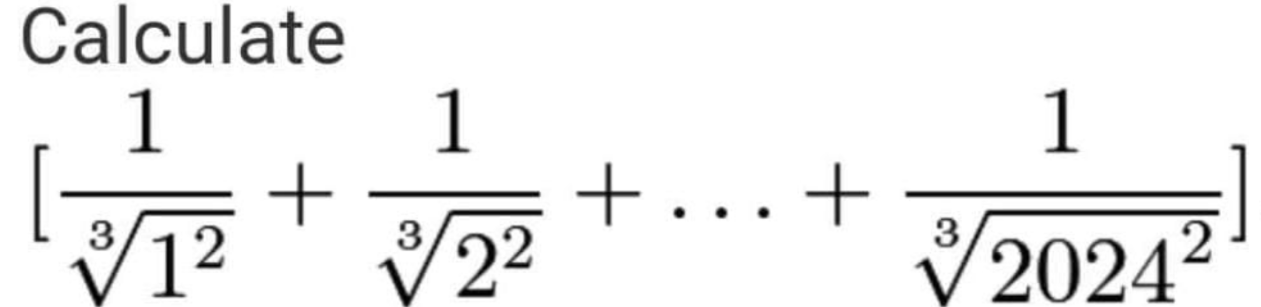
Commented by Frix last updated on 19/Aug/24

$$\mathrm{35} \\ $$
Commented by lmcp1203 last updated on 19/Aug/24
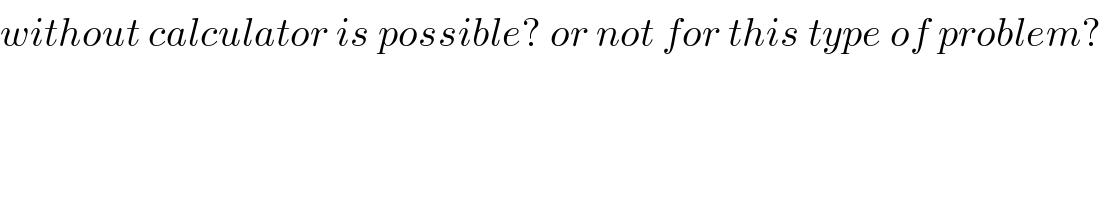
$${without}\:{calculator}\:{is}\:{possible}?\:{or}\:{not}\:{for}\:{this}\:{type}\:{of}\:{problem}? \\ $$
Answered by BHOOPENDRA last updated on 19/Aug/24

$$\underset{{n}=\mathrm{1}} {\overset{{n}=\mathrm{2024}} {\sum}}\frac{\mathrm{1}}{{n}^{\frac{\mathrm{2}}{\mathrm{3}}} } \\ $$$$\Rightarrow\:\mathrm{35}\:{approx}\: \\ $$$$ \\ $$
Answered by Berbere last updated on 19/Aug/24

$${yes}\:\underset{{k}=\mathrm{1}} {\overset{{m}} {\sum}}\frac{\mathrm{1}}{{k}^{\frac{\mathrm{2}}{\mathrm{3}}} } \\ $$$${zeta}/{hurwitz}\:{function} \\ $$$$ \\ $$
Commented by lmcp1203 last updated on 19/Aug/24

$${thank}\:{you} \\ $$
