Question and Answers Forum

All Questions      Topic List

Geometry Questions

Previous in All Question      Next in All Question      

Previous in Geometry      Next in Geometry      

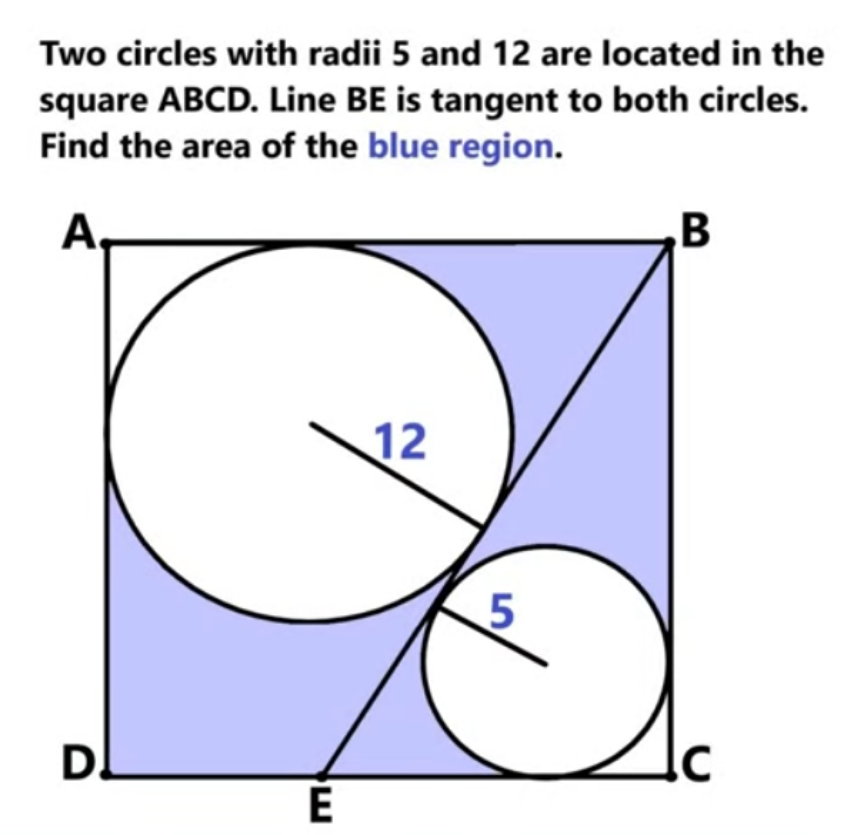
Question Number 205428 by mr W last updated on 21/Mar/24

Answered by A5T last updated on 21/Mar/24

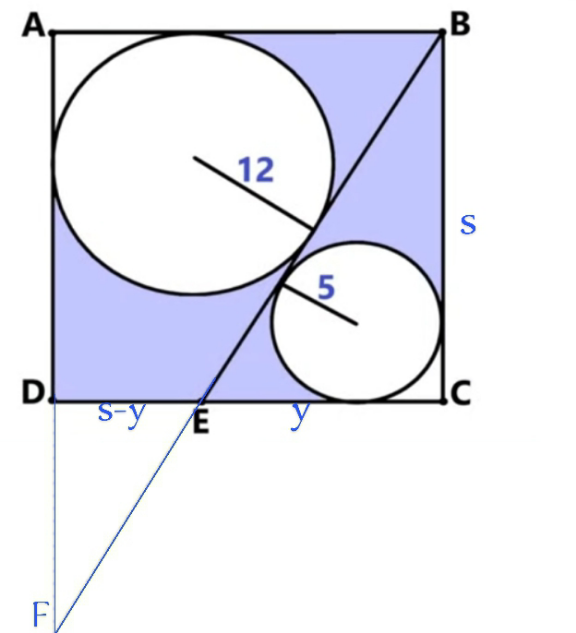
Commented by A5T last updated on 21/Mar/24

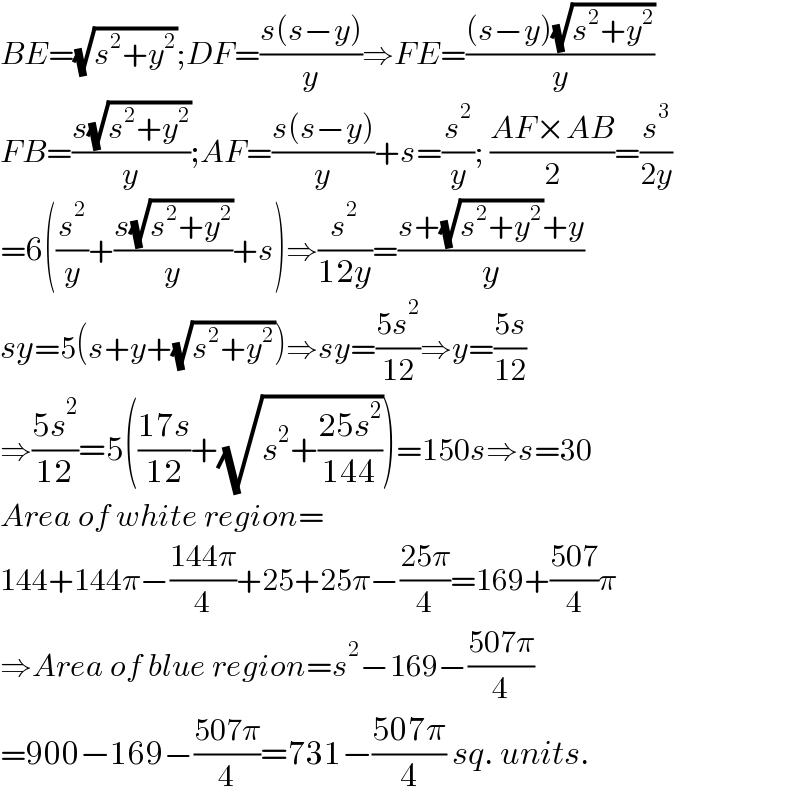
BE=(√(s^2 +y^2 ));DF=((s(s−y))/y)⇒FE=(((s−y)(√(s^2 +y^2 )))/y) FB=((s(√(s^2 +y^2 )))/y);AF=((s(s−y))/y)+s=(s^2 /y); ((AF×AB)/2)=(s^3 /(2y)) =6((s^2 /y)+((s(√(s^2 +y^2 )))/y)+s)⇒(s^2 /(12y))=((s+(√(s^2 +y^2 ))+y)/y) sy=5(s+y+(√(s^2 +y^2 )))⇒sy=((5s^2 )/(12))⇒y=((5s)/(12)) ⇒((5s^2 )/(12))=5(((17s)/(12))+(√(s^2 +((25s^2 )/(144)))))=150s⇒s=30 Area of white region= 144+144π−((144π)/4)+25+25π−((25π)/4)=169+((507)/4)π ⇒Area of blue region=s^2 −169−((507π)/4) =900−169−((507π)/4)=731−((507π)/4) sq. units.

$${BE}=\sqrt{{s}^{\mathrm{2}} +{y}^{\mathrm{2}} };{DF}=\frac{{s}\left({s}−{y}\right)}{{y}}\Rightarrow{FE}=\frac{\left({s}−{y}\right)\sqrt{{s}^{\mathrm{2}} +{y}^{\mathrm{2}} }}{{y}} \\ $$$${FB}=\frac{{s}\sqrt{{s}^{\mathrm{2}} +{y}^{\mathrm{2}} }}{{y}};{AF}=\frac{{s}\left({s}−{y}\right)}{{y}}+{s}=\frac{{s}^{\mathrm{2}} }{{y}};\:\frac{{AF}×{AB}}{\mathrm{2}}=\frac{{s}^{\mathrm{3}} }{\mathrm{2}{y}} \\ $$$$=\mathrm{6}\left(\frac{{s}^{\mathrm{2}} }{{y}}+\frac{{s}\sqrt{{s}^{\mathrm{2}} +{y}^{\mathrm{2}} }}{{y}}+{s}\right)\Rightarrow\frac{{s}^{\mathrm{2}} }{\mathrm{12}{y}}=\frac{{s}+\sqrt{{s}^{\mathrm{2}} +{y}^{\mathrm{2}} }+{y}}{{y}} \\ $$$${sy}=\mathrm{5}\left({s}+{y}+\sqrt{{s}^{\mathrm{2}} +{y}^{\mathrm{2}} }\right)\Rightarrow{sy}=\frac{\mathrm{5}{s}^{\mathrm{2}} }{\mathrm{12}}\Rightarrow{y}=\frac{\mathrm{5}{s}}{\mathrm{12}} \\ $$$$\Rightarrow\frac{\mathrm{5}{s}^{\mathrm{2}} }{\mathrm{12}}=\mathrm{5}\left(\frac{\mathrm{17}{s}}{\mathrm{12}}+\sqrt{{s}^{\mathrm{2}} +\frac{\mathrm{25}{s}^{\mathrm{2}} }{\mathrm{144}}}\right)=\mathrm{150}{s}\Rightarrow{s}=\mathrm{30} \\ $$$${Area}\:{of}\:{white}\:{region}= \\ $$$$\mathrm{144}+\mathrm{144}\pi−\frac{\mathrm{144}\pi}{\mathrm{4}}+\mathrm{25}+\mathrm{25}\pi−\frac{\mathrm{25}\pi}{\mathrm{4}}=\mathrm{169}+\frac{\mathrm{507}}{\mathrm{4}}\pi \\ $$$$\Rightarrow{Area}\:{of}\:{blue}\:{region}={s}^{\mathrm{2}} −\mathrm{169}−\frac{\mathrm{507}\pi}{\mathrm{4}} \\ $$$$=\mathrm{900}−\mathrm{169}−\frac{\mathrm{507}\pi}{\mathrm{4}}=\mathrm{731}−\frac{\mathrm{507}\pi}{\mathrm{4}}\:{sq}.\:{units}. \\ $$

Commented by mr W last updated on 21/Mar/24

��

Answered by mr W last updated on 21/Mar/24

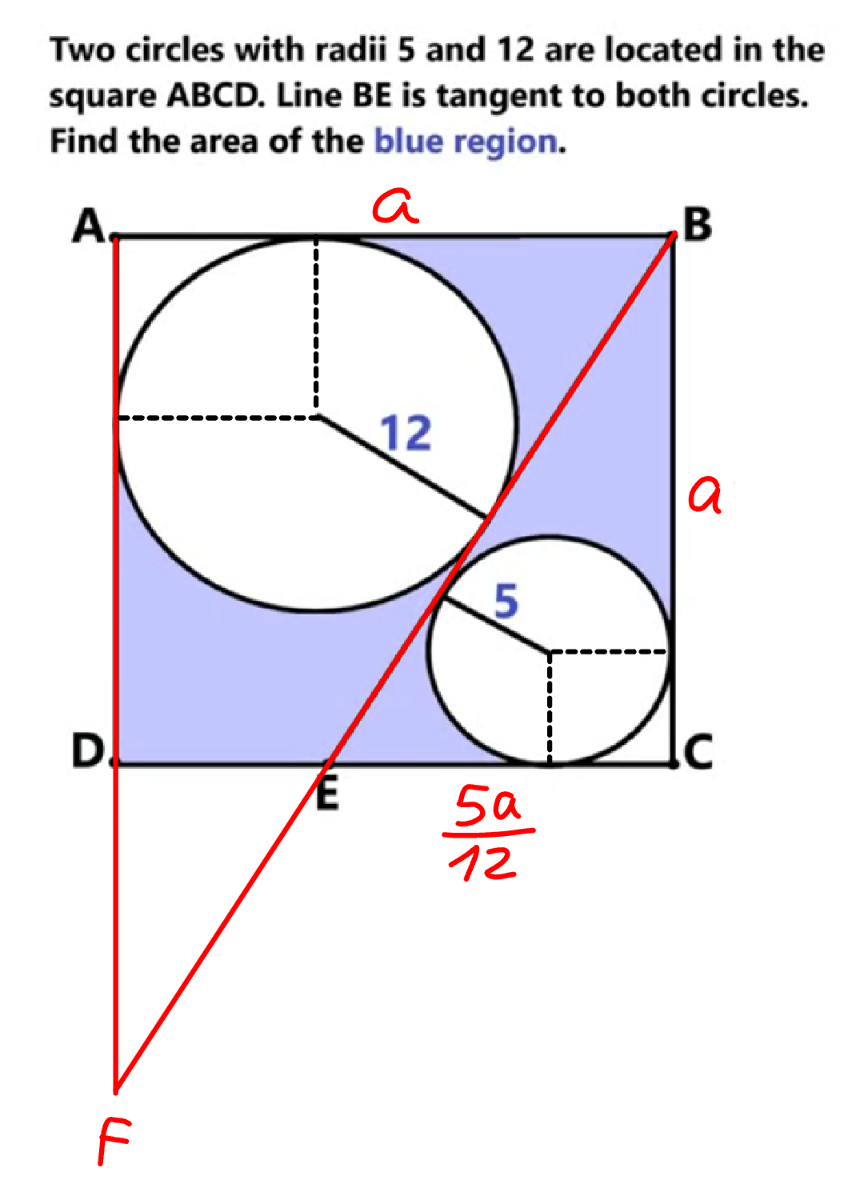
Commented by mr W last updated on 21/Mar/24

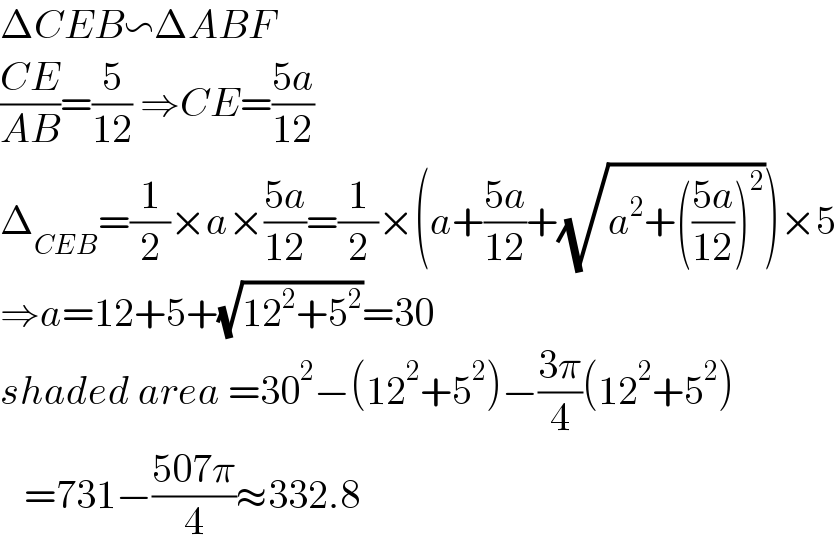
ΔCEB∽ΔABF ((CE)/(AB))=(5/(12)) ⇒CE=((5a)/(12)) Δ_(CEB) =(1/2)×a×((5a)/(12))=(1/2)×(a+((5a)/(12))+(√(a^2 +(((5a)/(12)))^2 )))×5 ⇒a=12+5+(√(12^2 +5^2 ))=30 shaded area =30^2 −(12^2 +5^2 )−((3π)/4)(12^2 +5^2 ) =731−((507π)/4)≈332.8

$$\Delta{CEB}\backsim\Delta{ABF} \\ $$$$\frac{{CE}}{{AB}}=\frac{\mathrm{5}}{\mathrm{12}}\:\Rightarrow{CE}=\frac{\mathrm{5}{a}}{\mathrm{12}} \\ $$$$\Delta_{{CEB}} =\frac{\mathrm{1}}{\mathrm{2}}×{a}×\frac{\mathrm{5}{a}}{\mathrm{12}}=\frac{\mathrm{1}}{\mathrm{2}}×\left({a}+\frac{\mathrm{5}{a}}{\mathrm{12}}+\sqrt{{a}^{\mathrm{2}} +\left(\frac{\mathrm{5}{a}}{\mathrm{12}}\right)^{\mathrm{2}} }\right)×\mathrm{5} \\ $$$$\Rightarrow{a}=\mathrm{12}+\mathrm{5}+\sqrt{\mathrm{12}^{\mathrm{2}} +\mathrm{5}^{\mathrm{2}} }=\mathrm{30} \\ $$$${shaded}\:{area}\:=\mathrm{30}^{\mathrm{2}} −\left(\mathrm{12}^{\mathrm{2}} +\mathrm{5}^{\mathrm{2}} \right)−\frac{\mathrm{3}\pi}{\mathrm{4}}\left(\mathrm{12}^{\mathrm{2}} +\mathrm{5}^{\mathrm{2}} \right) \\ $$$$\:\:\:=\mathrm{731}−\frac{\mathrm{507}\pi}{\mathrm{4}}\approx\mathrm{332}.\mathrm{8} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com