
Question Number 203985 by ajfour last updated on 03/Feb/24
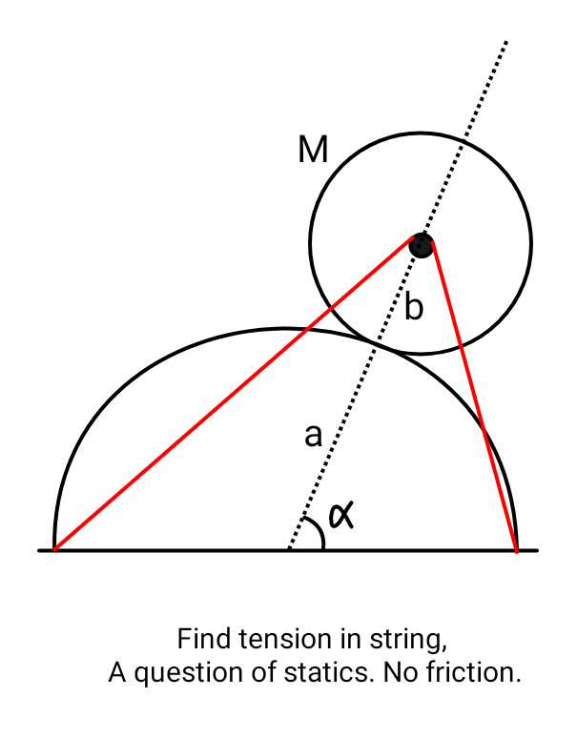
Commented by mr W last updated on 03/Feb/24
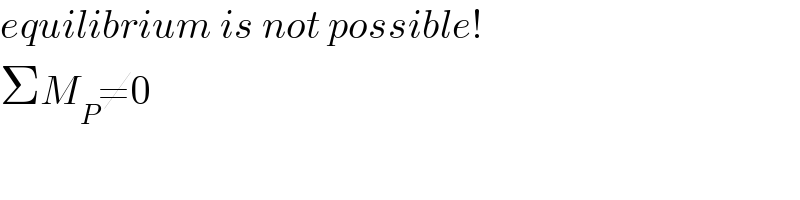
$${equilibrium}\:{is}\:{not}\:{possible}! \\ $$$$\Sigma{M}_{{P}} \neq\mathrm{0} \\ $$
Commented by mr W last updated on 03/Feb/24
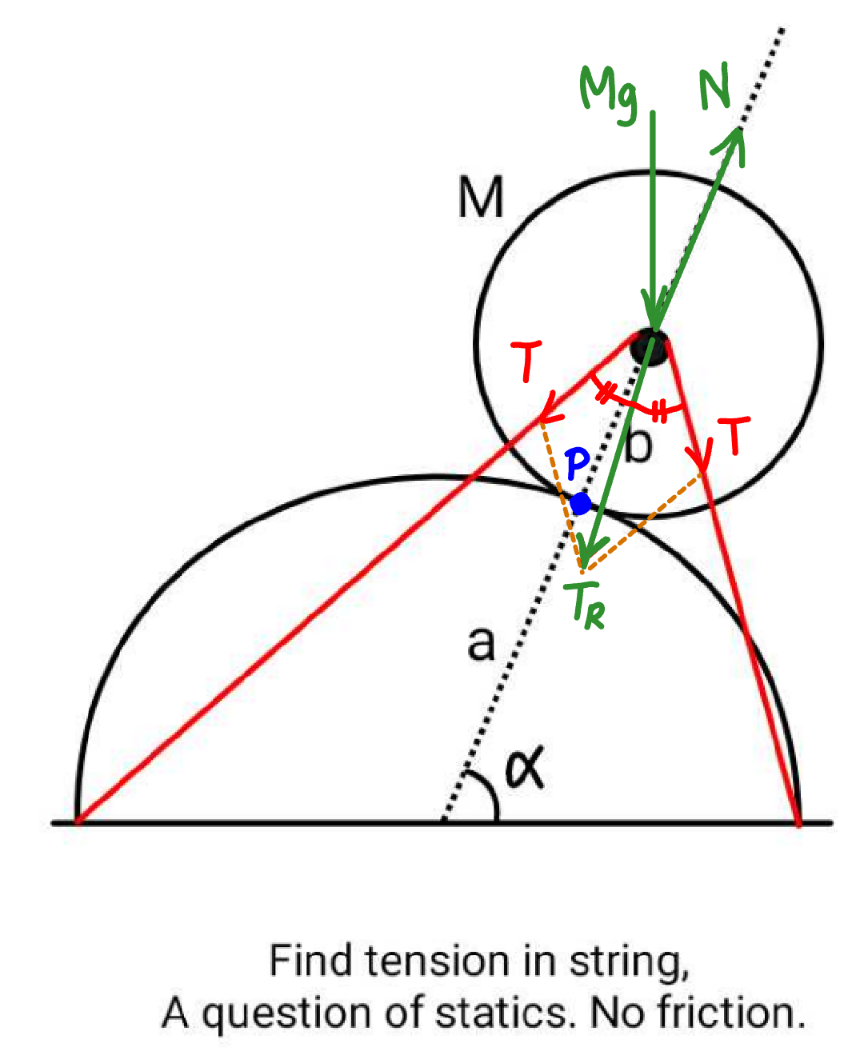
Commented by ajfour last updated on 03/Feb/24

$${If}\:{we}\:{assume}\:{friction},\:{can}\:{we}\: \\ $$$${determine}\:{both}\:{tension}\:{and}\: \\ $$$${existing}\:{friction},\:{coefficient}\:\mu\:{not} \\ $$$${given}\:{but}\:{that}\:{equilibrium}\:{persists}? \\ $$
Commented by mr W last updated on 04/Feb/24

$${even}\:{with}\:{friction}\:{no}\:{equilibrium} \\ $$$${is}\:{possible}. \\ $$$${the}\:{resultant}\:{force}\:\left({T}_{{R}} \:{in}\:{diagram}\right) \\ $$$${of}\:{the}\:{tension}\:{in}\:{string}\:{lies}\:{in}\:{the} \\ $$$${direction}\:{of}\:{angle}\:{bisector}.\:{this}\:{force} \\ $$$${and}\:{the}\:{weight}\:\left({Mg}\right)\:{of}\:{the}\:{cylinder} \\ $$$${always}\:{bring}\:{the}\:{cylinder}\:{to}\:{rotate} \\ $$$${clockwise}.\:{the}\:{friction}\:{can}\:{not} \\ $$$${prevent}\:{this}\:{and}\:{keep}\:{the}\:{cylinder}\: \\ $$$${in}\:{equilibrium}. \\ $$$${in}\:{the}\:{first}\:{case}\:{of}\:{following}\: \\ $$$${diagrams}\:{an}\:{equilibrium}\:{is}\:{possible}. \\ $$$${but}\:{we}\:{have}\:{the}\:{second}\:{case}. \\ $$
Commented by mr W last updated on 04/Feb/24

Commented by mr W last updated on 04/Feb/24

Commented by ajfour last updated on 04/Feb/24

$${Yes}\:{true},\:{unless}\:\theta=\frac{\pi}{\mathrm{2}}\:{equilibrium} \\ $$$${not}\:{possible}.\:{We}\:{need}\:{have}\:{friction} \\ $$$${at}\:{axle}\:{of}\:{upper}\:{cylinder}\:{and}\:{string}. \\ $$
Commented by mr W last updated on 04/Feb/24

$${yes}\:{sir}! \\ $$
