
Question Number 203211 by ajfour last updated on 12/Jan/24
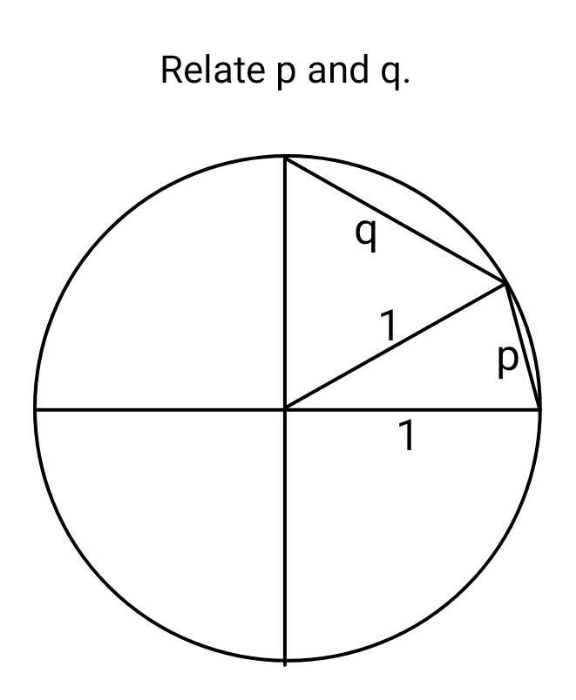
Answered by MM42 last updated on 12/Jan/24
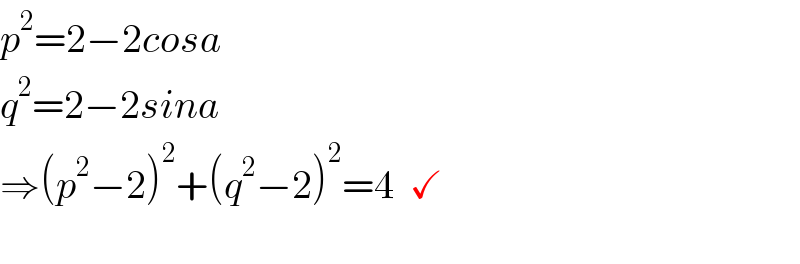
$${p}^{\mathrm{2}} =\mathrm{2}−\mathrm{2}{cosa} \\ $$$${q}^{\mathrm{2}} =\mathrm{2}−\mathrm{2}{sina} \\ $$$$\Rightarrow\left({p}^{\mathrm{2}} −\mathrm{2}\right)^{\mathrm{2}} +\left({q}^{\mathrm{2}} −\mathrm{2}\right)^{\mathrm{2}} =\mathrm{4}\:\:\checkmark \\ $$$$ \\ $$
Commented by ajfour last updated on 12/Jan/24

$${I}\:{like}\:{it}.\:{precise}! \\ $$
Commented by BaliramKumar last updated on 12/Jan/24

$${p}^{\mathrm{2}} \:\leq\:\mathrm{2}\:\:\&\:{q}^{\mathrm{2}} \:\leq\:\mathrm{2} \\ $$
Commented by MM42 last updated on 12/Jan/24

$$\:\underline{\underbrace{\lesseqgtr}} \\ $$
