
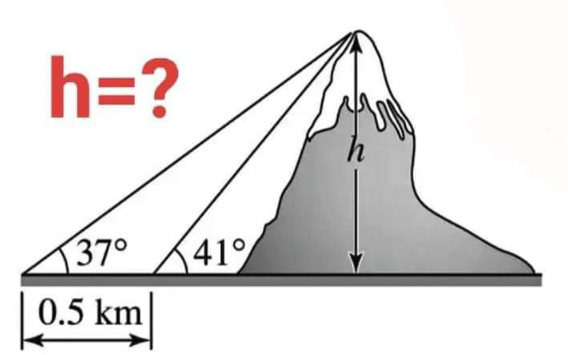
Question Number 202893 by sonukgindia last updated on 05/Jan/24
Answered by BaliramKumar last updated on 05/Jan/24
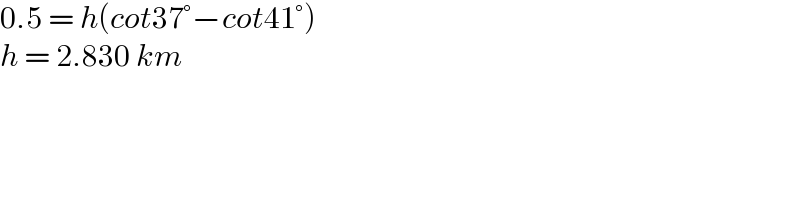
$$\mathrm{0}.\mathrm{5}\:=\:{h}\left({cot}\mathrm{37}°−{cot}\mathrm{41}°\right) \\ $$$${h}\:=\:\mathrm{2}.\mathrm{830}\:{km} \\ $$
Answered by deleteduser1 last updated on 05/Jan/24
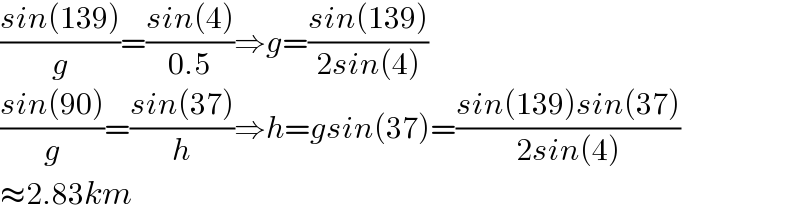
$$\frac{{sin}\left(\mathrm{139}\right)}{{g}}=\frac{{sin}\left(\mathrm{4}\right)}{\mathrm{0}.\mathrm{5}}\Rightarrow{g}=\frac{{sin}\left(\mathrm{139}\right)}{\mathrm{2}{sin}\left(\mathrm{4}\right)} \\ $$$$\frac{{sin}\left(\mathrm{90}\right)}{{g}}=\frac{{sin}\left(\mathrm{37}\right)}{{h}}\Rightarrow{h}={gsin}\left(\mathrm{37}\right)=\frac{{sin}\left(\mathrm{139}\right){sin}\left(\mathrm{37}\right)}{\mathrm{2}{sin}\left(\mathrm{4}\right)} \\ $$$$\approx\mathrm{2}.\mathrm{83}{km} \\ $$
