
Question Number 200420 by ajfour last updated on 18/Nov/23
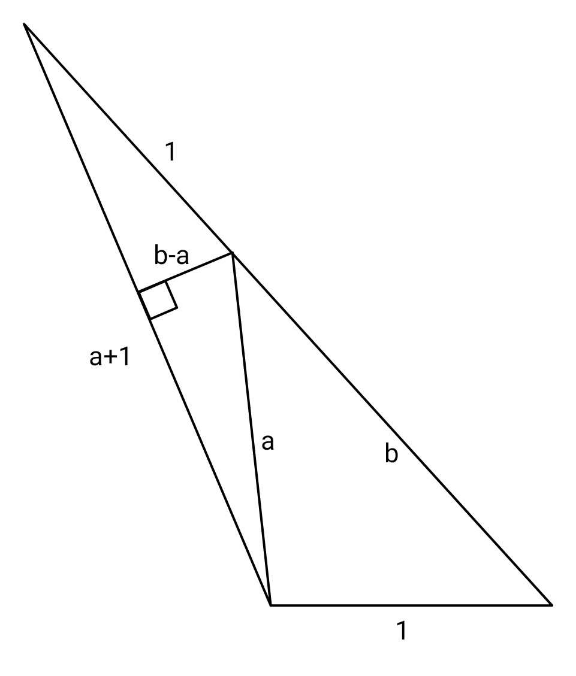
Commented by ajfour last updated on 18/Nov/23

$${plz}\:{solve}.. \\ $$
Commented by ajfour last updated on 18/Nov/23
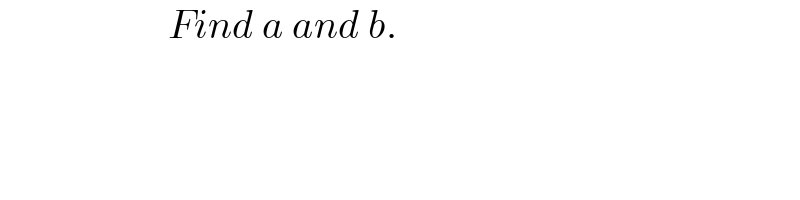
$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:{Find}\:{a}\:{and}\:{b}. \\ $$
Commented by deleteduser1 last updated on 18/Nov/23
![(√(1−(b−a)^2 ))+(√(a^2 −(b−a)^2 ))=a+(2/3) [a+(2/3)−(√(2ba−b^2 ))][a+(2/3)]=1×[1+x]...(i) (√(a^2 −x^2 ))=y=(√(1^2 −(b−x)^2 ))⇒a^2 =1−b^2 +2bx...(ii) (i)∧(ii)⇒a^2 +(4/3)a+(4/9)−a(√(2ba−b^2 ))−(2/3)(√(2ba−b^2 ))−1=x =((a^2 +b^2 −1)/(2b))[This is the relationship between a&b]](Q200445.png)
$$\sqrt{\mathrm{1}−\left({b}−{a}\right)^{\mathrm{2}} }+\sqrt{{a}^{\mathrm{2}} −\left({b}−{a}\right)^{\mathrm{2}} }={a}+\frac{\mathrm{2}}{\mathrm{3}} \\ $$$$\left[{a}+\frac{\mathrm{2}}{\mathrm{3}}−\sqrt{\mathrm{2}{ba}−{b}^{\mathrm{2}} }\right]\left[{a}+\frac{\mathrm{2}}{\mathrm{3}}\right]=\mathrm{1}×\left[\mathrm{1}+{x}\right]...\left({i}\right) \\ $$$$\sqrt{{a}^{\mathrm{2}} −{x}^{\mathrm{2}} }={y}=\sqrt{\mathrm{1}^{\mathrm{2}} −\left({b}−{x}\right)^{\mathrm{2}} }\Rightarrow{a}^{\mathrm{2}} =\mathrm{1}−{b}^{\mathrm{2}} +\mathrm{2}{bx}...\left({ii}\right) \\ $$$$\left({i}\right)\wedge\left({ii}\right)\Rightarrow{a}^{\mathrm{2}} +\frac{\mathrm{4}}{\mathrm{3}}{a}+\frac{\mathrm{4}}{\mathrm{9}}−{a}\sqrt{\mathrm{2}{ba}−{b}^{\mathrm{2}} }−\frac{\mathrm{2}}{\mathrm{3}}\sqrt{\mathrm{2}{ba}−{b}^{\mathrm{2}} }−\mathrm{1}={x} \\ $$$$=\frac{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} −\mathrm{1}}{\mathrm{2}{b}}\left[{This}\:{is}\:{the}\:{relationship}\:{between}\:{a\&b}\right] \\ $$
Commented by mr W last updated on 18/Nov/23

$${impossible}\:{figure}! \\ $$$${triangle}\:{with}\:{sides}:\:\mathrm{1},\:{a},\:\mathrm{1}+{a} \\ $$
Commented by deleteduser1 last updated on 18/Nov/23
$${Exactly},{the}\:{triangle}\:{has}\:{to}\:{be}\:{a}\:{line}. \\ $$
Commented by ajfour last updated on 18/Nov/23
$${yeah}\:{sorry},\:{lets}\:{have}\:{instead}\: \\ $$$${of}\:{the}\:{side}\:{a}+\mathrm{1}\:\:{we}\:{have}\:\:{a}+\frac{\mathrm{2}}{\mathrm{3}}\:. \\ $$
