
Question Number 200356 by sonukgindia last updated on 17/Nov/23

Commented by mr W last updated on 17/Nov/23
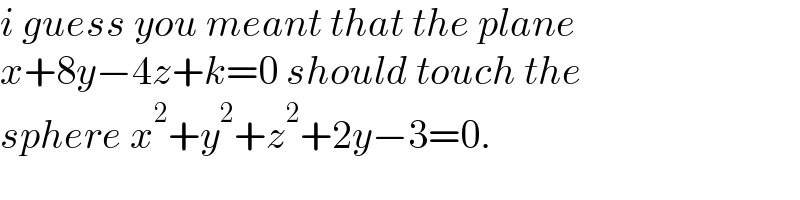
$${i}\:{guess}\:{you}\:{meant}\:{that}\:{the}\:{plane}\: \\ $$$${x}+\mathrm{8}{y}−\mathrm{4}{z}+{k}=\mathrm{0}\:{should}\:{touch}\:{the} \\ $$$${sphere}\:{x}^{\mathrm{2}} +{y}^{\mathrm{2}} +{z}^{\mathrm{2}} +\mathrm{2}{y}−\mathrm{3}=\mathrm{0}. \\ $$
Answered by mr W last updated on 17/Nov/23
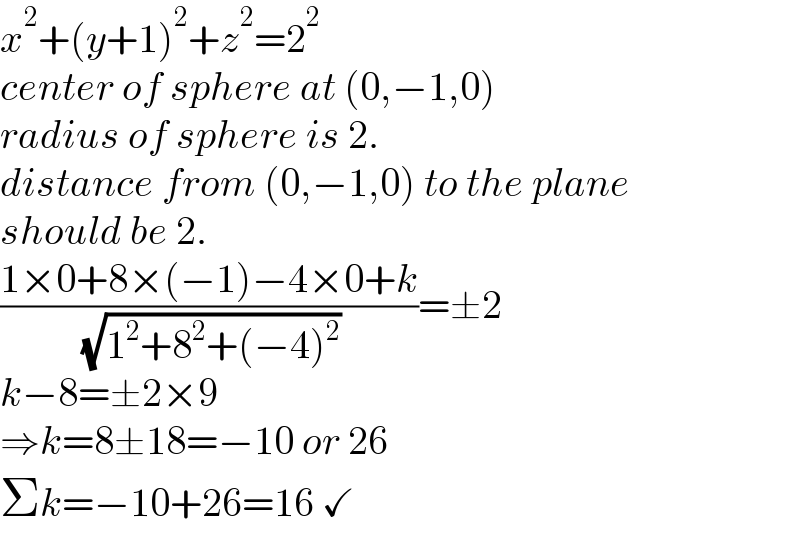
$${x}^{\mathrm{2}} +\left({y}+\mathrm{1}\right)^{\mathrm{2}} +{z}^{\mathrm{2}} =\mathrm{2}^{\mathrm{2}} \\ $$$${center}\:{of}\:{sphere}\:{at}\:\left(\mathrm{0},−\mathrm{1},\mathrm{0}\right) \\ $$$${radius}\:{of}\:{sphere}\:{is}\:\mathrm{2}. \\ $$$${distance}\:{from}\:\left(\mathrm{0},−\mathrm{1},\mathrm{0}\right)\:{to}\:{the}\:{plane} \\ $$$${should}\:{be}\:\mathrm{2}. \\ $$$$\frac{\mathrm{1}×\mathrm{0}+\mathrm{8}×\left(−\mathrm{1}\right)−\mathrm{4}×\mathrm{0}+{k}}{\:\sqrt{\mathrm{1}^{\mathrm{2}} +\mathrm{8}^{\mathrm{2}} +\left(−\mathrm{4}\right)^{\mathrm{2}} }}=\pm\mathrm{2} \\ $$$${k}−\mathrm{8}=\pm\mathrm{2}×\mathrm{9} \\ $$$$\Rightarrow{k}=\mathrm{8}\pm\mathrm{18}=−\mathrm{10}\:{or}\:\mathrm{26} \\ $$$$\Sigma{k}=−\mathrm{10}+\mathrm{26}=\mathrm{16}\:\checkmark \\ $$
