
Question Number 199592 by hardmath last updated on 05/Nov/23
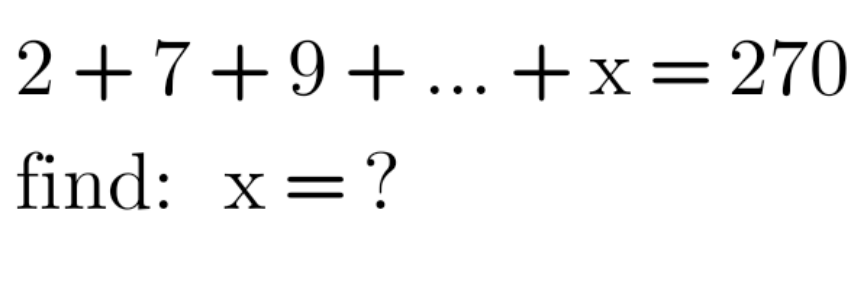
Commented by mr W last updated on 06/Nov/23
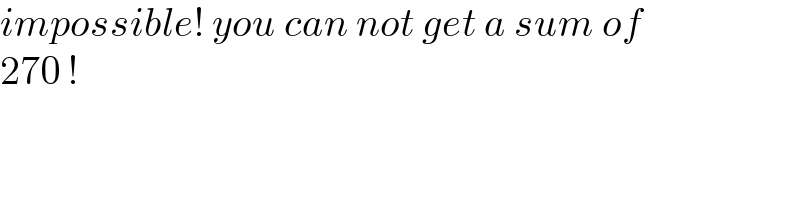
$${impossible}!\:{you}\:{can}\:{not}\:{get}\:{a}\:{sum}\:{of} \\ $$$$\mathrm{270}\:! \\ $$
Commented by mr W last updated on 05/Nov/23

$${the}\:{sequence}\:{is}\:{not}\:{clearly}\:{specified}! \\ $$$$\mathrm{2},\:\mathrm{7},\:\mathrm{9},\:\mathrm{16}?,\:\mathrm{25}?,\:.... \\ $$
Commented by hardmath last updated on 05/Nov/23
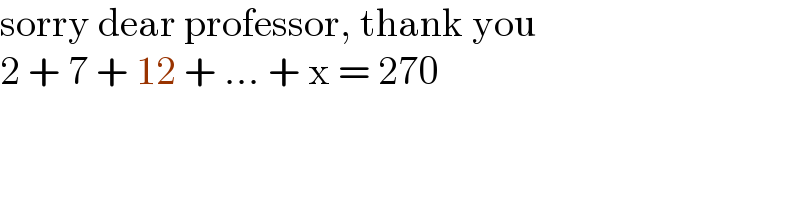
$$\mathrm{sorry}\:\mathrm{dear}\:\mathrm{professor},\:\mathrm{thank}\:\mathrm{you} \\ $$$$\mathrm{2}\:+\:\mathrm{7}\:+\:\mathrm{12}\:+\:...\:+\:\mathrm{x}\:=\:\mathrm{270} \\ $$
Commented by hardmath last updated on 06/Nov/23
$$\mathrm{thank}\:\mathrm{you}\:\mathrm{dear}\:\mathrm{professor} \\ $$
Commented by hardmath last updated on 06/Nov/23

$$\mathrm{my}\:\mathrm{dear}\:\mathrm{professor}, \\ $$$$\mathrm{how}\:\mathrm{we}\:\mathrm{write}\:\mathrm{it}\:\mathrm{is}\:\mathrm{correct} \\ $$
Commented by mr W last updated on 06/Nov/23
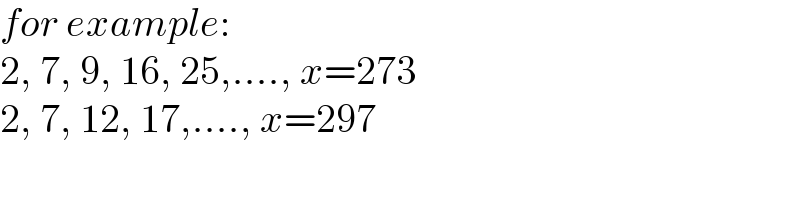
$${for}\:{example}: \\ $$$$\mathrm{2},\:\mathrm{7},\:\mathrm{9},\:\mathrm{16},\:\mathrm{25},....,\:{x}=\mathrm{273} \\ $$$$\mathrm{2},\:\mathrm{7},\:\mathrm{12},\:\mathrm{17},....,\:{x}=\mathrm{297} \\ $$
Commented by hardmath last updated on 06/Nov/23
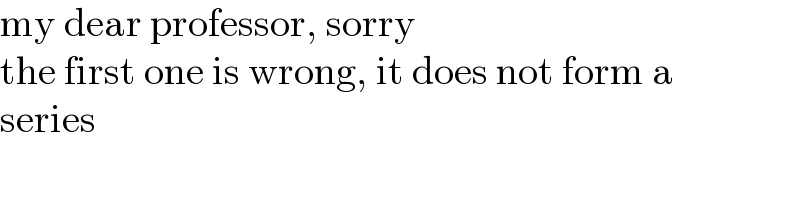
$$\mathrm{my}\:\mathrm{dear}\:\mathrm{professor},\:\mathrm{sorry} \\ $$$$\mathrm{the}\:\mathrm{first}\:\mathrm{one}\:\mathrm{is}\:\mathrm{wrong},\:\mathrm{it}\:\mathrm{does}\:\mathrm{not}\:\mathrm{form}\:\mathrm{a} \\ $$$$\mathrm{series} \\ $$
Commented by mr W last updated on 06/Nov/23

$${it}'{s}\:{a}\:{fibonacci}\:{sequence}. \\ $$$$\mathrm{2}+\mathrm{7}=\mathrm{9} \\ $$$$\mathrm{7}+\mathrm{9}=\mathrm{16} \\ $$$$\mathrm{9}+\mathrm{16}=\mathrm{25} \\ $$$$... \\ $$$${a}_{{n}−\mathrm{2}} +{a}_{{n}−\mathrm{1}} ={a}_{{n}} \\ $$
Commented by hardmath last updated on 06/Nov/23
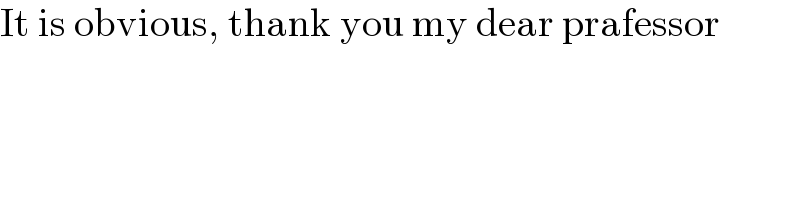
$$\mathrm{It}\:\mathrm{is}\:\mathrm{obvious},\:\mathrm{thank}\:\mathrm{you}\:\mathrm{my}\:\mathrm{dear}\:\mathrm{prafessor} \\ $$
