Question and Answers Forum

All Questions      Topic List

Algebra Questions

Previous in All Question      Next in All Question      

Previous in Algebra      Next in Algebra      

Question Number 198014 by hardmath last updated on 07/Oct/23

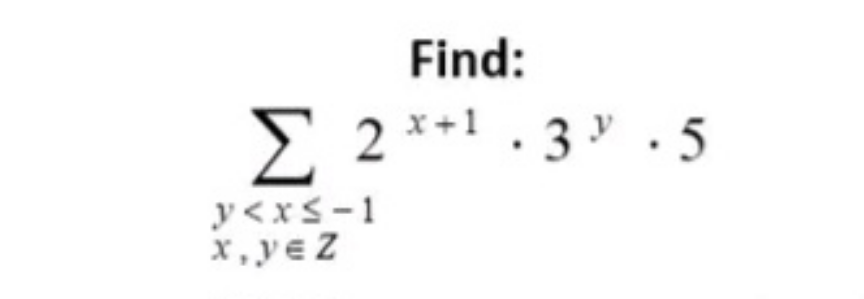
Answered by mahdipoor last updated on 07/Oct/23

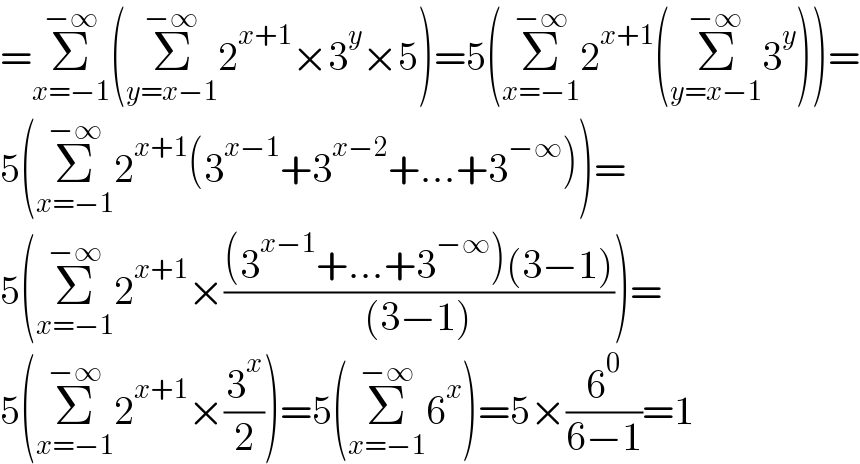
=Σ_(x=−1) ^(−∞) (Σ_(y=x−1) ^(−∞) 2^(x+1) ×3^y ×5)=5(Σ_(x=−1) ^(−∞) 2^(x+1) (Σ_(y=x−1) ^(−∞) 3^y ))= 5(Σ_(x=−1) ^(−∞) 2^(x+1) (3^(x−1) +3^(x−2) +...+3^(−∞) ))= 5(Σ_(x=−1) ^(−∞) 2^(x+1) ×(((3^(x−1) +...+3^(−∞) )(3−1))/((3−1))))= 5(Σ_(x=−1) ^(−∞) 2^(x+1) ×(3^x /2))=5(Σ_(x=−1) ^(−∞) 6^x )=5×(6^0 /(6−1))=1

$$=\underset{{x}=−\mathrm{1}} {\overset{−\infty} {\sum}}\left(\underset{{y}={x}−\mathrm{1}} {\overset{−\infty} {\sum}}\mathrm{2}^{{x}+\mathrm{1}} ×\mathrm{3}^{{y}} ×\mathrm{5}\right)=\mathrm{5}\left(\underset{{x}=−\mathrm{1}} {\overset{−\infty} {\sum}}\mathrm{2}^{{x}+\mathrm{1}} \left(\underset{{y}={x}−\mathrm{1}} {\overset{−\infty} {\sum}}\mathrm{3}^{{y}} \right)\right)= \\ $$$$\mathrm{5}\left(\underset{{x}=−\mathrm{1}} {\overset{−\infty} {\sum}}\mathrm{2}^{{x}+\mathrm{1}} \left(\mathrm{3}^{{x}−\mathrm{1}} +\mathrm{3}^{{x}−\mathrm{2}} +...+\mathrm{3}^{−\infty} \right)\right)= \\ $$$$\mathrm{5}\left(\underset{{x}=−\mathrm{1}} {\overset{−\infty} {\sum}}\mathrm{2}^{{x}+\mathrm{1}} ×\frac{\left(\mathrm{3}^{{x}−\mathrm{1}} +...+\mathrm{3}^{−\infty} \right)\left(\mathrm{3}−\mathrm{1}\right)}{\left(\mathrm{3}−\mathrm{1}\right)}\right)= \\ $$$$\mathrm{5}\left(\underset{{x}=−\mathrm{1}} {\overset{−\infty} {\sum}}\mathrm{2}^{{x}+\mathrm{1}} ×\frac{\mathrm{3}^{{x}} }{\mathrm{2}}\right)=\mathrm{5}\left(\underset{{x}=−\mathrm{1}} {\overset{−\infty} {\sum}}\mathrm{6}^{{x}} \right)=\mathrm{5}×\frac{\mathrm{6}^{\mathrm{0}} }{\mathrm{6}−\mathrm{1}}=\mathrm{1} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com