
Question Number 195681 by sonukgindia last updated on 07/Aug/23

Answered by Frix last updated on 07/Aug/23
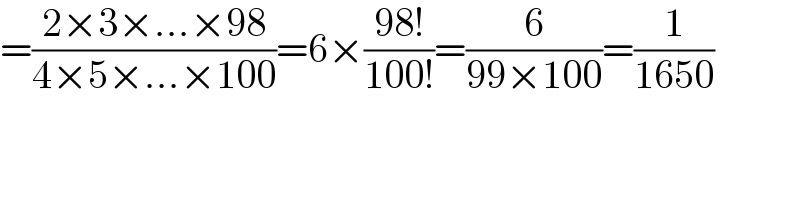
$$=\frac{\mathrm{2}×\mathrm{3}×...×\mathrm{98}}{\mathrm{4}×\mathrm{5}×...×\mathrm{100}}=\mathrm{6}×\frac{\mathrm{98}!}{\mathrm{100}!}=\frac{\mathrm{6}}{\mathrm{99}×\mathrm{100}}=\frac{\mathrm{1}}{\mathrm{1650}} \\ $$
Answered by MM42 last updated on 07/Aug/23
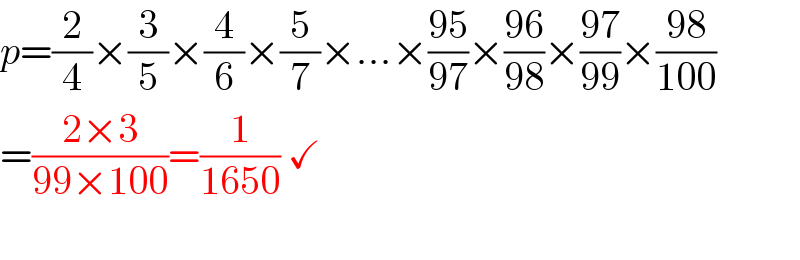
$${p}=\frac{\mathrm{2}}{\mathrm{4}}×\frac{\mathrm{3}}{\mathrm{5}}×\frac{\mathrm{4}}{\mathrm{6}}×\frac{\mathrm{5}}{\mathrm{7}}×...×\frac{\mathrm{95}}{\mathrm{97}}×\frac{\mathrm{96}}{\mathrm{98}}×\frac{\mathrm{97}}{\mathrm{99}}×\frac{\mathrm{98}}{\mathrm{100}} \\ $$$$=\frac{\mathrm{2}×\mathrm{3}}{\mathrm{99}×\mathrm{100}}=\frac{\mathrm{1}}{\mathrm{1650}}\:\checkmark \\ $$$$ \\ $$
