
Question Number 193949 by sonukgindia last updated on 23/Jun/23
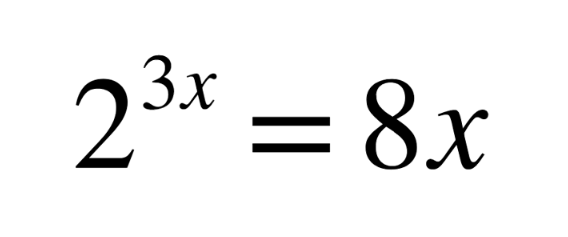
Answered by aleks041103 last updated on 23/Jun/23
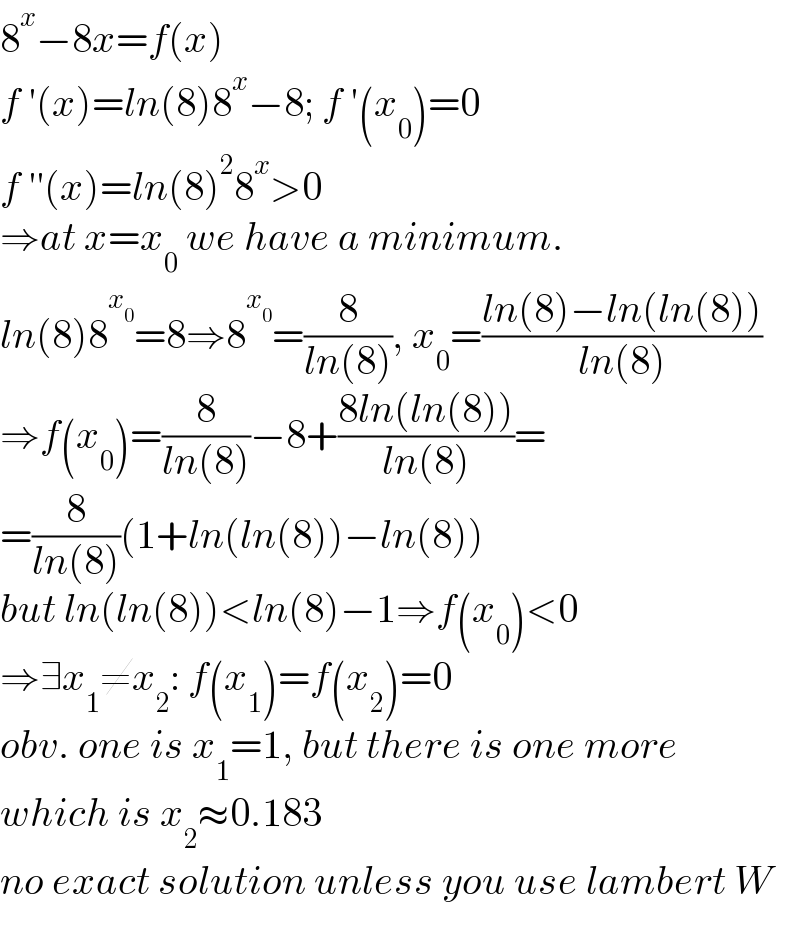
$$\mathrm{8}^{{x}} −\mathrm{8}{x}={f}\left({x}\right) \\ $$$${f}\:'\left({x}\right)={ln}\left(\mathrm{8}\right)\mathrm{8}^{{x}} −\mathrm{8};\:{f}\:'\left({x}_{\mathrm{0}} \right)=\mathrm{0} \\ $$$${f}\:''\left({x}\right)={ln}\left(\mathrm{8}\right)^{\mathrm{2}} \mathrm{8}^{{x}} >\mathrm{0} \\ $$$$\Rightarrow{at}\:{x}={x}_{\mathrm{0}} \:{we}\:{have}\:{a}\:{minimum}. \\ $$$${ln}\left(\mathrm{8}\right)\mathrm{8}^{{x}_{\mathrm{0}} } =\mathrm{8}\Rightarrow\mathrm{8}^{{x}_{\mathrm{0}} } =\frac{\mathrm{8}}{{ln}\left(\mathrm{8}\right)},\:{x}_{\mathrm{0}} =\frac{{ln}\left(\mathrm{8}\right)−{ln}\left({ln}\left(\mathrm{8}\right)\right)}{{ln}\left(\mathrm{8}\right)} \\ $$$$\Rightarrow{f}\left({x}_{\mathrm{0}} \right)=\frac{\mathrm{8}}{{ln}\left(\mathrm{8}\right)}−\mathrm{8}+\frac{\mathrm{8}{ln}\left({ln}\left(\mathrm{8}\right)\right)}{{ln}\left(\mathrm{8}\right)}= \\ $$$$=\frac{\mathrm{8}}{{ln}\left(\mathrm{8}\right)}\left(\mathrm{1}+{ln}\left({ln}\left(\mathrm{8}\right)\right)−{ln}\left(\mathrm{8}\right)\right) \\ $$$${but}\:{ln}\left({ln}\left(\mathrm{8}\right)\right)<{ln}\left(\mathrm{8}\right)−\mathrm{1}\Rightarrow{f}\left({x}_{\mathrm{0}} \right)<\mathrm{0} \\ $$$$\Rightarrow\exists{x}_{\mathrm{1}} \neq{x}_{\mathrm{2}} :\:{f}\left({x}_{\mathrm{1}} \right)={f}\left({x}_{\mathrm{2}} \right)=\mathrm{0} \\ $$$${obv}.\:{one}\:{is}\:{x}_{\mathrm{1}} =\mathrm{1},\:{but}\:{there}\:{is}\:{one}\:{more} \\ $$$${which}\:{is}\:{x}_{\mathrm{2}} \approx\mathrm{0}.\mathrm{183} \\ $$$${no}\:{exact}\:{solution}\:{unless}\:{you}\:{use}\:{lambert}\:{W} \\ $$
Answered by Subhi last updated on 24/Jun/23
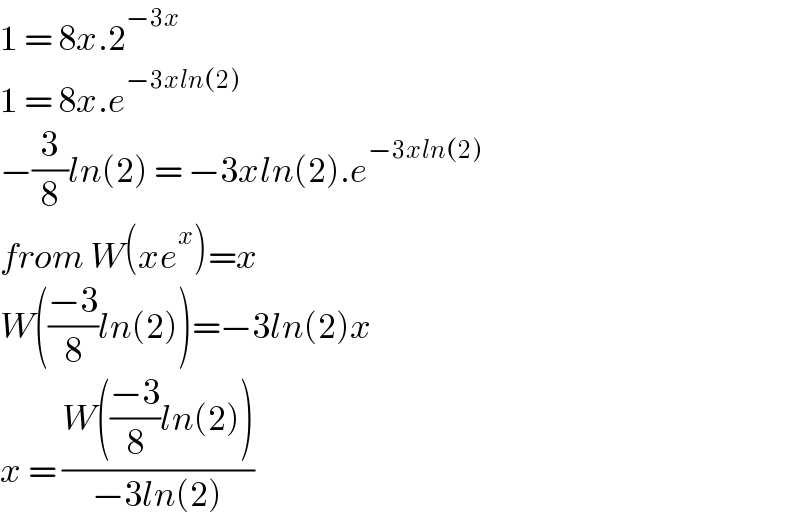
$$\mathrm{1}\:=\:\mathrm{8}{x}.\mathrm{2}^{−\mathrm{3}{x}} \:\: \\ $$$$\mathrm{1}\:=\:\mathrm{8}{x}.{e}^{−\mathrm{3}{xln}\left(\mathrm{2}\right)} \\ $$$$−\frac{\mathrm{3}}{\mathrm{8}}{ln}\left(\mathrm{2}\right)\:=\:−\mathrm{3}{xln}\left(\mathrm{2}\right).{e}^{−\mathrm{3}{xln}\left(\mathrm{2}\right)} \\ $$$${from}\:{W}\left({xe}^{{x}} \right)={x} \\ $$$${W}\left(\frac{−\mathrm{3}}{\mathrm{8}}{ln}\left(\mathrm{2}\right)\right)=−\mathrm{3}{ln}\left(\mathrm{2}\right){x} \\ $$$${x}\:=\:\frac{{W}\left(\frac{−\mathrm{3}}{\mathrm{8}}{ln}\left(\mathrm{2}\right)\right)}{−\mathrm{3}{ln}\left(\mathrm{2}\right)} \\ $$
Answered by Spillover last updated on 24/Jun/23

$${let}\:{y}=\mathrm{2}^{\mathrm{3}{x}} =\mathrm{8}{x}\:\:\:\:\:{y}=\mathrm{2}^{\mathrm{3}{x}\:\:} \:\:{y}=\mathrm{8}{x} \\ $$$${draw}\:{the}\:{graph}\:{then}\:{find}\:{point}\:{of}\: \\ $$$${intersection} \\ $$$$ \\ $$
