
Question Number 189135 by Shrinava last updated on 12/Mar/23
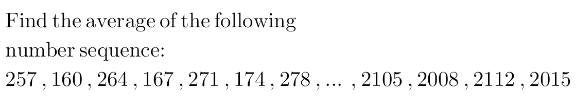
Answered by a.lgnaoui last updated on 12/Mar/23

$$\left(\mathrm{257}−\mathrm{160}\right)=\left(\mathrm{264}−\mathrm{167}\right)=\left(\mathrm{271}−\mathrm{174}\right)=......=\left(\mathrm{2112}−\mathrm{2015}\right) \\ $$$$=\mathrm{97}\:\:\Rightarrow{suite}\:{aritmetique}\: \\ $$$${de}\:{raison}:\mathrm{97}\:\:\:;{u}_{\mathrm{0}} \:=\mathrm{257} \\ $$$${u}_{{n}+\mathrm{1}} =\mathrm{257}\left(\mathrm{1}+\mathrm{97}{n}\:\:\right)\:\left({n}>=\mathrm{0}\right) \\ $$
Commented by Shrinava last updated on 13/Mar/23
$$\mathrm{thankyou}\:\mathrm{dearProfessor}\:\mathrm{cool} \\ $$
Answered by mr W last updated on 13/Mar/23

$${a}=\left\{\mathrm{257},\:\mathrm{264},\:\mathrm{271},\:...,\:\mathrm{2105},\:\mathrm{2112}\right\} \\ $$$$\underset{{i}=\mathrm{1}} {\overset{\mathrm{266}} {\sum}}{a}_{{i}} =\frac{\mathrm{266}×\left(\mathrm{257}+\mathrm{2112}\right)}{\mathrm{2}}=\mathrm{266}×\mathrm{1184}.\mathrm{5} \\ $$$${b}=\left\{\mathrm{160},\:\mathrm{167},\:\mathrm{174},\:...,\:\mathrm{2008},\:\mathrm{2015}\right\} \\ $$$$\underset{{i}=\mathrm{1}} {\overset{\mathrm{266}} {\sum}}{b}_{{i}} =\frac{\mathrm{266}×\left(\mathrm{160}+\mathrm{2015}\right)}{\mathrm{2}}=\mathrm{266}×\mathrm{1087}.\mathrm{5} \\ $$$${average}\:=\frac{\Sigma}{{n}}=\frac{\Sigma{a}+\Sigma{b}}{\mathrm{2}×\mathrm{266}} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\frac{\mathrm{1184}.\mathrm{5}+\mathrm{1087}.\mathrm{5}}{\mathrm{2}}=\mathrm{1086}\:\checkmark \\ $$
Commented by Shrinava last updated on 15/Mar/23
$$\mathrm{cool}\:\mathrm{dear}\:\mathrm{professor},\mathrm{thank}\:\mathrm{you} \\ $$
