Question and Answers Forum

All Questions      Topic List

Others Questions

Previous in All Question      Next in All Question      

Previous in Others      Next in Others      

Question Number 188203 by moh777 last updated on 26/Feb/23

Answered by mr W last updated on 26/Feb/23

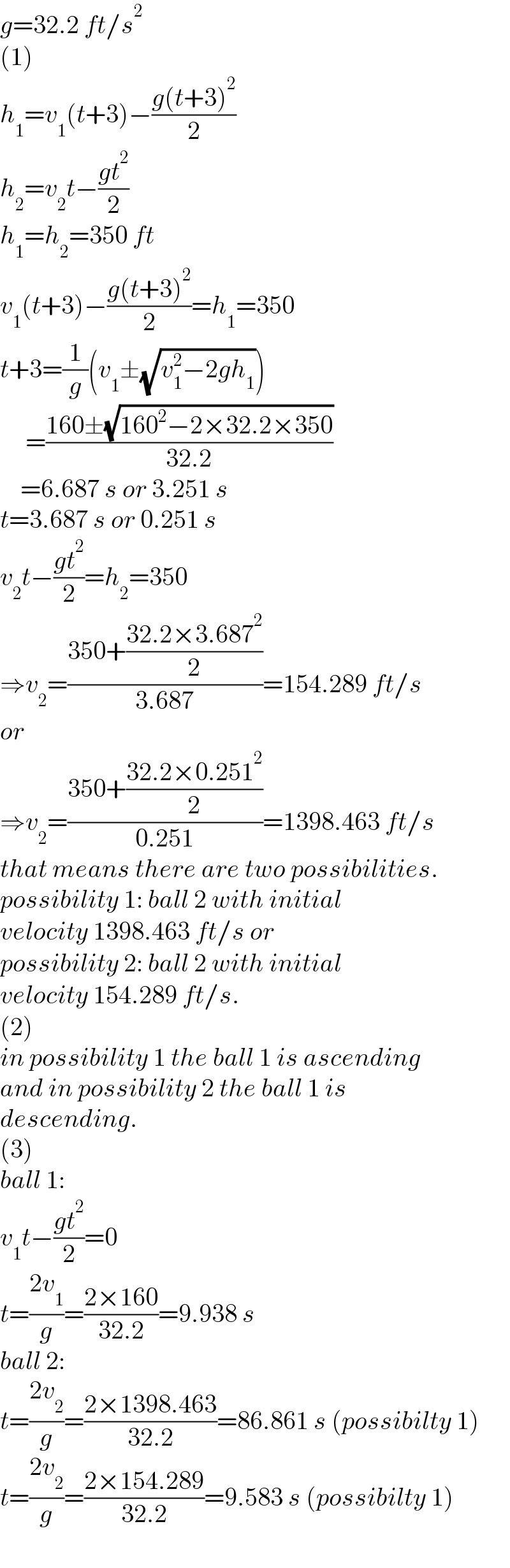
g=32.2 ft/s^2 (1) h_1 =v_1 (t+3)−((g(t+3)^2 )/2) h_2 =v_2 t−((gt^2 )/2) h_1 =h_2 =350 ft v_1 (t+3)−((g(t+3)^2 )/2)=h_1 =350 t+3=(1/g)(v_1 ±(√(v_1 ^2 −2gh_1 ))) =((160±(√(160^2 −2×32.2×350)))/(32.2)) =6.687 s or 3.251 s t=3.687 s or 0.251 s v_2 t−((gt^2 )/2)=h_2 =350 ⇒v_2 =((350+((32.2×3.687^2 )/2))/(3.687))=154.289 ft/s or ⇒v_2 =((350+((32.2×0.251^2 )/2))/(0.251))=1398.463 ft/s that means there are two possibilities. possibility 1: ball 2 with initial velocity 1398.463 ft/s or possibility 2: ball 2 with initial velocity 154.289 ft/s. (2) in possibility 1 the ball 1 is ascending and in possibility 2 the ball 1 is descending. (3) ball 1: v_1 t−((gt^2 )/2)=0 t=((2v_1 )/g)=((2×160)/(32.2))=9.938 s ball 2: t=((2v_2 )/g)=((2×1398.463)/(32.2))=86.861 s (possibilty 1) t=((2v_2 )/g)=((2×154.289)/(32.2))=9.583 s (possibilty 1)

$${g}=\mathrm{32}.\mathrm{2}\:{ft}/{s}^{\mathrm{2}} \\ $$$$\left(\mathrm{1}\right) \\ $$$${h}_{\mathrm{1}} ={v}_{\mathrm{1}} \left({t}+\mathrm{3}\right)−\frac{{g}\left({t}+\mathrm{3}\right)^{\mathrm{2}} }{\mathrm{2}} \\ $$$${h}_{\mathrm{2}} ={v}_{\mathrm{2}} {t}−\frac{{gt}^{\mathrm{2}} }{\mathrm{2}} \\ $$$${h}_{\mathrm{1}} ={h}_{\mathrm{2}} =\mathrm{350}\:{ft} \\ $$$${v}_{\mathrm{1}} \left({t}+\mathrm{3}\right)−\frac{{g}\left({t}+\mathrm{3}\right)^{\mathrm{2}} }{\mathrm{2}}={h}_{\mathrm{1}} =\mathrm{350} \\ $$$${t}+\mathrm{3}=\frac{\mathrm{1}}{{g}}\left({v}_{\mathrm{1}} \pm\sqrt{{v}_{\mathrm{1}} ^{\mathrm{2}} −\mathrm{2}{gh}_{\mathrm{1}} }\right) \\ $$$$\:\:\:\:\:=\frac{\mathrm{160}\pm\sqrt{\mathrm{160}^{\mathrm{2}} −\mathrm{2}×\mathrm{32}.\mathrm{2}×\mathrm{350}}}{\mathrm{32}.\mathrm{2}} \\ $$$$\:\:\:\:=\mathrm{6}.\mathrm{687}\:{s}\:{or}\:\mathrm{3}.\mathrm{251}\:{s} \\ $$$${t}=\mathrm{3}.\mathrm{687}\:{s}\:{or}\:\mathrm{0}.\mathrm{251}\:{s} \\ $$$${v}_{\mathrm{2}} {t}−\frac{{gt}^{\mathrm{2}} }{\mathrm{2}}={h}_{\mathrm{2}} =\mathrm{350} \\ $$$$\Rightarrow{v}_{\mathrm{2}} =\frac{\mathrm{350}+\frac{\mathrm{32}.\mathrm{2}×\mathrm{3}.\mathrm{687}^{\mathrm{2}} }{\mathrm{2}}}{\mathrm{3}.\mathrm{687}}=\mathrm{154}.\mathrm{289}\:{ft}/{s} \\ $$$${or} \\ $$$$\Rightarrow{v}_{\mathrm{2}} =\frac{\mathrm{350}+\frac{\mathrm{32}.\mathrm{2}×\mathrm{0}.\mathrm{251}^{\mathrm{2}} }{\mathrm{2}}}{\mathrm{0}.\mathrm{251}}=\mathrm{1398}.\mathrm{463}\:{ft}/{s} \\ $$$${that}\:{means}\:{there}\:{are}\:{two}\:{possibilities}. \\ $$$${possibility}\:\mathrm{1}:\:{ball}\:\mathrm{2}\:{with}\:{initial} \\ $$$${velocity}\:\mathrm{1398}.\mathrm{463}\:{ft}/{s}\:{or} \\ $$$${possibility}\:\mathrm{2}:\:{ball}\:\mathrm{2}\:{with}\:{initial} \\ $$$${velocity}\:\mathrm{154}.\mathrm{289}\:{ft}/{s}. \\ $$$$\left(\mathrm{2}\right) \\ $$$${in}\:{possibility}\:\mathrm{1}\:{the}\:{ball}\:\mathrm{1}\:{is}\:{ascending} \\ $$$${and}\:{in}\:{possibility}\:\mathrm{2}\:{the}\:{ball}\:\mathrm{1}\:{is}\: \\ $$$${descending}. \\ $$$$\left(\mathrm{3}\right) \\ $$$${ball}\:\mathrm{1}: \\ $$$${v}_{\mathrm{1}} {t}−\frac{{gt}^{\mathrm{2}} }{\mathrm{2}}=\mathrm{0} \\ $$$${t}=\frac{\mathrm{2}{v}_{\mathrm{1}} }{{g}}=\frac{\mathrm{2}×\mathrm{160}}{\mathrm{32}.\mathrm{2}}=\mathrm{9}.\mathrm{938}\:{s} \\ $$$${ball}\:\mathrm{2}: \\ $$$${t}=\frac{\mathrm{2}{v}_{\mathrm{2}} }{{g}}=\frac{\mathrm{2}×\mathrm{1398}.\mathrm{463}}{\mathrm{32}.\mathrm{2}}=\mathrm{86}.\mathrm{861}\:{s}\:\left({possibilty}\:\mathrm{1}\right) \\ $$$${t}=\frac{\mathrm{2}{v}_{\mathrm{2}} }{{g}}=\frac{\mathrm{2}×\mathrm{154}.\mathrm{289}}{\mathrm{32}.\mathrm{2}}=\mathrm{9}.\mathrm{583}\:{s}\:\left({possibilty}\:\mathrm{1}\right) \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com