
Question Number 188177 by cortano12 last updated on 26/Feb/23

Commented by cortano12 last updated on 26/Feb/23
![[ BCDH ] = 6m^2 [ ABI ] = 4m^2 [ DGE ] =2m^2 Find [ AIF ]](Q188178.png)
$$\:\left[\:\mathrm{BCDH}\:\right]\:=\:\mathrm{6m}^{\mathrm{2}} \\ $$$$\:\left[\:\mathrm{ABI}\:\right]\:=\:\mathrm{4m}^{\mathrm{2}} \\ $$$$\:\left[\:\mathrm{DGE}\:\right]\:=\mathrm{2m}^{\mathrm{2}} \\ $$$$\:\mathrm{Find}\:\left[\:\mathrm{AIF}\:\right] \\ $$
Commented by cortano12 last updated on 26/Feb/23
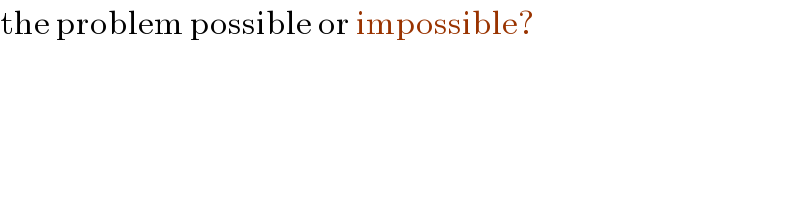
$$\mathrm{the}\:\mathrm{problem}\:\mathrm{possible}\:\mathrm{or}\:\mathrm{impossible}? \\ $$
Answered by mr W last updated on 26/Feb/23
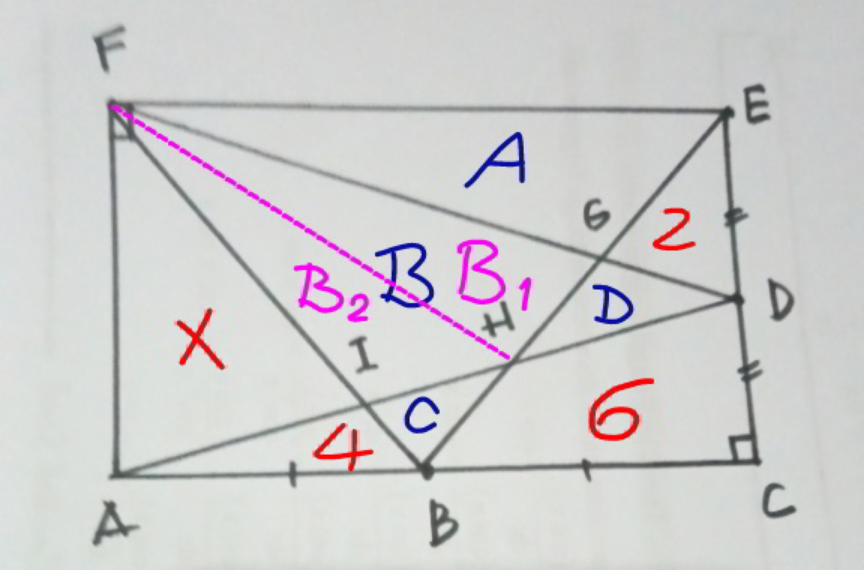
Commented by cortano12 last updated on 26/Feb/23
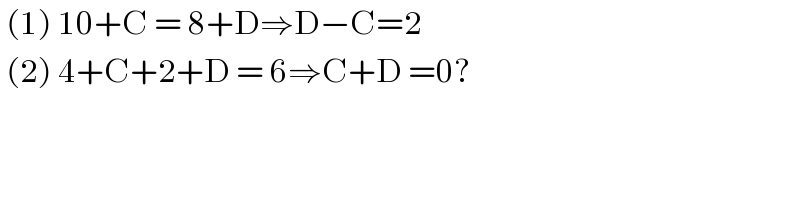
$$\:\left(\mathrm{1}\right)\:\mathrm{10}+\mathrm{C}\:=\:\mathrm{8}+\mathrm{D}\Rightarrow\mathrm{D}−\mathrm{C}=\mathrm{2} \\ $$$$\:\left(\mathrm{2}\right)\:\mathrm{4}+\mathrm{C}+\mathrm{2}+\mathrm{D}\:=\:\mathrm{6}\Rightarrow\mathrm{C}+\mathrm{D}\:=\mathrm{0}? \\ $$
Commented by mr W last updated on 26/Feb/23
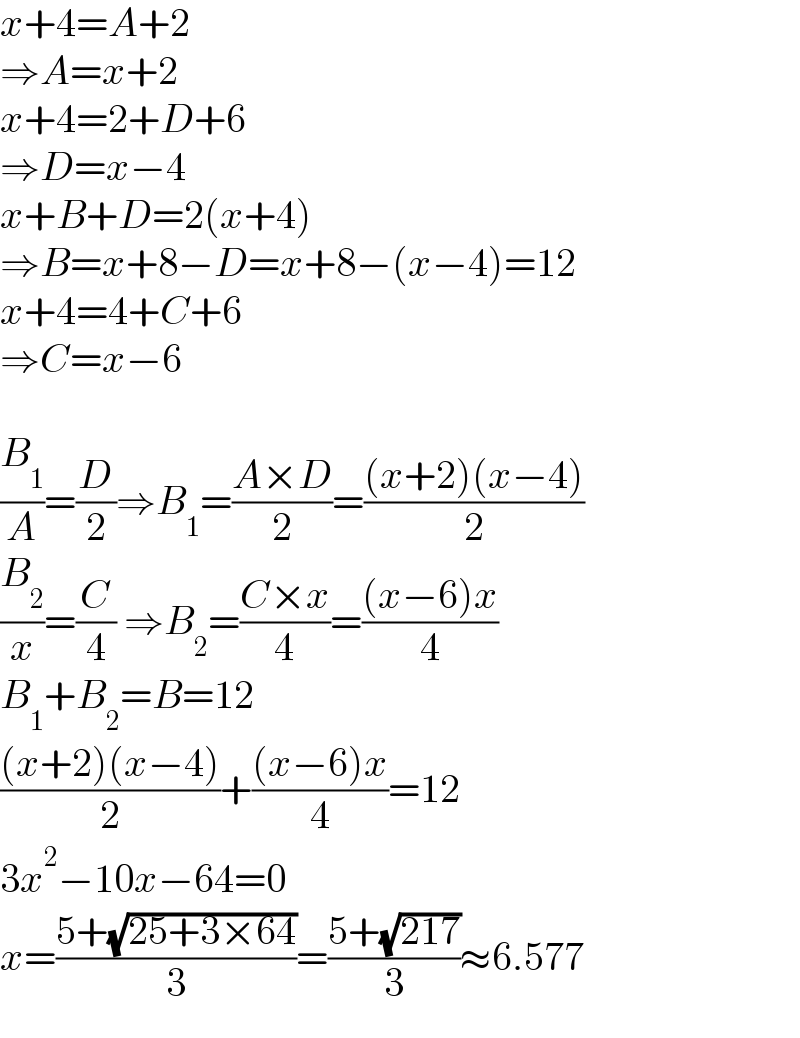
$${x}+\mathrm{4}={A}+\mathrm{2} \\ $$$$\Rightarrow{A}={x}+\mathrm{2} \\ $$$${x}+\mathrm{4}=\mathrm{2}+{D}+\mathrm{6} \\ $$$$\Rightarrow{D}={x}−\mathrm{4} \\ $$$${x}+{B}+{D}=\mathrm{2}\left({x}+\mathrm{4}\right) \\ $$$$\Rightarrow{B}={x}+\mathrm{8}−{D}={x}+\mathrm{8}−\left({x}−\mathrm{4}\right)=\mathrm{12} \\ $$$${x}+\mathrm{4}=\mathrm{4}+{C}+\mathrm{6} \\ $$$$\Rightarrow{C}={x}−\mathrm{6} \\ $$$$ \\ $$$$\frac{{B}_{\mathrm{1}} }{{A}}=\frac{{D}}{\mathrm{2}}\Rightarrow{B}_{\mathrm{1}} =\frac{{A}×{D}}{\mathrm{2}}=\frac{\left({x}+\mathrm{2}\right)\left({x}−\mathrm{4}\right)}{\mathrm{2}} \\ $$$$\frac{{B}_{\mathrm{2}} }{{x}}=\frac{{C}}{\mathrm{4}}\:\Rightarrow{B}_{\mathrm{2}} =\frac{{C}×{x}}{\mathrm{4}}=\frac{\left({x}−\mathrm{6}\right){x}}{\mathrm{4}} \\ $$$${B}_{\mathrm{1}} +{B}_{\mathrm{2}} ={B}=\mathrm{12} \\ $$$$\frac{\left({x}+\mathrm{2}\right)\left({x}−\mathrm{4}\right)}{\mathrm{2}}+\frac{\left({x}−\mathrm{6}\right){x}}{\mathrm{4}}=\mathrm{12} \\ $$$$\mathrm{3}{x}^{\mathrm{2}} −\mathrm{10}{x}−\mathrm{64}=\mathrm{0} \\ $$$${x}=\frac{\mathrm{5}+\sqrt{\mathrm{25}+\mathrm{3}×\mathrm{64}}}{\mathrm{3}}=\frac{\mathrm{5}+\sqrt{\mathrm{217}}}{\mathrm{3}}\approx\mathrm{6}.\mathrm{577} \\ $$
Commented by cortano12 last updated on 26/Feb/23
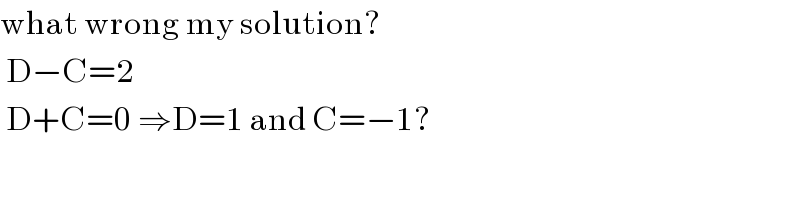
$$\mathrm{what}\:\mathrm{wrong}\:\mathrm{my}\:\mathrm{solution}? \\ $$$$\:\mathrm{D}−\mathrm{C}=\mathrm{2} \\ $$$$\:\mathrm{D}+\mathrm{C}=\mathrm{0}\:\Rightarrow\mathrm{D}=\mathrm{1}\:\mathrm{and}\:\mathrm{C}=−\mathrm{1}? \\ $$
Commented by cortano12 last updated on 26/Feb/23

$$\mathrm{the}\:\mathrm{answer}\:\mathrm{x}=\mathrm{7} \\ $$
Commented by cortano12 last updated on 26/Feb/23
![Let [ BCH ] = u = 4+C let [ CDH ] = v= 2+D then u+v = 6 ⇒D+C =? i think impossible](Q188188.png)
$$\mathrm{Let}\:\left[\:\mathrm{BCH}\:\right]\:=\:\mathrm{u}\:=\:\mathrm{4}+\mathrm{C} \\ $$$$\:\mathrm{let}\:\left[\:\mathrm{CDH}\:\right]\:=\:\mathrm{v}=\:\mathrm{2}+\mathrm{D} \\ $$$$\:\mathrm{then}\:\mathrm{u}+\mathrm{v}\:=\:\mathrm{6}\:\Rightarrow\mathrm{D}+\mathrm{C}\:=? \\ $$$$\:\mathrm{i}\:\mathrm{think}\:\mathrm{impossible} \\ $$
Commented by mr W last updated on 26/Feb/23
![we should select [ABI]+[DGE]≠[BCDH]](Q188189.png)
$${we}\:{should}\:{select}\:\left[{ABI}\right]+\left[{DGE}\right]\neq\left[{BCDH}\right] \\ $$
Commented by cortano12 last updated on 26/Feb/23

$$\mathrm{why}? \\ $$
Commented by cortano12 last updated on 26/Feb/23
![[ ABH ] = [ BCH ] , since AB=BC [ CDH ] = [ DHE ], since CD=DE so [ BCDH ] = [ BCH ]+ [ CHD ] 6 = 4+C + 2+D](Q188191.png)
$$\:\left[\:\mathrm{ABH}\:\right]\:=\:\left[\:\mathrm{BCH}\:\right]\:,\:\mathrm{since}\:\mathrm{AB}=\mathrm{BC} \\ $$$$\:\left[\:\mathrm{CDH}\:\right]\:=\:\left[\:\mathrm{DHE}\:\right],\:\mathrm{since}\:\mathrm{CD}=\mathrm{DE} \\ $$$$\:\mathrm{so}\:\left[\:\mathrm{BCDH}\:\right]\:=\:\left[\:\mathrm{BCH}\:\right]+\:\left[\:\mathrm{CHD}\:\right] \\ $$$$\:\mathrm{6}\:=\:\mathrm{4}+\mathrm{C}\:+\:\mathrm{2}+\mathrm{D} \\ $$
Commented by mr W last updated on 26/Feb/23

Commented by mr W last updated on 26/Feb/23

$${eqn}.\:{of}\:{AD}: \\ $$$${y}=\frac{{xb}}{\mathrm{2}{a}} \\ $$$${eqn}.\:{of}\:{BF}: \\ $$$${y}=−\frac{\mathrm{2}{b}}{{a}}\left({x}−\frac{{a}}{\mathrm{2}}\right) \\ $$$${eqn}.\:{of}\:{BE}: \\ $$$${y}=\frac{\mathrm{2}{b}}{{a}}\left({x}−\frac{{a}}{\mathrm{2}}\right) \\ $$$${eqn}.\:{of}\:{DF}: \\ $$$${y}={b}−\frac{{xb}}{\mathrm{2}{a}} \\ $$$${intersection}\:{point}\:{I}: \\ $$$${y}_{{I}} =\frac{{x}_{{I}} {b}}{\mathrm{2}{a}}=−\frac{\mathrm{2}{b}}{{a}}\left({x}_{{I}} −\frac{{a}}{\mathrm{2}}\right) \\ $$$$\Rightarrow{x}_{{I}} =\frac{\mathrm{2}{a}}{\mathrm{5}} \\ $$$$\Rightarrow{y}_{{I}} =\frac{{b}}{\mathrm{5}} \\ $$$${area}\:{X}=\frac{{a}}{\mathrm{4}}×\frac{{b}}{\mathrm{5}}=\frac{{ab}}{\mathrm{20}} \\ $$$${intersection}\:{point}\:{G}: \\ $$$${y}_{{G}} =\frac{\mathrm{2}{b}}{{a}}\left({x}_{{G}} −\frac{{a}}{\mathrm{2}}\right)={b}−\frac{{x}_{{G}} {b}}{\mathrm{2}{a}} \\ $$$$\Rightarrow{x}_{{G}} =\frac{\mathrm{4}{a}}{\mathrm{5}} \\ $$$$\Rightarrow{y}_{{G}} =\frac{\mathrm{3}{b}}{\mathrm{5}} \\ $$$${area}\:{Y}=\frac{{b}}{\mathrm{4}}×\left({a}−\frac{\mathrm{4}{a}}{\mathrm{5}}\right)=\frac{{ab}}{\mathrm{20}}={X} \\ $$$${intersection}\:{point}\:{H}: \\ $$$${y}_{{H}} =\frac{{x}_{{H}} {b}}{\mathrm{2}{a}}=\frac{\mathrm{2}{b}}{{a}}\left({x}_{{H}} −\frac{{a}}{\mathrm{2}}\right) \\ $$$$\Rightarrow{x}_{{H}} =\frac{\mathrm{2}{a}}{\mathrm{3}} \\ $$$$\Rightarrow{y}_{{H}} =\frac{{b}}{\mathrm{3}} \\ $$$${area}\:{Z}=\frac{{a}}{\mathrm{4}}×\frac{{b}}{\mathrm{3}}+\frac{{b}}{\mathrm{4}}×\left({a}−\frac{\mathrm{2}{a}}{\mathrm{3}}\right)=\frac{{ab}}{\mathrm{6}}>{X}+{Y} \\ $$$${we}\:{see}: \\ $$$${area}\:{X}={area}\:{Y} \\ $$$${area}\:{Z}\neq{area}\:{X}+{area}\:{Y} \\ $$
Commented by mr W last updated on 26/Feb/23
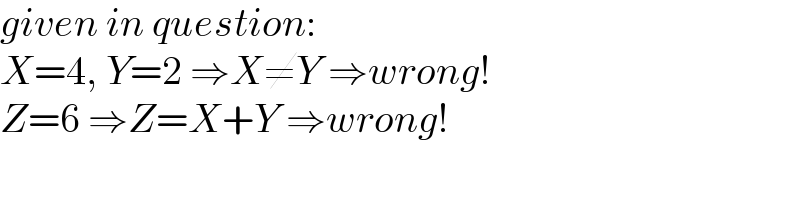
$${given}\:{in}\:{question}: \\ $$$${X}=\mathrm{4},\:{Y}=\mathrm{2}\:\Rightarrow{X}\neq{Y}\:\Rightarrow{wrong}! \\ $$$${Z}=\mathrm{6}\:\Rightarrow{Z}={X}+{Y}\:\Rightarrow{wrong}! \\ $$
Commented by mr W last updated on 26/Feb/23
$${following}\:{is}\:{e}.{g}.\:{solvable}: \\ $$
Commented by mr W last updated on 26/Feb/23
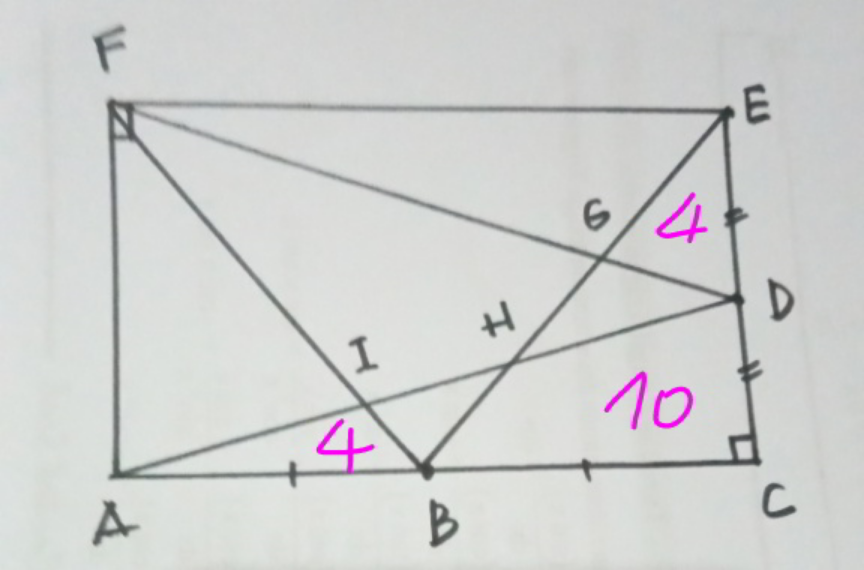
Answered by horsebrand11 last updated on 26/Feb/23
![it should be [ BCHD ]=10 ⇒4+C+2+D=10; C+D=4 ⇒14+C=12+D⇒D−C=2 then { ((D=3)),((C=1)) :} , it clear [ AFI ]= 11](Q188238.png)
$${it}\:{should}\:{be}\:\left[\:{BCHD}\:\right]=\mathrm{10} \\ $$$$\Rightarrow\mathrm{4}+{C}+\mathrm{2}+{D}=\mathrm{10};\:{C}+{D}=\mathrm{4} \\ $$$$\Rightarrow\mathrm{14}+{C}=\mathrm{12}+{D}\Rightarrow{D}−{C}=\mathrm{2} \\ $$$${then}\:\begin{cases}{{D}=\mathrm{3}}\\{{C}=\mathrm{1}}\end{cases}\:,\:{it}\:{clear}\:\left[\:{AFI}\:\right]=\:\mathrm{11} \\ $$
