Question and Answers Forum

All Questions      Topic List

Geometry Questions

Previous in All Question      Next in All Question      

Previous in Geometry      Next in Geometry      

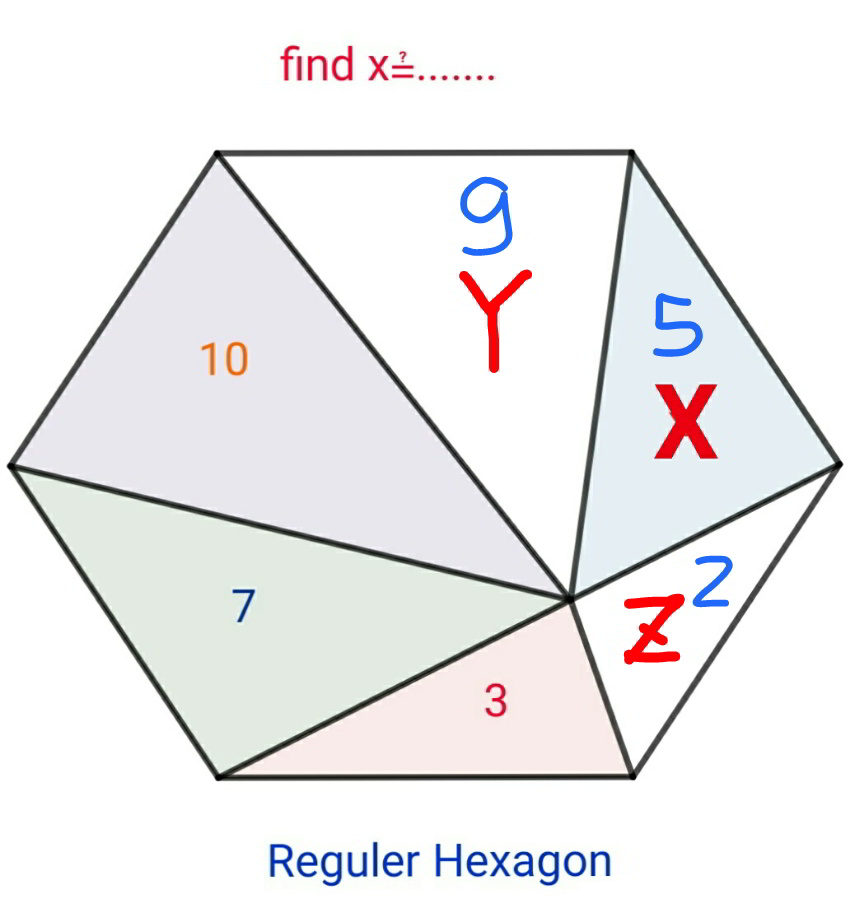
Question Number 187774 by normans last updated on 21/Feb/23

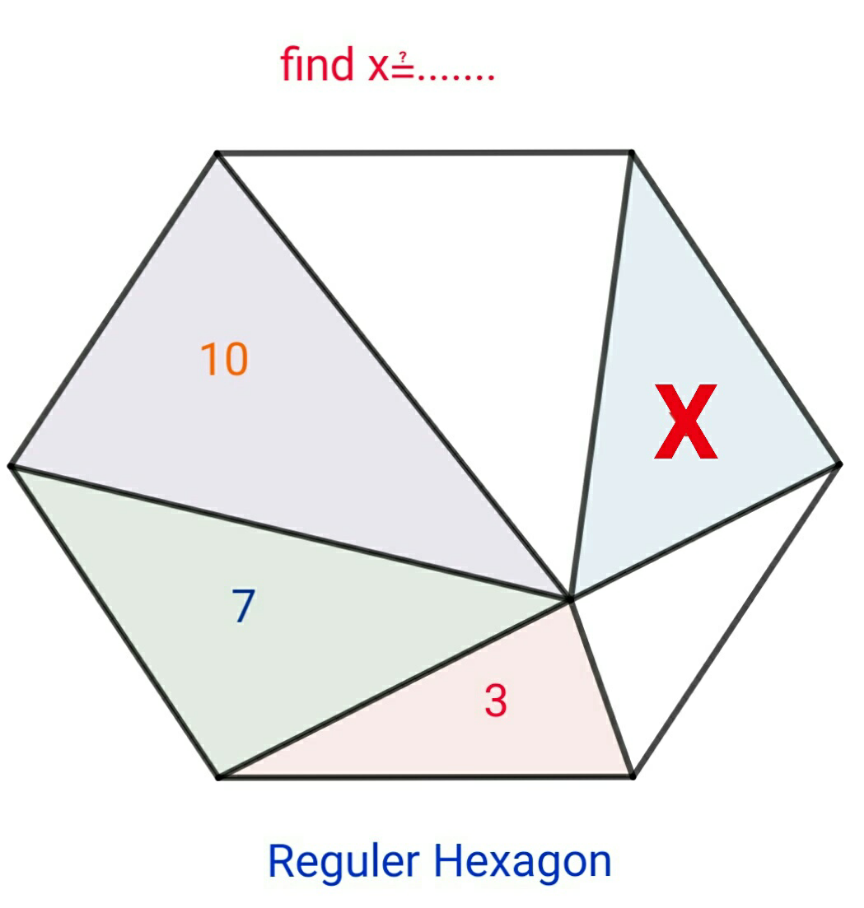
Answered by mr W last updated on 21/Feb/23

Commented by mr W last updated on 21/Feb/23

Method I a=side length of regular hexagon let OP=d sin α=(h_1 /d) sin β=(h_3 /d) α+β=(π/3) cos (α+β)=cos (π/3)=(1/2) ((√((d^2 −h_1 ^2 )(d^2 −h_3 ^2 )))/d^2 )−((h_1 h_3 )/d^2 )=(1/2) (d^2 −h_1 ^2 )(d^2 −h_3 ^2 )=((d^2 /2)+h_1 h_3 )^2 ⇒3d^2 =4(h_1 ^2 +h_3 ^2 +h_1 h_3 ) cos (α−(π/6))=((h_2 +(((√3)a)/2))/d) (√3) cos α+sin α=((2h_2 +(√3)a)/d) ((√(3(d^2 −h_1 ^2 )))/d)+(h_1 /d)=((2h_2 +(√3)a)/d) (√(3(d^2 −h_1 ^2 )))+h_1 =2h_2 +(√3)a (√(4(h_1 ^2 +h_3 ^2 +h_1 h_3 )−3h_1 ^2 ))+h_1 =2h_2 +(√3)a h_1 +2h_3 +h_1 =2h_2 +(√3)a ⇒(((√3)a)/2)=h_1 +h_3 −h_2 similarly ⇒(((√3)a)/2)=h_4 +h_2 −h_3 ⇒h_4 +h_2 −h_3 =h_1 +h_3 −h_2 ⇒h_4 =h_1 +2(h_3 −h_2 ) ⇒h=h_1 +h_4 =2(h_1 +h_3 −h_2 ) ⇒X+A_2 =2(A_1 +A_3 −A_2 ) ⇒X+7=2(10+3−7)=12 ⇒X=5

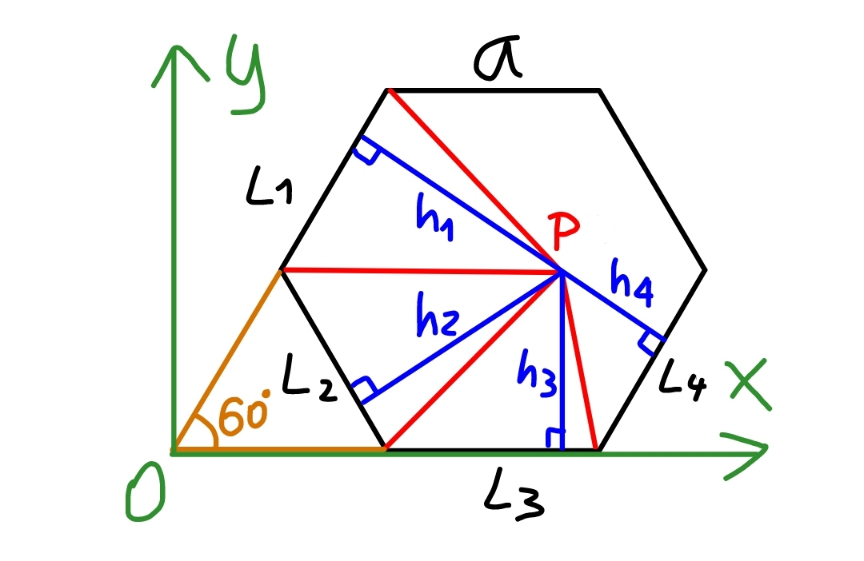
$$\boldsymbol{{Method}}\:\boldsymbol{{I}} \\ $$$${a}={side}\:{length}\:{of}\:{regular}\:{hexagon} \\ $$$${let}\:{OP}={d} \\ $$$$\mathrm{sin}\:\alpha=\frac{{h}_{\mathrm{1}} }{{d}} \\ $$$$\mathrm{sin}\:\beta=\frac{{h}_{\mathrm{3}} }{{d}} \\ $$$$\alpha+\beta=\frac{\pi}{\mathrm{3}} \\ $$$$\mathrm{cos}\:\left(\alpha+\beta\right)=\mathrm{cos}\:\frac{\pi}{\mathrm{3}}=\frac{\mathrm{1}}{\mathrm{2}} \\ $$$$\frac{\sqrt{\left({d}^{\mathrm{2}} −{h}_{\mathrm{1}} ^{\mathrm{2}} \right)\left({d}^{\mathrm{2}} −{h}_{\mathrm{3}} ^{\mathrm{2}} \right)}}{{d}^{\mathrm{2}} }−\frac{{h}_{\mathrm{1}} {h}_{\mathrm{3}} }{{d}^{\mathrm{2}} }=\frac{\mathrm{1}}{\mathrm{2}} \\ $$$$\left({d}^{\mathrm{2}} −{h}_{\mathrm{1}} ^{\mathrm{2}} \right)\left({d}^{\mathrm{2}} −{h}_{\mathrm{3}} ^{\mathrm{2}} \right)=\left(\frac{{d}^{\mathrm{2}} }{\mathrm{2}}+{h}_{\mathrm{1}} {h}_{\mathrm{3}} \right)^{\mathrm{2}} \\ $$$$\Rightarrow\mathrm{3}{d}^{\mathrm{2}} =\mathrm{4}\left({h}_{\mathrm{1}} ^{\mathrm{2}} +{h}_{\mathrm{3}} ^{\mathrm{2}} +{h}_{\mathrm{1}} {h}_{\mathrm{3}} \right) \\ $$$$\mathrm{cos}\:\left(\alpha−\frac{\pi}{\mathrm{6}}\right)=\frac{{h}_{\mathrm{2}} +\frac{\sqrt{\mathrm{3}}{a}}{\mathrm{2}}}{{d}} \\ $$$$\sqrt{\mathrm{3}}\:\mathrm{cos}\:\alpha+\mathrm{sin}\:\alpha=\frac{\mathrm{2}{h}_{\mathrm{2}} +\sqrt{\mathrm{3}}{a}}{{d}} \\ $$$$\frac{\sqrt{\mathrm{3}\left({d}^{\mathrm{2}} −{h}_{\mathrm{1}} ^{\mathrm{2}} \right)}}{{d}}+\frac{{h}_{\mathrm{1}} }{{d}}=\frac{\mathrm{2}{h}_{\mathrm{2}} +\sqrt{\mathrm{3}}{a}}{{d}} \\ $$$$\sqrt{\mathrm{3}\left({d}^{\mathrm{2}} −{h}_{\mathrm{1}} ^{\mathrm{2}} \right)}+{h}_{\mathrm{1}} =\mathrm{2}{h}_{\mathrm{2}} +\sqrt{\mathrm{3}}{a} \\ $$$$\sqrt{\mathrm{4}\left({h}_{\mathrm{1}} ^{\mathrm{2}} +{h}_{\mathrm{3}} ^{\mathrm{2}} +{h}_{\mathrm{1}} {h}_{\mathrm{3}} \right)−\mathrm{3}{h}_{\mathrm{1}} ^{\mathrm{2}} }+{h}_{\mathrm{1}} =\mathrm{2}{h}_{\mathrm{2}} +\sqrt{\mathrm{3}}{a} \\ $$$${h}_{\mathrm{1}} +\mathrm{2}{h}_{\mathrm{3}} +{h}_{\mathrm{1}} =\mathrm{2}{h}_{\mathrm{2}} +\sqrt{\mathrm{3}}{a} \\ $$$$\Rightarrow\frac{\sqrt{\mathrm{3}}{a}}{\mathrm{2}}={h}_{\mathrm{1}} +{h}_{\mathrm{3}} −{h}_{\mathrm{2}} \\ $$$${similarly} \\ $$$$\Rightarrow\frac{\sqrt{\mathrm{3}}{a}}{\mathrm{2}}={h}_{\mathrm{4}} +{h}_{\mathrm{2}} −{h}_{\mathrm{3}} \\ $$$$\Rightarrow{h}_{\mathrm{4}} +{h}_{\mathrm{2}} −{h}_{\mathrm{3}} ={h}_{\mathrm{1}} +{h}_{\mathrm{3}} −{h}_{\mathrm{2}} \\ $$$$\Rightarrow{h}_{\mathrm{4}} ={h}_{\mathrm{1}} +\mathrm{2}\left({h}_{\mathrm{3}} −{h}_{\mathrm{2}} \right) \\ $$$$\Rightarrow{h}={h}_{\mathrm{1}} +{h}_{\mathrm{4}} =\mathrm{2}\left({h}_{\mathrm{1}} +{h}_{\mathrm{3}} −{h}_{\mathrm{2}} \right) \\ $$$$\Rightarrow{X}+{A}_{\mathrm{2}} =\mathrm{2}\left({A}_{\mathrm{1}} +{A}_{\mathrm{3}} −{A}_{\mathrm{2}} \right) \\ $$$$\Rightarrow{X}+\mathrm{7}=\mathrm{2}\left(\mathrm{10}+\mathrm{3}−\mathrm{7}\right)=\mathrm{12} \\ $$$$\Rightarrow{X}=\mathrm{5} \\ $$

Answered by mr W last updated on 21/Feb/23

Commented by mr W last updated on 21/Feb/23

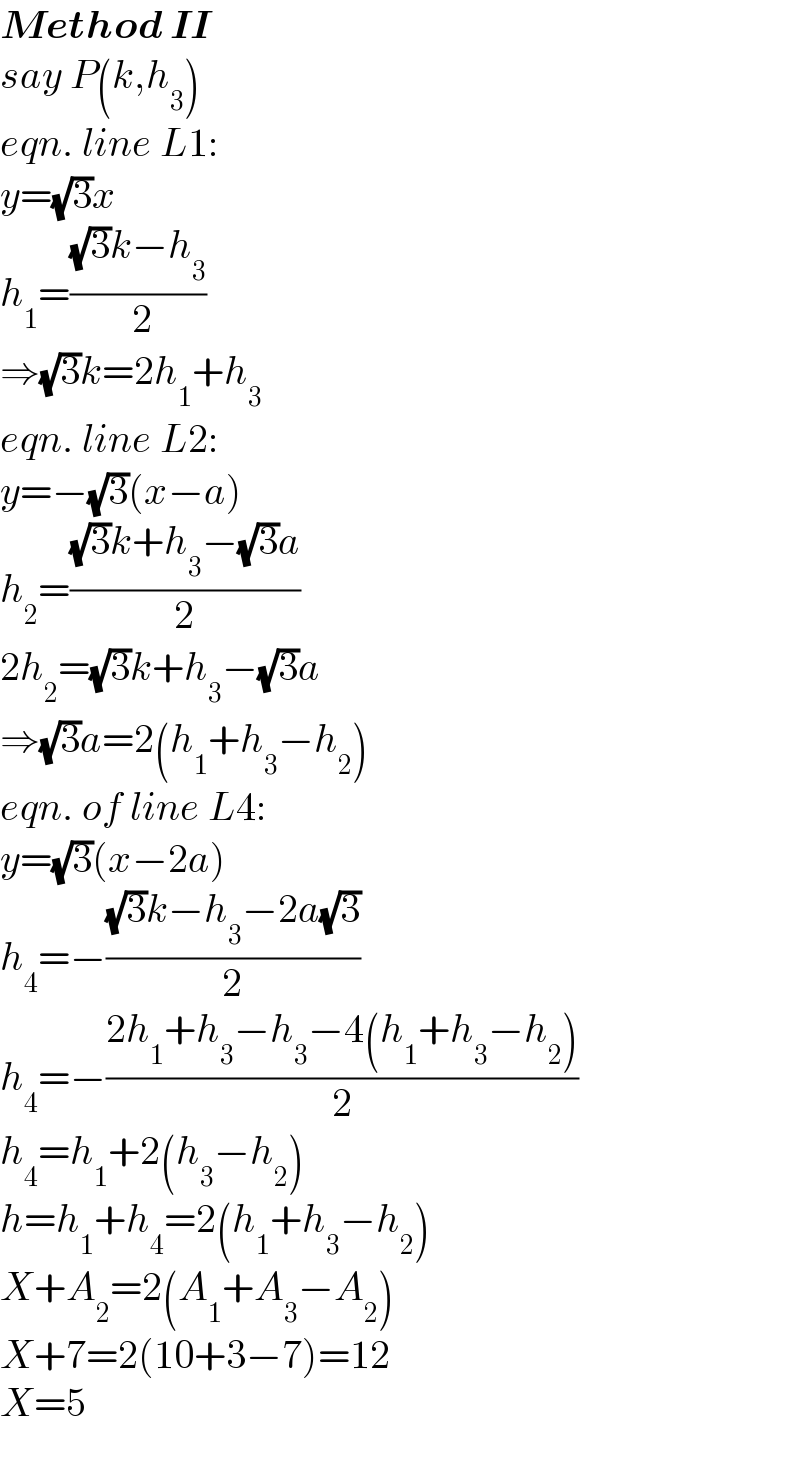
Method II say P(k,h_3 ) eqn. line L1: y=(√3)x h_1 =(((√3)k−h_3 )/( 2)) ⇒(√3)k=2h_1 +h_3 eqn. line L2: y=−(√3)(x−a) h_2 =(((√3)k+h_3 −(√3)a)/2) 2h_2 =(√3)k+h_3 −(√3)a ⇒(√3)a=2(h_1 +h_3 −h_2 ) eqn. of line L4: y=(√3)(x−2a) h_4 =−(((√3)k−h_3 −2a(√3))/2) h_4 =−((2h_1 +h_3 −h_3 −4(h_1 +h_3 −h_2 ))/2) h_4 =h_1 +2(h_3 −h_2 ) h=h_1 +h_4 =2(h_1 +h_3 −h_2 ) X+A_2 =2(A_1 +A_3 −A_2 ) X+7=2(10+3−7)=12 X=5

$$\boldsymbol{{Method}}\:\boldsymbol{{II}} \\ $$$${say}\:{P}\left({k},{h}_{\mathrm{3}} \right) \\ $$$${eqn}.\:{line}\:{L}\mathrm{1}: \\ $$$${y}=\sqrt{\mathrm{3}}{x} \\ $$$${h}_{\mathrm{1}} =\frac{\sqrt{\mathrm{3}}{k}−{h}_{\mathrm{3}} }{\:\mathrm{2}} \\ $$$$\Rightarrow\sqrt{\mathrm{3}}{k}=\mathrm{2}{h}_{\mathrm{1}} +{h}_{\mathrm{3}} \\ $$$${eqn}.\:{line}\:{L}\mathrm{2}: \\ $$$${y}=−\sqrt{\mathrm{3}}\left({x}−{a}\right) \\ $$$${h}_{\mathrm{2}} =\frac{\sqrt{\mathrm{3}}{k}+{h}_{\mathrm{3}} −\sqrt{\mathrm{3}}{a}}{\mathrm{2}} \\ $$$$\mathrm{2}{h}_{\mathrm{2}} =\sqrt{\mathrm{3}}{k}+{h}_{\mathrm{3}} −\sqrt{\mathrm{3}}{a} \\ $$$$\Rightarrow\sqrt{\mathrm{3}}{a}=\mathrm{2}\left({h}_{\mathrm{1}} +{h}_{\mathrm{3}} −{h}_{\mathrm{2}} \right) \\ $$$${eqn}.\:{of}\:{line}\:{L}\mathrm{4}: \\ $$$${y}=\sqrt{\mathrm{3}}\left({x}−\mathrm{2}{a}\right) \\ $$$${h}_{\mathrm{4}} =−\frac{\sqrt{\mathrm{3}}{k}−{h}_{\mathrm{3}} −\mathrm{2}{a}\sqrt{\mathrm{3}}}{\mathrm{2}} \\ $$$${h}_{\mathrm{4}} =−\frac{\mathrm{2}{h}_{\mathrm{1}} +{h}_{\mathrm{3}} −{h}_{\mathrm{3}} −\mathrm{4}\left({h}_{\mathrm{1}} +{h}_{\mathrm{3}} −{h}_{\mathrm{2}} \right)}{\mathrm{2}} \\ $$$${h}_{\mathrm{4}} ={h}_{\mathrm{1}} +\mathrm{2}\left({h}_{\mathrm{3}} −{h}_{\mathrm{2}} \right) \\ $$$${h}={h}_{\mathrm{1}} +{h}_{\mathrm{4}} =\mathrm{2}\left({h}_{\mathrm{1}} +{h}_{\mathrm{3}} −{h}_{\mathrm{2}} \right) \\ $$$${X}+{A}_{\mathrm{2}} =\mathrm{2}\left({A}_{\mathrm{1}} +{A}_{\mathrm{3}} −{A}_{\mathrm{2}} \right) \\ $$$${X}+\mathrm{7}=\mathrm{2}\left(\mathrm{10}+\mathrm{3}−\mathrm{7}\right)=\mathrm{12} \\ $$$${X}=\mathrm{5} \\ $$

Commented by mr W last updated on 21/Feb/23

Terms of Service

Privacy Policy

Contact: info@tinkutara.com