
Question Number 187752 by Noorzai last updated on 21/Feb/23
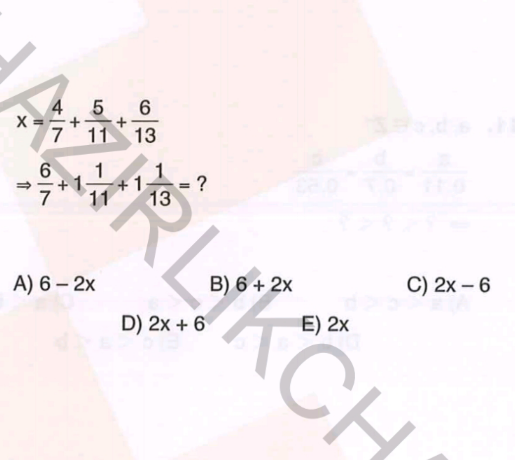
Commented by Noorzai last updated on 21/Feb/23

$$ \\ $$
Commented by Noorzai last updated on 21/Feb/23
$${can}\:{you}\:{help}\:{me}\:{to}\:{answer} \\ $$
Commented by mr W last updated on 21/Feb/23

$${there}\:{are}\:{at}\:{least}\:{one}\:{million}\:{of}\:{such} \\ $$$${similar}\:{questions}.\:{do}\:{you}\:{want}\:{to} \\ $$$${post}\:{all}\:{of}\:{them}?\:{if}\:{you}\:{don}'{t}\:{want}\:{to} \\ $$$${or}\:{are}\:{not}\:{able}\:{to}\:{learn}\:{from}\:{the}\: \\ $$$${similar}\:{questions}\:{which}\:{other}\:{people} \\ $$$${have}\:{solved}\:{for}\:{you},\:{then}\:{it}\:{makes}\:{no}\: \\ $$$${sense}\:{to}\:{solve}\:{this}\:{question}\:{for}\:{you},\: \\ $$$${because}\:{you}\:{will}\:{also}\:{not}\:{learn}\:{from}\: \\ $$$${this}\:{one}. \\ $$
Commented by mr W last updated on 21/Feb/23

$${no},\:{i}\:{won}'{t},\:{because}\:{i}\:{don}'{t}\:{believe} \\ $$$${that}\:{someone}\:{can}\:{understand} \\ $$$$\:\underset{\mathrm{0}} {\int}^{\infty} \frac{\mathrm{ln}\:{x}}{\mathrm{1}−{x}^{\mathrm{7}} }\:{dx},\:{but}\:{is}\:{not}\:{able}\:{to}\:{solve}\:{a} \\ $$$${kindergarten}\:{question}. \\ $$
Commented by mahdipoor last updated on 21/Feb/23
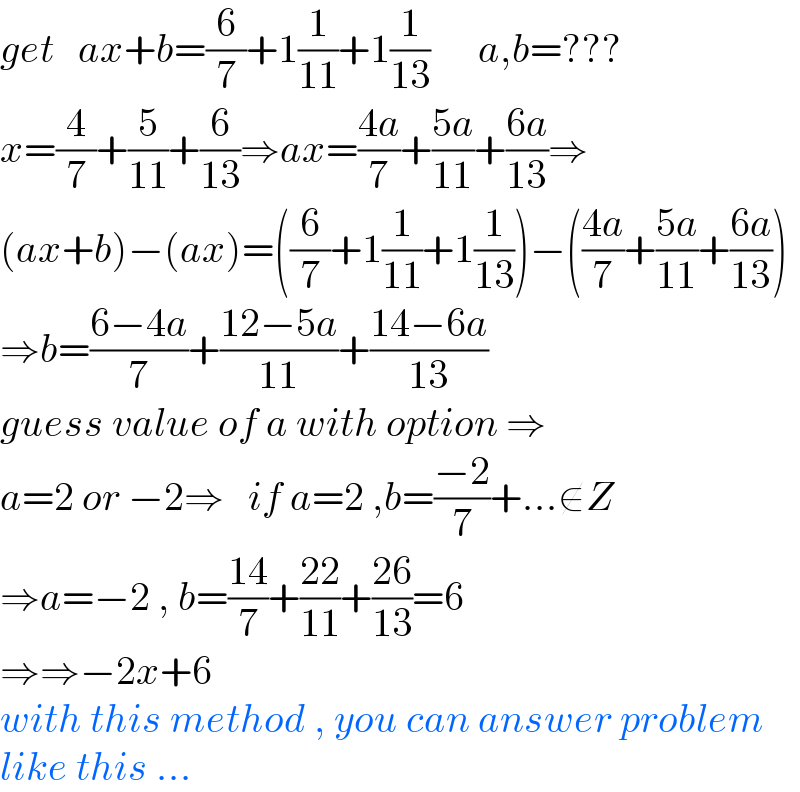
$${get}\:\:\:{ax}+{b}=\frac{\mathrm{6}}{\mathrm{7}}+\mathrm{1}\frac{\mathrm{1}}{\mathrm{11}}+\mathrm{1}\frac{\mathrm{1}}{\mathrm{13}}\:\:\:\:\:\:{a},{b}=??? \\ $$$${x}=\frac{\mathrm{4}}{\mathrm{7}}+\frac{\mathrm{5}}{\mathrm{11}}+\frac{\mathrm{6}}{\mathrm{13}}\Rightarrow{ax}=\frac{\mathrm{4}{a}}{\mathrm{7}}+\frac{\mathrm{5}{a}}{\mathrm{11}}+\frac{\mathrm{6}{a}}{\mathrm{13}}\Rightarrow \\ $$$$\left({ax}+{b}\right)−\left({ax}\right)=\left(\frac{\mathrm{6}}{\mathrm{7}}+\mathrm{1}\frac{\mathrm{1}}{\mathrm{11}}+\mathrm{1}\frac{\mathrm{1}}{\mathrm{13}}\right)−\left(\frac{\mathrm{4}{a}}{\mathrm{7}}+\frac{\mathrm{5}{a}}{\mathrm{11}}+\frac{\mathrm{6}{a}}{\mathrm{13}}\right) \\ $$$$\Rightarrow{b}=\frac{\mathrm{6}−\mathrm{4}{a}}{\mathrm{7}}+\frac{\mathrm{12}−\mathrm{5}{a}}{\mathrm{11}}+\frac{\mathrm{14}−\mathrm{6}{a}}{\mathrm{13}} \\ $$$${guess}\:{value}\:{of}\:{a}\:{with}\:{option}\:\Rightarrow \\ $$$${a}=\mathrm{2}\:{or}\:−\mathrm{2}\Rightarrow\:\:\:{if}\:{a}=\mathrm{2}\:,{b}=\frac{−\mathrm{2}}{\mathrm{7}}+...\notin{Z} \\ $$$$\Rightarrow{a}=−\mathrm{2}\:,\:{b}=\frac{\mathrm{14}}{\mathrm{7}}+\frac{\mathrm{22}}{\mathrm{11}}+\frac{\mathrm{26}}{\mathrm{13}}=\mathrm{6} \\ $$$$\Rightarrow\Rightarrow−\mathrm{2}{x}+\mathrm{6}\:\:\: \\ $$$${with}\:{this}\:{method}\:,\:{you}\:{can}\:{answer}\:{problem} \\ $$$${like}\:{this}\:...\: \\ $$
