
Question Number 18307 by mondodotto@gmail.com last updated on 18/Jul/17
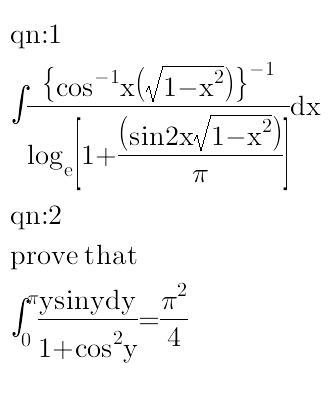
Answered by ajfour last updated on 18/Jul/17
![Q.2 I=∫_0 ^( π) ((ysin ydy)/(1+cos^2 y))=∫_0 ^( π) (((π−y)sin ydy)/(1+cos^2 y)) =π∫_0 ^( π) ((sin ydy)/(1+cos^2 y))−I ⇒ 2I=−πtan^(−1) (cos y)∣_0 ^π or I=−(π/2)[tan^(−1) (−1)−tan^(−1) 1] =−(π/2)(−(π/4)−(π/4)) I=(π^2 /4) .](Q18309.png)
$$\mathrm{Q}.\mathrm{2} \\ $$$$\mathrm{I}=\int_{\mathrm{0}} ^{\:\:\pi} \frac{\mathrm{ysin}\:\mathrm{ydy}}{\mathrm{1}+\mathrm{cos}\:^{\mathrm{2}} \mathrm{y}}=\int_{\mathrm{0}} ^{\:\:\pi} \frac{\left(\pi−\mathrm{y}\right)\mathrm{sin}\:\mathrm{ydy}}{\mathrm{1}+\mathrm{cos}\:^{\mathrm{2}} \mathrm{y}} \\ $$$$\:\:=\pi\int_{\mathrm{0}} ^{\:\:\pi} \frac{\mathrm{sin}\:\mathrm{ydy}}{\mathrm{1}+\mathrm{cos}\:^{\mathrm{2}} \mathrm{y}}−\mathrm{I} \\ $$$$\Rightarrow\:\:\:\mathrm{2I}=−\pi\mathrm{tan}^{−\mathrm{1}} \left(\mathrm{cos}\:\mathrm{y}\right)\mid_{\mathrm{0}} ^{\pi} \\ $$$$\:\:\:\:\:\mathrm{or}\:\:\:\mathrm{I}=−\frac{\pi}{\mathrm{2}}\left[\mathrm{tan}^{−\mathrm{1}} \left(−\mathrm{1}\right)−\mathrm{tan}^{−\mathrm{1}} \mathrm{1}\right] \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:=−\frac{\pi}{\mathrm{2}}\left(−\frac{\pi}{\mathrm{4}}−\frac{\pi}{\mathrm{4}}\right) \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{I}=\frac{\pi^{\mathrm{2}} }{\mathrm{4}}\:. \\ $$
