
Question Number 182256 by mathlove last updated on 06/Dec/22
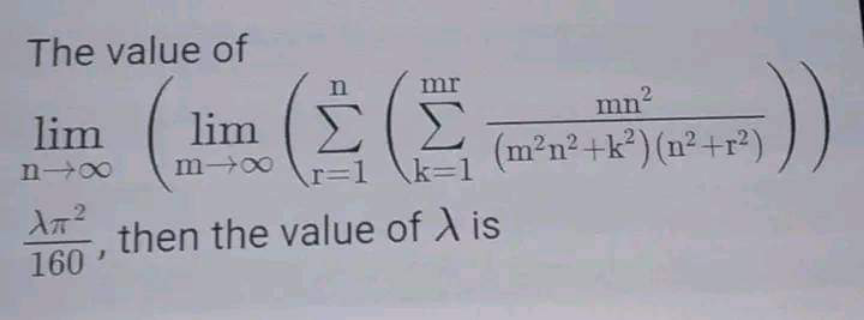
Answered by Ar Brandon last updated on 06/Dec/22
![L=lim_(n→∞) (lim_(m→∞) (Σ_(r=1) ^n (Σ_(k=1) ^(mr) ((mn^2 )/((m^2 n^2 +k^2 )(n^2 +r^2 ))))) =lim_(n→∞) (Σ_(r=1) ^n lim_(m→∞) ((1/m)Σ_(k=1) ^(mr) (n^2 /((n^2 +(k^2 /m^2 ))(n^2 +r^2 ))))) =lim_(n→∞) (Σ_(r=1) ^n (n^2 /(n^2 +r^2 ))∫_0 ^( r) (dx/((n^2 +x^2 ))))=lim_(n→∞) (Σ_(r=1) ^n (n^2 /(n^2 +r^2 ))[(1/n)arctan((x/n))]_0 ^r ) =lim_(n→∞) (Σ_(r=1) ^n (n/(n^2 +r^2 ))arctan((r/n)))=lim_(n→∞) (1/n)Σ_(r=1) ^n (1/(1+(r^2 /n^2 )))∙arctan((r/n)) =∫_0 ^1 ((arctanx)/(1+x^2 ))dx=∫_0 ^1 arctan(x)d(arctanx)=[(((arctanx)^2 )/2)]_0 ^1 =(π^2 /(32))](Q182259.png)
$$\mathscr{L}=\underset{{n}\rightarrow\infty} {\mathrm{lim}}\left(\underset{{m}\rightarrow\infty} {\mathrm{lim}}\left(\underset{{r}=\mathrm{1}} {\overset{{n}} {\sum}}\left(\underset{{k}=\mathrm{1}} {\overset{{mr}} {\sum}}\frac{{mn}^{\mathrm{2}} }{\left({m}^{\mathrm{2}} {n}^{\mathrm{2}} +{k}^{\mathrm{2}} \right)\left({n}^{\mathrm{2}} +{r}^{\mathrm{2}} \right)}\right)\right)\right. \\ $$$$\:\:\:\:=\underset{{n}\rightarrow\infty} {\mathrm{lim}}\left(\underset{{r}=\mathrm{1}} {\overset{{n}} {\sum}}\underset{{m}\rightarrow\infty} {\mathrm{lim}}\left(\frac{\mathrm{1}}{{m}}\underset{{k}=\mathrm{1}} {\overset{{mr}} {\sum}}\frac{{n}^{\mathrm{2}} }{\left({n}^{\mathrm{2}} +\frac{{k}^{\mathrm{2}} }{{m}^{\mathrm{2}} }\right)\left({n}^{\mathrm{2}} +{r}^{\mathrm{2}} \right)}\right)\right) \\ $$$$\:\:\:\:=\underset{{n}\rightarrow\infty} {\mathrm{lim}}\left(\underset{{r}=\mathrm{1}} {\overset{{n}} {\sum}}\frac{{n}^{\mathrm{2}} }{{n}^{\mathrm{2}} +{r}^{\mathrm{2}} }\int_{\mathrm{0}} ^{\:{r}} \frac{{dx}}{\left({n}^{\mathrm{2}} +{x}^{\mathrm{2}} \right)}\right)=\underset{{n}\rightarrow\infty} {\mathrm{lim}}\left(\underset{{r}=\mathrm{1}} {\overset{{n}} {\sum}}\frac{{n}^{\mathrm{2}} }{{n}^{\mathrm{2}} +{r}^{\mathrm{2}} }\left[\frac{\mathrm{1}}{{n}}\mathrm{arctan}\left(\frac{{x}}{{n}}\right)\right]_{\mathrm{0}} ^{{r}} \right) \\ $$$$\:\:\:\:=\underset{{n}\rightarrow\infty} {\mathrm{lim}}\left(\underset{{r}=\mathrm{1}} {\overset{{n}} {\sum}}\frac{{n}}{{n}^{\mathrm{2}} +{r}^{\mathrm{2}} }\mathrm{arctan}\left(\frac{{r}}{{n}}\right)\right)=\underset{{n}\rightarrow\infty} {\mathrm{lim}}\frac{\mathrm{1}}{{n}}\underset{{r}=\mathrm{1}} {\overset{{n}} {\sum}}\frac{\mathrm{1}}{\mathrm{1}+\frac{{r}^{\mathrm{2}} }{{n}^{\mathrm{2}} }}\centerdot\mathrm{arctan}\left(\frac{{r}}{{n}}\right) \\ $$$$\:\:\:\:=\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\mathrm{arctan}{x}}{\mathrm{1}+{x}^{\mathrm{2}} }{dx}=\int_{\mathrm{0}} ^{\mathrm{1}} \mathrm{arctan}\left({x}\right){d}\left(\mathrm{arctan}{x}\right)=\left[\frac{\left(\mathrm{arctan}{x}\right)^{\mathrm{2}} }{\mathrm{2}}\right]_{\mathrm{0}} ^{\mathrm{1}} =\frac{\pi^{\mathrm{2}} }{\mathrm{32}} \\ $$
