Question and Answers Forum

All Questions      Topic List

None Questions

Previous in All Question      Next in All Question      

Previous in None      Next in None      

Question Number 18146 by mondodotto@gmail.com last updated on 15/Jul/17

Commented by prakash jain last updated on 16/Jul/17

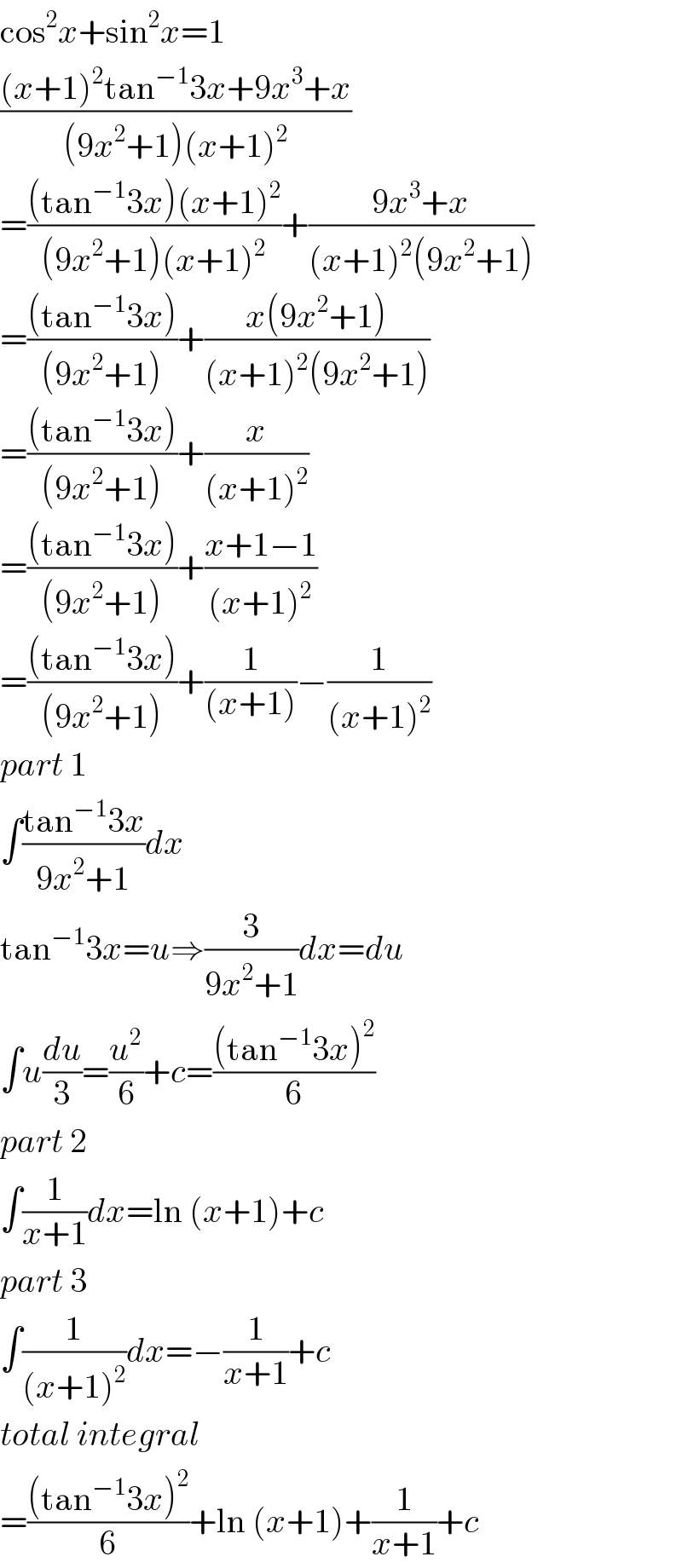
cos^2 x+sin^2 x=1 (((x+1)^2 tan^(−1) 3x+9x^3 +x)/((9x^2 +1)(x+1)^2 )) =(((tan^(−1) 3x)(x+1)^2 )/((9x^2 +1)(x+1)^2 ))+((9x^3 +x)/((x+1)^2 (9x^2 +1))) =(((tan^(−1) 3x))/((9x^2 +1)))+((x(9x^2 +1))/((x+1)^2 (9x^2 +1))) =(((tan^(−1) 3x))/((9x^2 +1)))+(x/((x+1)^2 )) =(((tan^(−1) 3x))/((9x^2 +1)))+((x+1−1)/((x+1)^2 )) =(((tan^(−1) 3x))/((9x^2 +1)))+(1/((x+1)))−(1/((x+1)^2 )) part 1 ∫((tan^(−1) 3x)/(9x^2 +1))dx tan^(−1) 3x=u⇒(3/(9x^2 +1))dx=du ∫u(du/3)=(u^2 /6)+c=(((tan^(−1) 3x)^2 )/6) part 2 ∫(1/(x+1))dx=ln (x+1)+c part 3 ∫(1/((x+1)^2 ))dx=−(1/(x+1))+c total integral =(((tan^(−1) 3x)^2 )/6)+ln (x+1)+(1/(x+1))+c

$$\mathrm{cos}^{\mathrm{2}} {x}+\mathrm{sin}^{\mathrm{2}} {x}=\mathrm{1} \\ $$$$\frac{\left({x}+\mathrm{1}\right)^{\mathrm{2}} \mathrm{tan}^{−\mathrm{1}} \mathrm{3}{x}+\mathrm{9}{x}^{\mathrm{3}} +{x}}{\left(\mathrm{9}{x}^{\mathrm{2}} +\mathrm{1}\right)\left({x}+\mathrm{1}\right)^{\mathrm{2}} } \\ $$$$=\frac{\left(\mathrm{tan}^{−\mathrm{1}} \mathrm{3}{x}\right)\left({x}+\mathrm{1}\right)^{\mathrm{2}} }{\left(\mathrm{9}{x}^{\mathrm{2}} +\mathrm{1}\right)\left({x}+\mathrm{1}\right)^{\mathrm{2}} }+\frac{\mathrm{9}{x}^{\mathrm{3}} +{x}}{\left({x}+\mathrm{1}\right)^{\mathrm{2}} \left(\mathrm{9}{x}^{\mathrm{2}} +\mathrm{1}\right)} \\ $$$$=\frac{\left(\mathrm{tan}^{−\mathrm{1}} \mathrm{3}{x}\right)}{\left(\mathrm{9}{x}^{\mathrm{2}} +\mathrm{1}\right)}+\frac{{x}\left(\mathrm{9}{x}^{\mathrm{2}} +\mathrm{1}\right)}{\left({x}+\mathrm{1}\right)^{\mathrm{2}} \left(\mathrm{9}{x}^{\mathrm{2}} +\mathrm{1}\right)} \\ $$$$=\frac{\left(\mathrm{tan}^{−\mathrm{1}} \mathrm{3}{x}\right)}{\left(\mathrm{9}{x}^{\mathrm{2}} +\mathrm{1}\right)}+\frac{{x}}{\left({x}+\mathrm{1}\right)^{\mathrm{2}} } \\ $$$$=\frac{\left(\mathrm{tan}^{−\mathrm{1}} \mathrm{3}{x}\right)}{\left(\mathrm{9}{x}^{\mathrm{2}} +\mathrm{1}\right)}+\frac{{x}+\mathrm{1}−\mathrm{1}}{\left({x}+\mathrm{1}\right)^{\mathrm{2}} } \\ $$$$=\frac{\left(\mathrm{tan}^{−\mathrm{1}} \mathrm{3}{x}\right)}{\left(\mathrm{9}{x}^{\mathrm{2}} +\mathrm{1}\right)}+\frac{\mathrm{1}}{\left({x}+\mathrm{1}\right)}−\frac{\mathrm{1}}{\left({x}+\mathrm{1}\right)^{\mathrm{2}} } \\ $$$${part}\:\mathrm{1} \\ $$$$\int\frac{\mathrm{tan}^{−\mathrm{1}} \mathrm{3}{x}}{\mathrm{9}{x}^{\mathrm{2}} +\mathrm{1}}{dx} \\ $$$$\mathrm{tan}^{−\mathrm{1}} \mathrm{3}{x}={u}\Rightarrow\frac{\mathrm{3}}{\mathrm{9}{x}^{\mathrm{2}} +\mathrm{1}}{dx}={du} \\ $$$$\int{u}\frac{{du}}{\mathrm{3}}=\frac{{u}^{\mathrm{2}} }{\mathrm{6}}+{c}=\frac{\left(\mathrm{tan}^{−\mathrm{1}} \mathrm{3}{x}\right)^{\mathrm{2}} }{\mathrm{6}} \\ $$$${part}\:\mathrm{2} \\ $$$$\int\frac{\mathrm{1}}{{x}+\mathrm{1}}{dx}=\mathrm{ln}\:\left({x}+\mathrm{1}\right)+{c} \\ $$$${part}\:\mathrm{3} \\ $$$$\int\frac{\mathrm{1}}{\left({x}+\mathrm{1}\right)^{\mathrm{2}} }{dx}=−\frac{\mathrm{1}}{{x}+\mathrm{1}}+{c} \\ $$$${total}\:{integral} \\ $$$$=\frac{\left(\mathrm{tan}^{−\mathrm{1}} \mathrm{3}{x}\right)^{\mathrm{2}} }{\mathrm{6}}+\mathrm{ln}\:\left({x}+\mathrm{1}\right)+\frac{\mathrm{1}}{{x}+\mathrm{1}}+{c} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com