Question and Answers Forum

All Questions      Topic List

Algebra Questions

Previous in All Question      Next in All Question      

Previous in Algebra      Next in Algebra      

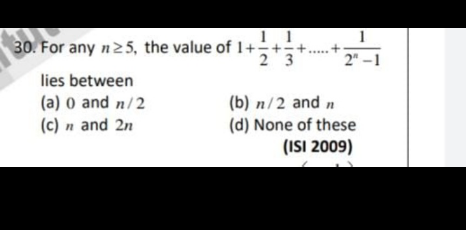
Question Number 178073 by infinityaction last updated on 12/Oct/22

Commented by Rasheed.Sindhi last updated on 12/Oct/22

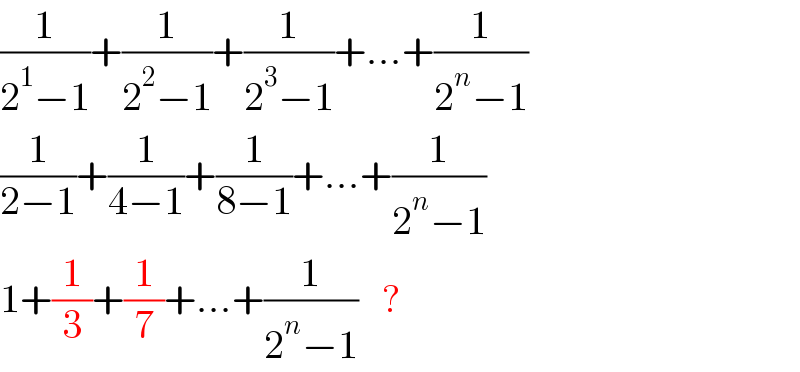
(1/(2^1 −1))+(1/(2^2 −1))+(1/(2^3 −1))+...+(1/(2^n −1)) (1/(2−1))+(1/(4−1))+(1/(8−1))+...+(1/(2^n −1)) 1+(1/3)+(1/7)+...+(1/(2^n −1)) ?

$$\frac{\mathrm{1}}{\mathrm{2}^{\mathrm{1}} −\mathrm{1}}+\frac{\mathrm{1}}{\mathrm{2}^{\mathrm{2}} −\mathrm{1}}+\frac{\mathrm{1}}{\mathrm{2}^{\mathrm{3}} −\mathrm{1}}+...+\frac{\mathrm{1}}{\mathrm{2}^{{n}} −\mathrm{1}} \\ $$$$\frac{\mathrm{1}}{\mathrm{2}−\mathrm{1}}+\frac{\mathrm{1}}{\mathrm{4}−\mathrm{1}}+\frac{\mathrm{1}}{\mathrm{8}−\mathrm{1}}+...+\frac{\mathrm{1}}{\mathrm{2}^{{n}} −\mathrm{1}} \\ $$$$\mathrm{1}+\frac{\mathrm{1}}{\mathrm{3}}+\frac{\mathrm{1}}{\mathrm{7}}+...+\frac{\mathrm{1}}{\mathrm{2}^{{n}} −\mathrm{1}}\:\:\:\:? \\ $$

Commented by mr W last updated on 12/Oct/22

i think it′s Σ_(k=1) ^(2^n −1) (1/k)

$${i}\:{think}\:{it}'{s} \\ $$$$\underset{{k}=\mathrm{1}} {\overset{\mathrm{2}^{{n}} −\mathrm{1}} {\sum}}\frac{\mathrm{1}}{{k}} \\ $$

Commented by Rasheed.Sindhi last updated on 12/Oct/22

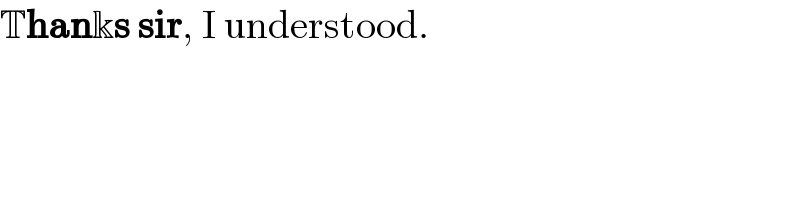
Thanks sir, I understood.

$$\mathbb{T}\boldsymbol{\mathrm{han}}\Bbbk\boldsymbol{\mathrm{s}}\:\boldsymbol{\mathrm{sir}},\:\mathrm{I}\:\mathrm{understood}. \\ $$

Commented by infinityaction last updated on 13/Oct/22

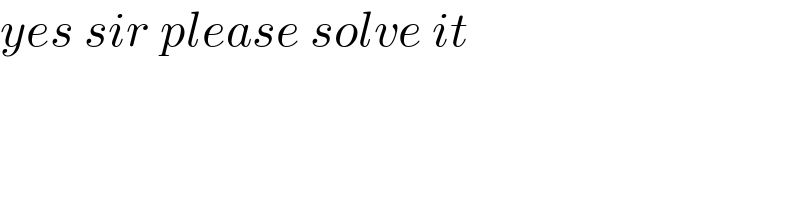
yes sir please solve it

$${yes}\:{sir}\:{please}\:{solve}\:{it}\: \\ $$

Answered by mindispower last updated on 13/Oct/22

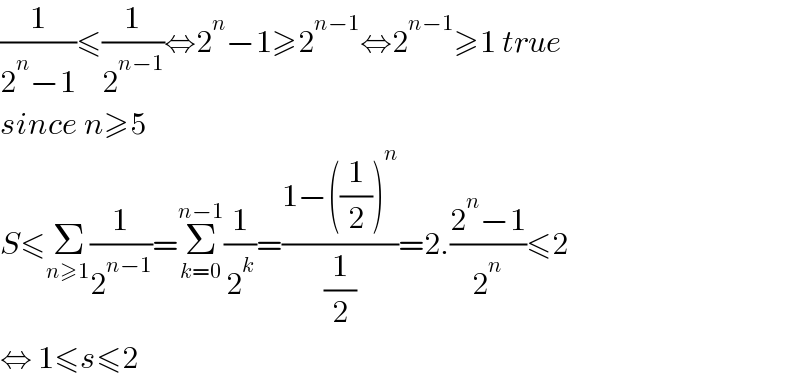
(1/(2^n −1))≤(1/2^(n−1) )⇔2^n −1≥2^(n−1) ⇔2^(n−1) ≥1 true since n≥5 S≤Σ_(n≥1) (1/2^(n−1) )=Σ_(k=0) ^(n−1) (1/2^k )=((1−((1/2))^n )/(1/2))=2.((2^n −1)/2^n )≤2 ⇔ 1≤s≤2

$$\frac{\mathrm{1}}{\mathrm{2}^{{n}} −\mathrm{1}}\leqslant\frac{\mathrm{1}}{\mathrm{2}^{{n}−\mathrm{1}} }\Leftrightarrow\mathrm{2}^{{n}} −\mathrm{1}\geqslant\mathrm{2}^{{n}−\mathrm{1}} \Leftrightarrow\mathrm{2}^{{n}−\mathrm{1}} \geqslant\mathrm{1}\:{true} \\ $$$${since}\:{n}\geqslant\mathrm{5} \\ $$$${S}\leqslant\underset{{n}\geqslant\mathrm{1}} {\sum}\frac{\mathrm{1}}{\mathrm{2}^{{n}−\mathrm{1}} }=\underset{{k}=\mathrm{0}} {\overset{{n}−\mathrm{1}} {\sum}}\frac{\mathrm{1}}{\mathrm{2}^{{k}} }=\frac{\mathrm{1}−\left(\frac{\mathrm{1}}{\mathrm{2}}\right)^{{n}} }{\frac{\mathrm{1}}{\mathrm{2}}}=\mathrm{2}.\frac{\mathrm{2}^{{n}} −\mathrm{1}}{\mathrm{2}^{{n}} }\leqslant\mathrm{2} \\ $$$$\Leftrightarrow\:\mathrm{1}\leqslant{s}\leqslant\mathrm{2} \\ $$

Commented by mindispower last updated on 13/Oct/22

2≤(5/2)≤(n/2)

$$\mathrm{2}\leqslant\frac{\mathrm{5}}{\mathrm{2}}\leqslant\frac{{n}}{\mathrm{2}} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com