Question and Answers Forum

All Questions      Topic List

None Questions

Previous in All Question      Next in All Question      

Previous in None      Next in None      

Question Number 177884 by DAVONG last updated on 10/Oct/22

Answered by mr W last updated on 10/Oct/22

6 black and n red balls in bag. to take 3 balls there are totally C_3 ^(n+6) ways. to take 1 black and 2 red balls there are C_1 ^6 C_2 ^n ways. P_n =((C_1 ^6 C_2 ^n )/C_3 ^(n+6) ) =((6×n(n−1)×6)/(2×(n+6)(n+5)(n+4))) =((18n(n−1))/((n+6)(n+5)(n+4))) P_n is maximum when n=11 or 12 P_(n,max) =((18×11×10)/(17×16×15))=((33)/(68)) ⇒(D)

$$\mathrm{6}\:{black}\:{and}\:{n}\:{red}\:{balls}\:{in}\:{bag}. \\ $$$${to}\:{take}\:\mathrm{3}\:{balls}\:{there}\:{are}\:{totally} \\ $$$${C}_{\mathrm{3}} ^{{n}+\mathrm{6}} \:{ways}. \\ $$$${to}\:{take}\:\mathrm{1}\:{black}\:{and}\:\mathrm{2}\:{red}\:{balls}\:{there} \\ $$$${are}\:{C}_{\mathrm{1}} ^{\mathrm{6}} {C}_{\mathrm{2}} ^{{n}} \:{ways}. \\ $$$${P}_{{n}} =\frac{{C}_{\mathrm{1}} ^{\mathrm{6}} {C}_{\mathrm{2}} ^{{n}} }{{C}_{\mathrm{3}} ^{{n}+\mathrm{6}} } \\ $$$$\:\:\:\:\:=\frac{\mathrm{6}×{n}\left({n}−\mathrm{1}\right)×\mathrm{6}}{\mathrm{2}×\left({n}+\mathrm{6}\right)\left({n}+\mathrm{5}\right)\left({n}+\mathrm{4}\right)} \\ $$$$\:\:\:\:\:=\frac{\mathrm{18}{n}\left({n}−\mathrm{1}\right)}{\left({n}+\mathrm{6}\right)\left({n}+\mathrm{5}\right)\left({n}+\mathrm{4}\right)} \\ $$$${P}_{{n}} \:{is}\:{maximum}\:{when}\:{n}=\mathrm{11}\:{or}\:\mathrm{12} \\ $$$${P}_{{n},{max}} =\frac{\mathrm{18}×\mathrm{11}×\mathrm{10}}{\mathrm{17}×\mathrm{16}×\mathrm{15}}=\frac{\mathrm{33}}{\mathrm{68}} \\ $$$$\Rightarrow\left({D}\right) \\ $$

Commented by mr W last updated on 10/Oct/22

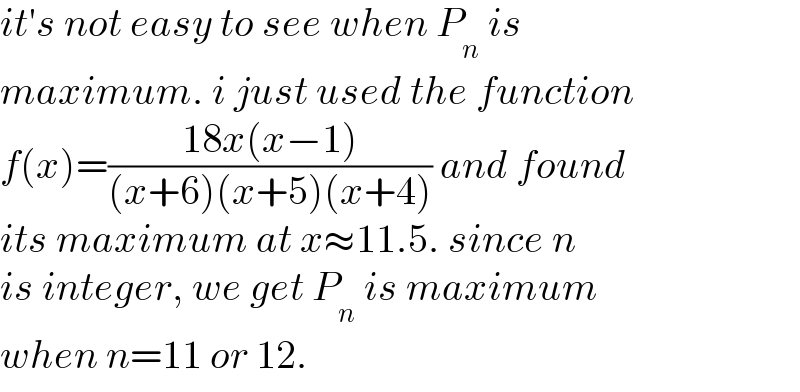
it′s not easy to see when P_n is maximum. i just used the function f(x)=((18x(x−1))/((x+6)(x+5)(x+4))) and found its maximum at x≈11.5. since n is integer, we get P_n is maximum when n=11 or 12.

$${it}'{s}\:{not}\:{easy}\:{to}\:{see}\:{when}\:{P}_{{n}} \:{is}\: \\ $$$${maximum}.\:{i}\:{just}\:{used}\:{the}\:{function} \\ $$$${f}\left({x}\right)=\frac{\mathrm{18}{x}\left({x}−\mathrm{1}\right)}{\left({x}+\mathrm{6}\right)\left({x}+\mathrm{5}\right)\left({x}+\mathrm{4}\right)}\:{and}\:{found} \\ $$$${its}\:{maximum}\:{at}\:{x}\approx\mathrm{11}.\mathrm{5}.\:{since}\:{n} \\ $$$${is}\:{integer},\:{we}\:{get}\:{P}_{{n}} \:{is}\:{maximum} \\ $$$${when}\:{n}=\mathrm{11}\:{or}\:\mathrm{12}. \\ $$

Commented by Acem last updated on 10/Oct/22

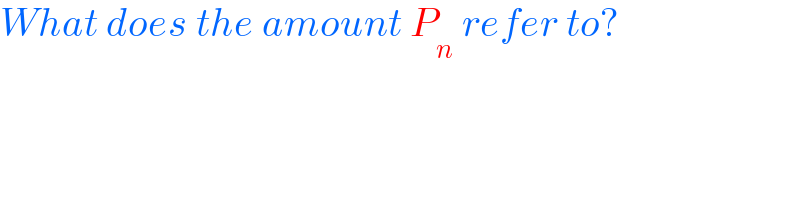
What does the amount P_n refer to?

$${What}\:{does}\:{the}\:{amount}\:{P}_{{n}} \:{refer}\:{to}? \\ $$

Commented by Acem last updated on 10/Oct/22

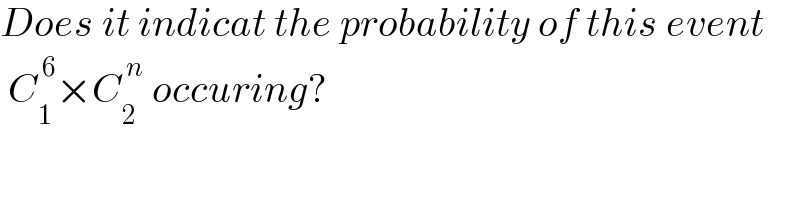
Does it indicat the probability of this event C_1 ^( 6) ×C_2 ^( n) occuring?

$${Does}\:{it}\:{indicat}\:{the}\:{probability}\:{of}\:{this}\:{event} \\ $$$$\:{C}_{\mathrm{1}} ^{\:\mathrm{6}} ×{C}_{\mathrm{2}} ^{\:{n}} \:{occuring}? \\ $$

Commented by mr W last updated on 10/Oct/22

yes. as the question says: P_n is the probability of taking 1 black ball and 2 red balls.

$${yes}.\:{as}\:{the}\:{question}\:{says}:\:{P}_{{n}} \:{is}\:{the} \\ $$$${probability}\:{of}\:{taking}\:\mathrm{1}\:{black}\:{ball}\:{and} \\ $$$$\mathrm{2}\:{red}\:{balls}. \\ $$

Commented by Acem last updated on 11/Oct/22

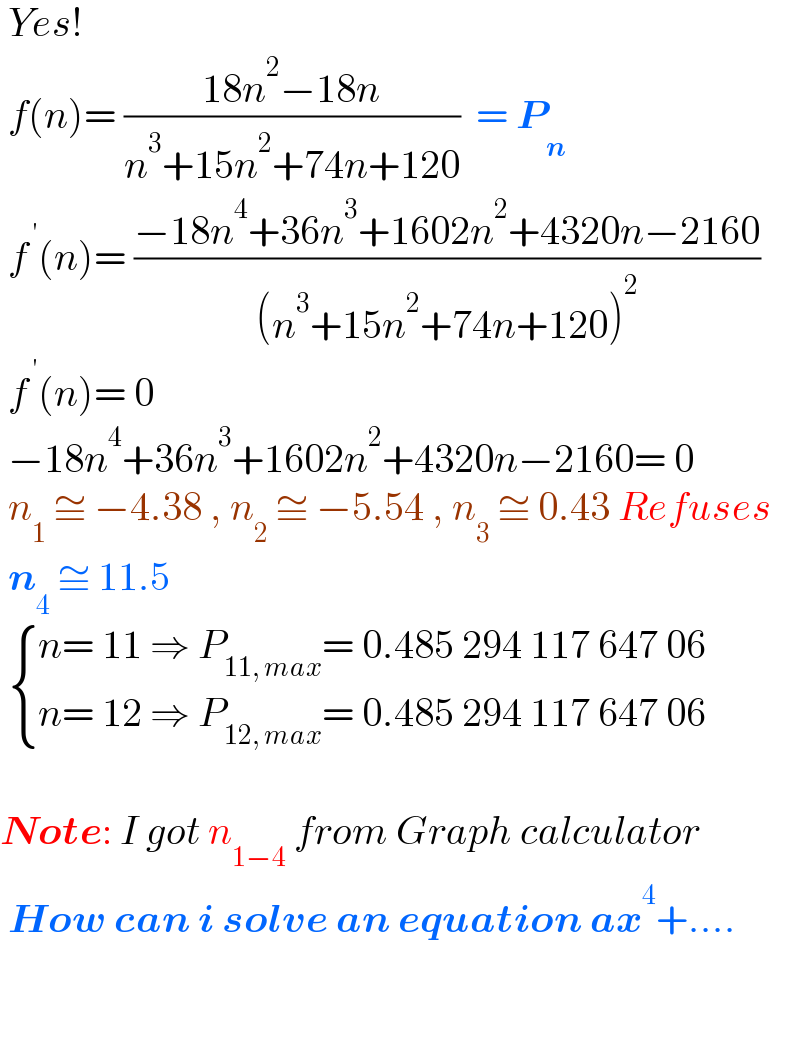
Yes! f(n)= ((18n^2 −18n)/(n^3 +15n^2 +74n+120)) = P_n f^( ′) (n)= ((−18n^4 +36n^3 +1602n^2 +4320n−2160)/((n^3 +15n^2 +74n+120)^2 )) f^( ′) (n)= 0 −18n^4 +36n^3 +1602n^2 +4320n−2160= 0 n_1 ≅ −4.38 , n_2 ≅ −5.54 , n_3 ≅ 0.43 Refuses n_4 ≅ 11.5 { ((n= 11 ⇒ P_(11, max) = 0.485 294 117 647 06)),((n= 12 ⇒ P_(12, max) = 0.485 294 117 647 06)) :} Note: I got n_(1−4) from Graph calculator How can i solve an equation ax^4 +....

$$\:{Yes}!\: \\ $$$$\:{f}\left({n}\right)=\:\frac{\mathrm{18}{n}^{\mathrm{2}} −\mathrm{18}{n}}{{n}^{\mathrm{3}} +\mathrm{15}{n}^{\mathrm{2}} +\mathrm{74}{n}+\mathrm{120}}\:\:=\:\boldsymbol{{P}}_{\boldsymbol{{n}}} \\ $$$$\:{f}^{\:'} \left({n}\right)=\:\frac{−\mathrm{18}{n}^{\mathrm{4}} +\mathrm{36}{n}^{\mathrm{3}} +\mathrm{1602}{n}^{\mathrm{2}} +\mathrm{4320}{n}−\mathrm{2160}}{\left({n}^{\mathrm{3}} +\mathrm{15}{n}^{\mathrm{2}} +\mathrm{74}{n}+\mathrm{120}\right)^{\mathrm{2}} } \\ $$$$\:{f}^{\:'} \left({n}\right)=\:\mathrm{0} \\ $$$$\:−\mathrm{18}{n}^{\mathrm{4}} +\mathrm{36}{n}^{\mathrm{3}} +\mathrm{1602}{n}^{\mathrm{2}} +\mathrm{4320}{n}−\mathrm{2160}=\:\mathrm{0} \\ $$$$\:{n}_{\mathrm{1}} \:\cong\:−\mathrm{4}.\mathrm{38}\:,\:{n}_{\mathrm{2}} \:\cong\:−\mathrm{5}.\mathrm{54}\:,\:{n}_{\mathrm{3}} \:\cong\:\mathrm{0}.\mathrm{43}\:{Refuses} \\ $$$$\:\boldsymbol{{n}}_{\mathrm{4}} \:\cong\:\mathrm{11}.\mathrm{5} \\ $$$$\:\begin{cases}{{n}=\:\mathrm{11}\:\Rightarrow\:{P}_{\mathrm{11},\:{max}} =\:\mathrm{0}.\mathrm{485}\:\mathrm{294}\:\mathrm{117}\:\mathrm{647}\:\mathrm{06}}\\{{n}=\:\mathrm{12}\:\Rightarrow\:{P}_{\mathrm{12},\:{max}} =\:\mathrm{0}.\mathrm{485}\:\mathrm{294}\:\mathrm{117}\:\mathrm{647}\:\mathrm{06}}\end{cases} \\ $$$$\: \\ $$$$\boldsymbol{{Note}}:\:{I}\:{got}\:{n}_{\mathrm{1}−\mathrm{4}} \:{from}\:{Graph}\:{calculator} \\ $$$$\:\boldsymbol{{How}}\:\boldsymbol{{can}}\:\boldsymbol{{i}}\:\boldsymbol{{solve}}\:\boldsymbol{{an}}\:\boldsymbol{{equation}}\:\boldsymbol{{ax}}^{\mathrm{4}} +.... \\ $$$$ \\ $$$$ \\ $$

Commented by mr W last updated on 11/Oct/22

usually it is a quick way to determine P_(n, max ) using graph calculator. but really solving f′(n)=0 is not a good way, even impossible like above. besides, in an examination it is not allowed to use graph calculator. the best and yet easy and exact way is the following: we only need to find the n_(max) such that P_n ≥P_(n−1) , i.e. ((18n(n−1))/((n+6)(n+5)(n+4))) ≥((18(n−1)(n−2))/((n+5)(n+4)(n+3))) ⇒(n/(n+6)) ≥((n−2)/(n+3)) n^2 +3n≥n^2 +4n−12 n≤12 that means till n=12, P_n ≥P_(n−1) and upon n=13, P_n <P_(n−1) . so P_(12) is the maximum. P_(12) =((18×12×11)/(18×17×16))=((33)/(68))

$${usually}\:{it}\:{is}\:{a}\:{quick}\:{way}\:{to}\:{determine} \\ $$$${P}_{{n},\:{max}\:} \:{using}\:{graph}\:{calculator}. \\ $$$${but}\:{really}\:{solving}\:{f}'\left({n}\right)=\mathrm{0}\:{is}\:{not}\:{a} \\ $$$${good}\:{way},\:{even}\:{impossible}\:{like}\:{above}. \\ $$$${besides},\:{in}\:{an}\:{examination}\:{it}\:{is}\:{not}\: \\ $$$${allowed}\:{to}\:{use}\:{graph}\:{calculator}. \\ $$$$ \\ $$$${the}\:{best}\:{and}\:{yet}\:{easy}\:{and}\:{exact}\:{way}\: \\ $$$${is}\:{the}\:{following}: \\ $$$${we}\:{only}\:{need}\:{to}\:{find}\:{the}\:{n}_{{max}} \:{such} \\ $$$${that}\:{P}_{{n}} \geqslant{P}_{{n}−\mathrm{1}} ,\:{i}.{e}. \\ $$$$\:\frac{\mathrm{18}{n}\left({n}−\mathrm{1}\right)}{\left({n}+\mathrm{6}\right)\left({n}+\mathrm{5}\right)\left({n}+\mathrm{4}\right)}\:\geqslant\frac{\mathrm{18}\left({n}−\mathrm{1}\right)\left({n}−\mathrm{2}\right)}{\left({n}+\mathrm{5}\right)\left({n}+\mathrm{4}\right)\left({n}+\mathrm{3}\right)} \\ $$$$\:\Rightarrow\frac{{n}}{{n}+\mathrm{6}}\:\geqslant\frac{{n}−\mathrm{2}}{{n}+\mathrm{3}} \\ $$$${n}^{\mathrm{2}} +\mathrm{3}{n}\geqslant{n}^{\mathrm{2}} +\mathrm{4}{n}−\mathrm{12} \\ $$$${n}\leqslant\mathrm{12} \\ $$$${that}\:{means}\:{till}\:{n}=\mathrm{12},\:{P}_{{n}} \geqslant{P}_{{n}−\mathrm{1}} \\ $$$${and}\:{upon}\:{n}=\mathrm{13},\:{P}_{{n}} <{P}_{{n}−\mathrm{1}} . \\ $$$${so}\:{P}_{\mathrm{12}} \:{is}\:{the}\:{maximum}. \\ $$$$\:{P}_{\mathrm{12}} =\frac{\mathrm{18}×\mathrm{12}×\mathrm{11}}{\mathrm{18}×\mathrm{17}×\mathrm{16}}=\frac{\mathrm{33}}{\mathrm{68}} \\ $$

Commented by Tawa11 last updated on 11/Oct/22

Great sirs

$$\mathrm{Great}\:\mathrm{sirs} \\ $$

Commented by Acem last updated on 11/Oct/22

The P_(n_max) ≥ P_(n−1) is a genius idea!! Big Thanks Note: In order to avoid the student from calculating the cases in which n less than 12, in parallel with P_(n_max) ≥ P_(n−1) we add beside another logical inequality: P_(n_max) ≥ P_(n+1) & P_(n_max) ≥ P_(n−1) ((18n(n−1))/((n+6)(n+5)(n+4))) ≥ ((18(n+1).n)/((n+7)(n+6)(n+5))) ((n−1)/(n+4)) ≥ ((n+1)/(n+7)) ⇒ n≥ 11 Then we have n≤ 12 & n≥ 11 i.e. n_1 = 11, n_2 =12 both achieve to be P_(11or 12_max) P_(11 or 12−max) = ((33)/(68)) My greetings

$$\:{The}\:{P}_{{n\_max}} \:\geqslant\:{P}_{{n}−\mathrm{1}} \:{is}\:{a}\:\boldsymbol{{genius}}\:\boldsymbol{{idea}}!! \\ $$$$\:{Big}\:{Thanks} \\ $$$$ \\ $$$$\:\boldsymbol{{Note}}:\:{In}\:{order}\:{to}\:{avoid}\:{the}\:{student}\:{from} \\ $$$$\:\:\:{calculating}\:{the}\:{cases}\:{in}\:{which}\:{n}\:{less} \\ $$$$\:\:\:{than}\:\mathrm{12},\:{in}\:{parallel}\:{with}\:{P}_{{n\_max}} \:\geqslant\:{P}_{{n}−\mathrm{1}} \\ $$$$\:\:\:{we}\:{add}\:{beside}\:{another}\:{logical}\:{inequality}: \\ $$$$\:\boldsymbol{{P}}_{\boldsymbol{{n\_max}}} \:\geqslant\:\boldsymbol{{P}}_{\boldsymbol{{n}}+\mathrm{1}} \:\:\&\:{P}_{{n\_max}} \:\geqslant\:{P}_{{n}−\mathrm{1}} \: \\ $$$$ \\ $$$$\:\frac{\mathrm{18}{n}\left({n}−\mathrm{1}\right)}{\left({n}+\mathrm{6}\right)\left({n}+\mathrm{5}\right)\left({n}+\mathrm{4}\right)}\:\geqslant\:\frac{\mathrm{18}\left({n}+\mathrm{1}\right).{n}}{\left({n}+\mathrm{7}\right)\left({n}+\mathrm{6}\right)\left({n}+\mathrm{5}\right)} \\ $$$$ \\ $$$$\:\:\:\:\:\:\:\frac{{n}−\mathrm{1}}{{n}+\mathrm{4}}\:\geqslant\:\frac{{n}+\mathrm{1}}{{n}+\mathrm{7}}\:\Rightarrow\:{n}\geqslant\:\mathrm{11} \\ $$$$ \\ $$$$\:{Then}\:{we}\:{have}\:\:{n}\leqslant\:\mathrm{12}\:\&\:{n}\geqslant\:\mathrm{11}\:{i}.{e}. \\ $$$$ \\ $$$$\:{n}_{\mathrm{1}} =\:\mathrm{11},\:{n}_{\mathrm{2}} =\mathrm{12}\:{both}\:{achieve}\:{to}\:{be}\:{P}_{\mathrm{11}{or}\:\mathrm{12\_}{max}} \\ $$$$\:{P}_{\mathrm{11}\:{or}\:\mathrm{12}−{max}} =\:\frac{\mathrm{33}}{\mathrm{68}} \\ $$$$ \\ $$$$\:{My}\:{greetings} \\ $$

Commented by Acem last updated on 11/Oct/22

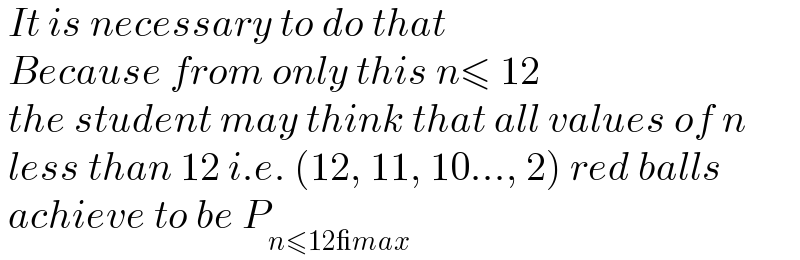
It is necessary to do that Because from only this n≤ 12 the student may think that all values of n less than 12 i.e. (12, 11, 10..., 2) red balls achieve to be P_(n≤12_max)

$$\:{It}\:{is}\:{necessary}\:{to}\:{do}\:{that} \\ $$$$\:{Because}\:{from}\:{only}\:{this}\:{n}\leqslant\:\mathrm{12} \\ $$$$\:{the}\:{student}\:{may}\:{think}\:{that}\:{all}\:{values}\:{of}\:{n} \\ $$$$\:{less}\:{than}\:\mathrm{12}\:{i}.{e}.\:\left(\mathrm{12},\:\mathrm{11},\:\mathrm{10}...,\:\mathrm{2}\right)\:{red}\:{balls} \\ $$$$\:{achieve}\:{to}\:{be}\:{P}_{{n}\leqslant\mathrm{12\_}{max}} \\ $$

Commented by mr W last updated on 11/Oct/22

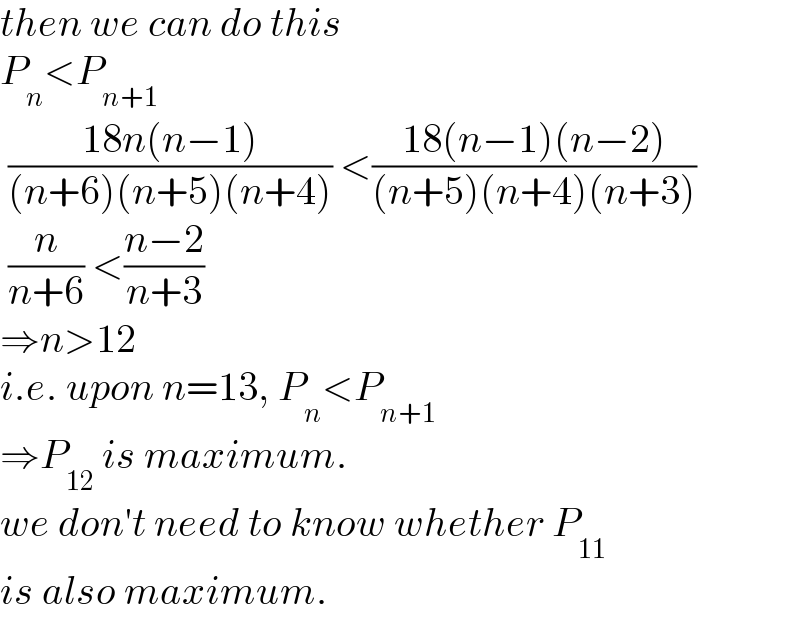
then we can do this P_n <P_(n+1) ((18n(n−1))/((n+6)(n+5)(n+4))) <((18(n−1)(n−2))/((n+5)(n+4)(n+3))) (n/(n+6)) <((n−2)/(n+3)) ⇒n>12 i.e. upon n=13, P_n <P_(n+1) ⇒P_(12) is maximum. we don′t need to know whether P_(11) is also maximum.

$${then}\:{we}\:{can}\:{do}\:{this} \\ $$$${P}_{{n}} <{P}_{{n}+\mathrm{1}} \\ $$$$\:\frac{\mathrm{18}{n}\left({n}−\mathrm{1}\right)}{\left({n}+\mathrm{6}\right)\left({n}+\mathrm{5}\right)\left({n}+\mathrm{4}\right)}\:<\frac{\mathrm{18}\left({n}−\mathrm{1}\right)\left({n}−\mathrm{2}\right)}{\left({n}+\mathrm{5}\right)\left({n}+\mathrm{4}\right)\left({n}+\mathrm{3}\right)} \\ $$$$\:\frac{{n}}{{n}+\mathrm{6}}\:<\frac{{n}−\mathrm{2}}{{n}+\mathrm{3}} \\ $$$$\Rightarrow{n}>\mathrm{12} \\ $$$${i}.{e}.\:{upon}\:{n}=\mathrm{13},\:{P}_{{n}} <{P}_{{n}+\mathrm{1}} \\ $$$$\Rightarrow{P}_{\mathrm{12}} \:{is}\:{maximum}. \\ $$$${we}\:{don}'{t}\:{need}\:{to}\:{know}\:{whether}\:{P}_{\mathrm{11}} \\ $$$${is}\:{also}\:{maximum}. \\ $$

Commented by Acem last updated on 12/Oct/22

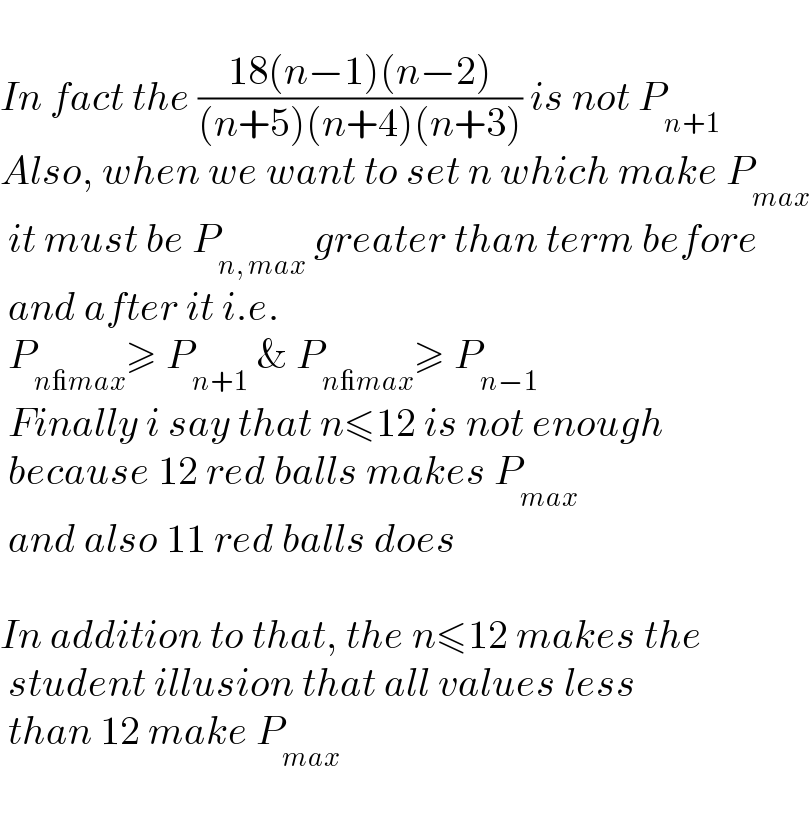
In fact the ((18(n−1)(n−2))/((n+5)(n+4)(n+3))) is not P_(n+1) Also, when we want to set n which make P_(max) it must be P_(n, max) greater than term before and after it i.e. P_(n_max) ≥ P_(n+1) & P_(n_max) ≥ P_(n−1) Finally i say that n≤12 is not enough because 12 red balls makes P_(max) and also 11 red balls does In addition to that, the n≤12 makes the student illusion that all values less than 12 make P_(max)

$$ \\ $$$${In}\:{fact}\:{the}\:\frac{\mathrm{18}\left({n}−\mathrm{1}\right)\left({n}−\mathrm{2}\right)}{\left({n}+\mathrm{5}\right)\left({n}+\mathrm{4}\right)\left({n}+\mathrm{3}\right)}\:{is}\:{not}\:{P}_{{n}+\mathrm{1}} \\ $$$${Also},\:{when}\:{we}\:{want}\:{to}\:{set}\:{n}\:{which}\:{make}\:{P}_{{max}} \\ $$$$\:{it}\:{must}\:{be}\:{P}_{{n},\:{max}} \:{greater}\:{than}\:{term}\:{before} \\ $$$$\:{and}\:{after}\:{it}\:{i}.{e}.\: \\ $$$$\:{P}_{{n\_max}} \geqslant\:{P}_{{n}+\mathrm{1}} \:\&\:{P}_{{n\_max}} \geqslant\:{P}_{{n}−\mathrm{1}} \\ $$$$\:{Finally}\:{i}\:{say}\:{that}\:{n}\leqslant\mathrm{12}\:{is}\:{not}\:{enough} \\ $$$$\:{because}\:\mathrm{12}\:{red}\:{balls}\:{makes}\:{P}_{{max}} \\ $$$$\:{and}\:{also}\:\mathrm{11}\:{red}\:{balls}\:{does} \\ $$$$ \\ $$$${In}\:{addition}\:{to}\:{that},\:{the}\:{n}\leqslant\mathrm{12}\:{makes}\:{the} \\ $$$$\:{student}\:{illusion}\:{that}\:{all}\:{values}\:{less} \\ $$$$\:{than}\:\mathrm{12}\:{make}\:{P}_{{max}} \\ $$$$\: \\ $$

Commented by mr W last updated on 12/Oct/22

as i said when we only need to get P_(n,max) it′s enough to know that P_(12) the maximum. we don′t need to care whether P_(11) is also maximum. from P_1 ≤P_2 ≤P_3 ≤....≤P_(11) ≤P_(12) >P_(13) >P_(14) >... we can be sure that P_(12) is the maximum. it is not said that only P_(12) is the maximum, but this is for the question not important.

$${as}\:{i}\:{said}\:{when}\:{we}\:{only}\:{need}\:{to}\:{get}\:{P}_{{n},{max}} \\ $$$${it}'{s}\:{enough}\:{to}\:{know}\:{that}\:{P}_{\mathrm{12}} \:{the} \\ $$$${maximum}.\:{we}\:{don}'{t}\:{need}\:{to}\:{care} \\ $$$${whether}\:{P}_{\mathrm{11}} \:{is}\:{also}\:{maximum}. \\ $$$${from}\: \\ $$$${P}_{\mathrm{1}} \leqslant{P}_{\mathrm{2}} \leqslant{P}_{\mathrm{3}} \leqslant....\leqslant{P}_{\mathrm{11}} \leqslant{P}_{\mathrm{12}} >{P}_{\mathrm{13}} >{P}_{\mathrm{14}} >... \\ $$$${we}\:{can}\:{be}\:{sure}\:{that}\:{P}_{\mathrm{12}} \:{is}\:{the}\:{maximum}. \\ $$$${it}\:{is}\:{not}\:{said}\:{that}\:{only}\:{P}_{\mathrm{12}} \:{is}\:{the} \\ $$$${maximum},\:{but}\:{this}\:{is}\:{for}\:{the} \\ $$$${question}\:{not}\:{important}. \\ $$

Commented by Acem last updated on 13/Oct/22

It′s ok and good

$$\: \\ $$$${It}'{s}\:{ok}\:{and}\:{good} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com