
Question Number 176367 by mr W last updated on 17/Sep/22
Commented by mr W last updated on 17/Sep/22

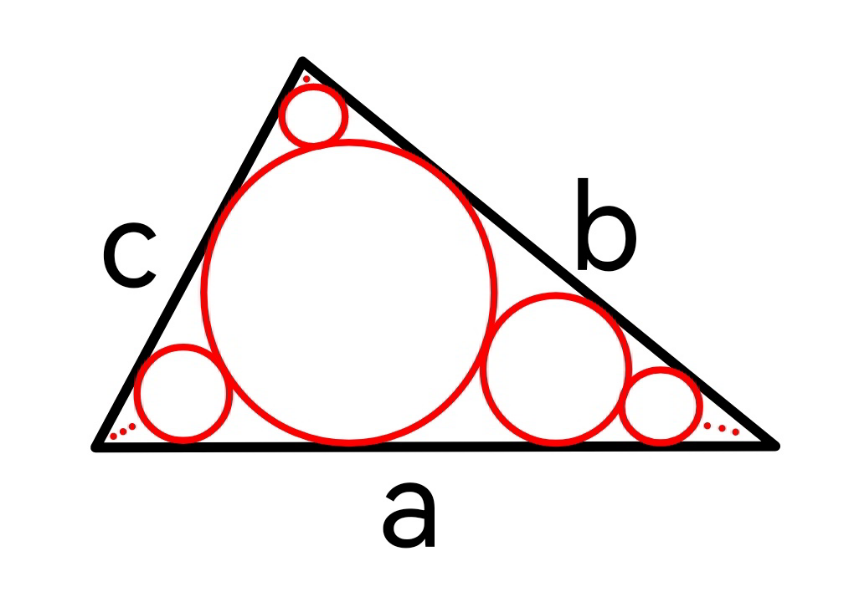
$${find}\:{the}\:{sum}\:{of}\:{areas}\:{of}\:{all}\:{inscribed} \\ $$$${circles}\:{in}\:{a}\:{triangle}\:{with}\:{side}\:{lengthes} \\ $$$${a},{b},{c}\:{as}\:{shown}. \\ $$
Answered by mr W last updated on 18/Sep/22
Commented by mr W last updated on 19/Sep/22
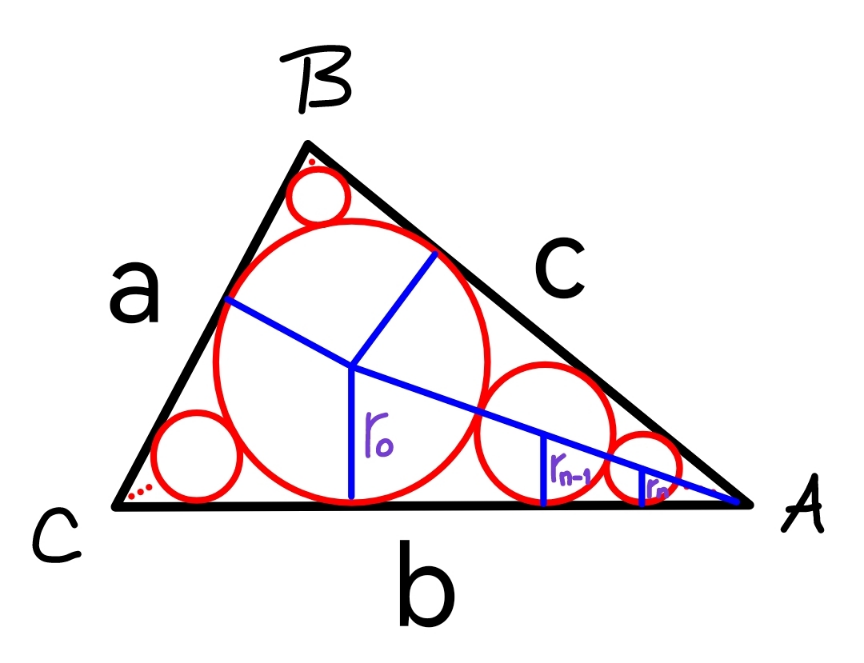
![let Δ=area of the triangle R=radius of circumcircle r_0 =radius of incircle Δ=((bc sin A)/2)=((abc sin A)/(2a))=((abc)/(4R)) r_0 =((2Δ)/(a+b+c)) (r_0 ^2 /Δ)=((4Δ)/((a+b+c)^2 ))=((abc)/(R(a+b+c)^2 )) (r_0 ^2 /Δ)=((2 sin A sin B sin C)/((sin A+sin B+sin C)^2 ))=tan (A/2) tan (B/2) tan (C/2) ((r_(n−1) −r_n )/(r_(n−1) +r_n ))=sin (A/2) (r_n /r_(n−1) )=((1−sin (A/2))/(1+sin (A/2))) (r_n ^2 /r_(n−1) ^2 )=(((1−sin (A/2))/(1+sin (A/2))))^2 =q_A <1 ⇒G.P. 1−q_A =(1+((1−sin (A/2))/(1+sin (A/2))))(1−((1−sin (A/2))/(1+sin (A/2)))) 1−q_A =((4 sin (A/2))/((1+sin (A/2))^2 )) (1/(1−q_A ))=(((1+sin (A/2))^2 )/(4 sin (A/2)))=(1/4)(sin (A/2)+(1/(sin (A/2))))+(1/2) A_n =πr_n ^2 Σ_(n=0) ^∞ A_n =πΣ_(n=0) ^∞ r_n ^2 =((πr_0 ^2 )/(1−q_a )) sum of areas of all inscribed circles: A_(i.c.) =((πr_0 ^2 )/(1−q_A ))+((πr_0 ^2 )/(1−q_B ))+((πr_0 ^2 )/(1−q_C ))−2πr_0 ^2 A_(i.c.) =((1/(1−q_A ))+(1/(1−q_B ))+(1/(1−q_C ))−2)πr_0 ^2 A_(i.c.) =[(1/4)(sin (A/2)+sin (B/2)+sin (C/2)+(1/(sin (A/2)))+(1/(sin (B/2)))+(1/(sin (C/2))))+(3/2)−2]πr_0 ^2 A_(i.c.) =(sin (A/2)+sin (B/2)+sin (C/2)+(1/(sin (A/2)))+(1/(sin (B/2)))+(1/(sin (C/2)))−2)((πr_0 ^2 )/4) (A_(i.c.) /Δ)=(sin (A/2)+sin (B/2)+sin (C/2)+(1/(sin (A/2)))+(1/(sin (B/2)))+(1/(sin (C/2)))−2)((πr_0 ^2 )/(4Δ)) (A_(i.c.) /Δ)=(π/4) tan (A/2) tan (B/2) tan (C/2)(sin (A/2)+sin (B/2)+sin (C/2)+(1/(sin (A/2)))+(1/(sin (B/2)))+(1/(sin (C/2)))−2) examples: A=40°, B=60°, C=80°: (A_(i.c.) /Δ)≈0.826 A=30°, B=40°, C=110°: (A_(i.c.) /Δ)≈0.812 A=60°, B=60°, C=60°: (A_(i.c.) /Δ)=((11(√3)π)/(72))≈0.831](Q176398.png)
$${let}\:\Delta={area}\:{of}\:{the}\:{triangle} \\ $$$${R}={radius}\:{of}\:{circumcircle} \\ $$$${r}_{\mathrm{0}} ={radius}\:{of}\:{incircle} \\ $$$$\Delta=\frac{{bc}\:\mathrm{sin}\:{A}}{\mathrm{2}}=\frac{{abc}\:\mathrm{sin}\:{A}}{\mathrm{2}{a}}=\frac{{abc}}{\mathrm{4}{R}} \\ $$$${r}_{\mathrm{0}} =\frac{\mathrm{2}\Delta}{{a}+{b}+{c}} \\ $$$$\frac{{r}_{\mathrm{0}} ^{\mathrm{2}} }{\Delta}=\frac{\mathrm{4}\Delta}{\left({a}+{b}+{c}\right)^{\mathrm{2}} }=\frac{{abc}}{{R}\left({a}+{b}+{c}\right)^{\mathrm{2}} } \\ $$$$\frac{{r}_{\mathrm{0}} ^{\mathrm{2}} }{\Delta}=\frac{\mathrm{2}\:\mathrm{sin}\:{A}\:\mathrm{sin}\:{B}\:\mathrm{sin}\:{C}}{\left(\mathrm{sin}\:{A}+\mathrm{sin}\:{B}+\mathrm{sin}\:{C}\right)^{\mathrm{2}} }=\mathrm{tan}\:\frac{{A}}{\mathrm{2}}\:\mathrm{tan}\:\frac{{B}}{\mathrm{2}}\:\mathrm{tan}\:\frac{{C}}{\mathrm{2}} \\ $$$$ \\ $$$$\frac{{r}_{{n}−\mathrm{1}} −{r}_{{n}} }{{r}_{{n}−\mathrm{1}} +{r}_{{n}} }=\mathrm{sin}\:\frac{{A}}{\mathrm{2}} \\ $$$$\frac{{r}_{{n}} }{{r}_{{n}−\mathrm{1}} }=\frac{\mathrm{1}−\mathrm{sin}\:\frac{{A}}{\mathrm{2}}}{\mathrm{1}+\mathrm{sin}\:\frac{{A}}{\mathrm{2}}} \\ $$$$\frac{{r}_{{n}} ^{\mathrm{2}} }{{r}_{{n}−\mathrm{1}} ^{\mathrm{2}} }=\left(\frac{\mathrm{1}−\mathrm{sin}\:\frac{{A}}{\mathrm{2}}}{\mathrm{1}+\mathrm{sin}\:\frac{{A}}{\mathrm{2}}}\right)^{\mathrm{2}} ={q}_{{A}} <\mathrm{1}\:\Rightarrow{G}.{P}. \\ $$$$\mathrm{1}−{q}_{{A}} =\left(\mathrm{1}+\frac{\mathrm{1}−\mathrm{sin}\:\frac{{A}}{\mathrm{2}}}{\mathrm{1}+\mathrm{sin}\:\frac{{A}}{\mathrm{2}}}\right)\left(\mathrm{1}−\frac{\mathrm{1}−\mathrm{sin}\:\frac{{A}}{\mathrm{2}}}{\mathrm{1}+\mathrm{sin}\:\frac{{A}}{\mathrm{2}}}\right) \\ $$$$\mathrm{1}−{q}_{{A}} =\frac{\mathrm{4}\:\mathrm{sin}\:\frac{{A}}{\mathrm{2}}}{\left(\mathrm{1}+\mathrm{sin}\:\frac{{A}}{\mathrm{2}}\right)^{\mathrm{2}} } \\ $$$$\frac{\mathrm{1}}{\mathrm{1}−{q}_{{A}} }=\frac{\left(\mathrm{1}+\mathrm{sin}\:\frac{{A}}{\mathrm{2}}\right)^{\mathrm{2}} }{\mathrm{4}\:\mathrm{sin}\:\frac{{A}}{\mathrm{2}}}=\frac{\mathrm{1}}{\mathrm{4}}\left(\mathrm{sin}\:\frac{{A}}{\mathrm{2}}+\frac{\mathrm{1}}{\mathrm{sin}\:\frac{{A}}{\mathrm{2}}}\right)+\frac{\mathrm{1}}{\mathrm{2}} \\ $$$$ \\ $$$${A}_{{n}} =\pi{r}_{{n}} ^{\mathrm{2}} \\ $$$$\underset{{n}=\mathrm{0}} {\overset{\infty} {\sum}}{A}_{{n}} =\pi\underset{{n}=\mathrm{0}} {\overset{\infty} {\sum}}{r}_{{n}} ^{\mathrm{2}} =\frac{\pi{r}_{\mathrm{0}} ^{\mathrm{2}} }{\mathrm{1}−{q}_{{a}} } \\ $$$${sum}\:{of}\:{areas}\:{of}\:{all}\:{inscribed}\:{circles}: \\ $$$${A}_{{i}.{c}.} =\frac{\pi{r}_{\mathrm{0}} ^{\mathrm{2}} }{\mathrm{1}−{q}_{{A}} }+\frac{\pi{r}_{\mathrm{0}} ^{\mathrm{2}} }{\mathrm{1}−{q}_{{B}} }+\frac{\pi{r}_{\mathrm{0}} ^{\mathrm{2}} }{\mathrm{1}−{q}_{{C}} }−\mathrm{2}\pi{r}_{\mathrm{0}} ^{\mathrm{2}} \\ $$$${A}_{{i}.{c}.} =\left(\frac{\mathrm{1}}{\mathrm{1}−{q}_{{A}} }+\frac{\mathrm{1}}{\mathrm{1}−{q}_{{B}} }+\frac{\mathrm{1}}{\mathrm{1}−{q}_{{C}} }−\mathrm{2}\right)\pi{r}_{\mathrm{0}} ^{\mathrm{2}} \\ $$$${A}_{{i}.{c}.} =\left[\frac{\mathrm{1}}{\mathrm{4}}\left(\mathrm{sin}\:\frac{{A}}{\mathrm{2}}+\mathrm{sin}\:\frac{{B}}{\mathrm{2}}+\mathrm{sin}\:\frac{{C}}{\mathrm{2}}+\frac{\mathrm{1}}{\mathrm{sin}\:\frac{{A}}{\mathrm{2}}}+\frac{\mathrm{1}}{\mathrm{sin}\:\frac{{B}}{\mathrm{2}}}+\frac{\mathrm{1}}{\mathrm{sin}\:\frac{{C}}{\mathrm{2}}}\right)+\frac{\mathrm{3}}{\mathrm{2}}−\mathrm{2}\right]\pi{r}_{\mathrm{0}} ^{\mathrm{2}} \\ $$$${A}_{{i}.{c}.} =\left(\mathrm{sin}\:\frac{{A}}{\mathrm{2}}+\mathrm{sin}\:\frac{{B}}{\mathrm{2}}+\mathrm{sin}\:\frac{{C}}{\mathrm{2}}+\frac{\mathrm{1}}{\mathrm{sin}\:\frac{{A}}{\mathrm{2}}}+\frac{\mathrm{1}}{\mathrm{sin}\:\frac{{B}}{\mathrm{2}}}+\frac{\mathrm{1}}{\mathrm{sin}\:\frac{{C}}{\mathrm{2}}}−\mathrm{2}\right)\frac{\pi{r}_{\mathrm{0}} ^{\mathrm{2}} }{\mathrm{4}} \\ $$$$\frac{{A}_{{i}.{c}.} }{\Delta}=\left(\mathrm{sin}\:\frac{{A}}{\mathrm{2}}+\mathrm{sin}\:\frac{{B}}{\mathrm{2}}+\mathrm{sin}\:\frac{{C}}{\mathrm{2}}+\frac{\mathrm{1}}{\mathrm{sin}\:\frac{{A}}{\mathrm{2}}}+\frac{\mathrm{1}}{\mathrm{sin}\:\frac{{B}}{\mathrm{2}}}+\frac{\mathrm{1}}{\mathrm{sin}\:\frac{{C}}{\mathrm{2}}}−\mathrm{2}\right)\frac{\pi{r}_{\mathrm{0}} ^{\mathrm{2}} }{\mathrm{4}\Delta} \\ $$$$\frac{{A}_{{i}.{c}.} }{\Delta}=\frac{\pi}{\mathrm{4}}\:\mathrm{tan}\:\frac{{A}}{\mathrm{2}}\:\mathrm{tan}\:\frac{{B}}{\mathrm{2}}\:\mathrm{tan}\:\frac{{C}}{\mathrm{2}}\left(\mathrm{sin}\:\frac{{A}}{\mathrm{2}}+\mathrm{sin}\:\frac{{B}}{\mathrm{2}}+\mathrm{sin}\:\frac{{C}}{\mathrm{2}}+\frac{\mathrm{1}}{\mathrm{sin}\:\frac{{A}}{\mathrm{2}}}+\frac{\mathrm{1}}{\mathrm{sin}\:\frac{{B}}{\mathrm{2}}}+\frac{\mathrm{1}}{\mathrm{sin}\:\frac{{C}}{\mathrm{2}}}−\mathrm{2}\right) \\ $$$$ \\ $$$${examples}: \\ $$$${A}=\mathrm{40}°,\:{B}=\mathrm{60}°,\:{C}=\mathrm{80}°:\:\frac{{A}_{{i}.{c}.} }{\Delta}\approx\mathrm{0}.\mathrm{826} \\ $$$${A}=\mathrm{30}°,\:{B}=\mathrm{40}°,\:{C}=\mathrm{110}°:\:\frac{{A}_{{i}.{c}.} }{\Delta}\approx\mathrm{0}.\mathrm{812} \\ $$$${A}=\mathrm{60}°,\:{B}=\mathrm{60}°,\:{C}=\mathrm{60}°:\:\frac{{A}_{{i}.{c}.} }{\Delta}=\frac{\mathrm{11}\sqrt{\mathrm{3}}\pi}{\mathrm{72}}\approx\mathrm{0}.\mathrm{831} \\ $$
Commented by Tawa11 last updated on 18/Sep/22

$$\mathrm{Wow},\:\mathrm{great}\:\mathrm{sir}. \\ $$
Commented by behi834171 last updated on 18/Sep/22
$${superb}! \\ $$$${fantastic}! \\ $$$${kudos}\:{to}\:{you}\:{prop}:\:\boldsymbol{{mrW}}! \\ $$
