Question and Answers Forum

All Questions      Topic List

None Questions

Previous in All Question      Next in All Question      

Previous in None      Next in None      

Question Number 176292 by mokys last updated on 15/Sep/22

Answered by floor(10²Eta[1]) last updated on 16/Sep/22

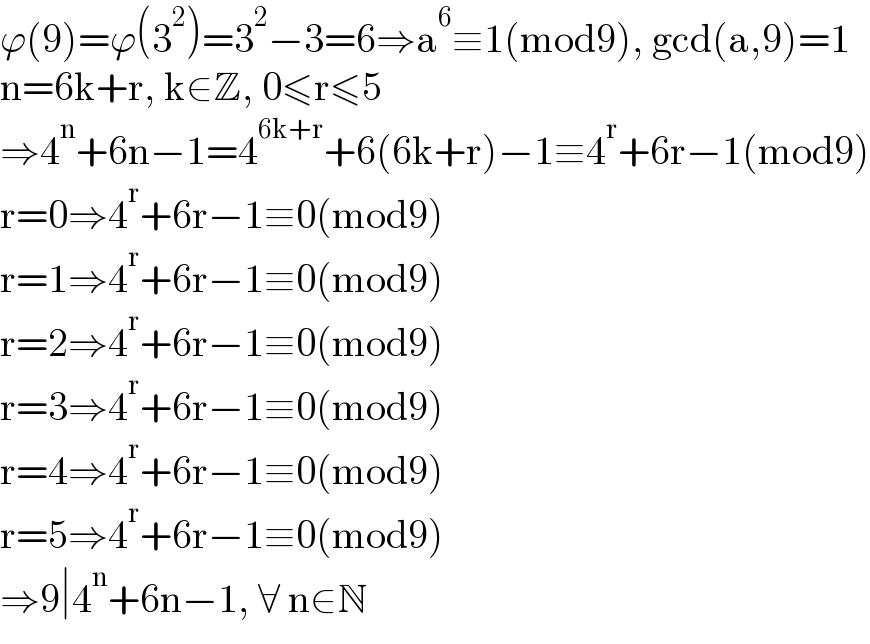
ϕ(9)=ϕ(3^2 )=3^2 −3=6⇒a^6 ≡1(mod9), gcd(a,9)=1 n=6k+r, k∈Z, 0≤r≤5 ⇒4^n +6n−1=4^(6k+r) +6(6k+r)−1≡4^r +6r−1(mod9) r=0⇒4^r +6r−1≡0(mod9) r=1⇒4^r +6r−1≡0(mod9) r=2⇒4^r +6r−1≡0(mod9) r=3⇒4^r +6r−1≡0(mod9) r=4⇒4^r +6r−1≡0(mod9) r=5⇒4^r +6r−1≡0(mod9) ⇒9∣4^n +6n−1, ∀ n∈N

$$\varphi\left(\mathrm{9}\right)=\varphi\left(\mathrm{3}^{\mathrm{2}} \right)=\mathrm{3}^{\mathrm{2}} −\mathrm{3}=\mathrm{6}\Rightarrow\mathrm{a}^{\mathrm{6}} \equiv\mathrm{1}\left(\mathrm{mod9}\right),\:\mathrm{gcd}\left(\mathrm{a},\mathrm{9}\right)=\mathrm{1} \\ $$$$\mathrm{n}=\mathrm{6k}+\mathrm{r},\:\mathrm{k}\in\mathbb{Z},\:\mathrm{0}\leqslant\mathrm{r}\leqslant\mathrm{5} \\ $$$$\Rightarrow\mathrm{4}^{\mathrm{n}} +\mathrm{6n}−\mathrm{1}=\mathrm{4}^{\mathrm{6k}+\mathrm{r}} +\mathrm{6}\left(\mathrm{6k}+\mathrm{r}\right)−\mathrm{1}\equiv\mathrm{4}^{\mathrm{r}} +\mathrm{6r}−\mathrm{1}\left(\mathrm{mod9}\right) \\ $$$$\mathrm{r}=\mathrm{0}\Rightarrow\mathrm{4}^{\mathrm{r}} +\mathrm{6r}−\mathrm{1}\equiv\mathrm{0}\left(\mathrm{mod9}\right) \\ $$$$\mathrm{r}=\mathrm{1}\Rightarrow\mathrm{4}^{\mathrm{r}} +\mathrm{6r}−\mathrm{1}\equiv\mathrm{0}\left(\mathrm{mod9}\right) \\ $$$$\mathrm{r}=\mathrm{2}\Rightarrow\mathrm{4}^{\mathrm{r}} +\mathrm{6r}−\mathrm{1}\equiv\mathrm{0}\left(\mathrm{mod9}\right) \\ $$$$\mathrm{r}=\mathrm{3}\Rightarrow\mathrm{4}^{\mathrm{r}} +\mathrm{6r}−\mathrm{1}\equiv\mathrm{0}\left(\mathrm{mod9}\right) \\ $$$$\mathrm{r}=\mathrm{4}\Rightarrow\mathrm{4}^{\mathrm{r}} +\mathrm{6r}−\mathrm{1}\equiv\mathrm{0}\left(\mathrm{mod9}\right) \\ $$$$\mathrm{r}=\mathrm{5}\Rightarrow\mathrm{4}^{\mathrm{r}} +\mathrm{6r}−\mathrm{1}\equiv\mathrm{0}\left(\mathrm{mod9}\right) \\ $$$$\Rightarrow\mathrm{9}\mid\mathrm{4}^{\mathrm{n}} +\mathrm{6n}−\mathrm{1},\:\forall\:\mathrm{n}\in\mathbb{N} \\ $$

Answered by a.lgnaoui last updated on 16/Sep/22

n=1 4^1 +6−1=9 n=2 4^2 +12−1=27=3×9 n=3 4^3 +18−1=81=9^2 n=4 4^4 +24−1=279=31×9 ....................................... n+1 4^(n+1) +6(n+1)−1=4^(n+1) +6n+5 4[4^n +6n−1]+(9−18n) =4(4^n +6n−1)+9(1−2n) n∈N^∗ Somme de 2 nombres divisibles par 9 donc divisible ar 9

$${n}=\mathrm{1}\:\:\:\:\:\:\mathrm{4}^{\mathrm{1}} +\mathrm{6}−\mathrm{1}=\mathrm{9} \\ $$$${n}=\mathrm{2}\:\:\:\:\:\:\mathrm{4}^{\mathrm{2}} +\mathrm{12}−\mathrm{1}=\mathrm{27}=\mathrm{3}×\mathrm{9} \\ $$$${n}=\mathrm{3}\:\:\:\:\:\:\:\mathrm{4}^{\mathrm{3}} +\mathrm{18}−\mathrm{1}=\mathrm{81}=\mathrm{9}^{\mathrm{2}} \: \\ $$$${n}=\mathrm{4}\:\:\:\:\:\:\:\:\mathrm{4}^{\mathrm{4}} +\mathrm{24}−\mathrm{1}=\mathrm{279}=\mathrm{31}×\mathrm{9} \\ $$$$....................................... \\ $$$${n}+\mathrm{1}\:\:\:\:\:\:\:\:\:\mathrm{4}^{{n}+\mathrm{1}} +\mathrm{6}\left({n}+\mathrm{1}\right)−\mathrm{1}=\mathrm{4}^{{n}+\mathrm{1}} +\mathrm{6}{n}+\mathrm{5} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{4}\left[\mathrm{4}^{{n}} +\mathrm{6}{n}−\mathrm{1}\right]+\left(\mathrm{9}−\mathrm{18}{n}\right) \\ $$$$\:=\mathrm{4}\left(\mathrm{4}^{{n}} \:+\mathrm{6}{n}−\mathrm{1}\right)+\mathrm{9}\left(\mathrm{1}−\mathrm{2}{n}\right)\:\:\:\:{n}\in\mathbb{N}^{\ast} \:\:\:\:\:\:\:\:\:\: \\ $$$${Somme}\:{de}\:\mathrm{2}\:{nombres}\:{divisibles}\:{par}\:\mathrm{9}\:\:\: \\ $$$${donc}\:\:\:\:{divisible}\:{ar}\:\mathrm{9} \\ $$$$ \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com