
Question Number 175571 by mnjuly1970 last updated on 02/Sep/22

Answered by behi834171 last updated on 03/Sep/22
![cos(A/2)=(√((p(p−a))/(bc))) and so on... ((a(b+c))/(bccos^2 (A/2)))=((a(b+c))/(p(p−a))) and so on.... ⇒lhs=((a(b+c))/(p(p−a)))+((b(c+a))/(p(p−b)))+((c(a+b))/(p(p−c)))= ≥(((abc(a+b)(b+c)(c+a))/(p^2 S^2 )))^(1/3) ≥ ≥(((abc×2(√(ab)).2(√(ac)).2(√(cb)))/(p^2 S^2 )))^(1/3) = =2((((abc)^2 )/(p^2 S^2 )))^(1/3) =2(((16R^2 S^2 )/(p^2 S^2 )))^(1/3) =2(((16R^2 )/p^2 ))^(1/3) ≥ ≥2((16×4))^(1/3) =8 .■ [p=((a+b+c)/2)=((2R(ΣsinA))/2)≥R×((3(√3))/2)⇒ ⇒p^2 ≥((27)/4)R^2 ⇒(R^2 /p^2 )≤(4/(27))<4]](Q175576.png)
$$\boldsymbol{{cos}}\frac{\boldsymbol{{A}}}{\mathrm{2}}=\sqrt{\frac{\boldsymbol{{p}}\left(\boldsymbol{{p}}−\boldsymbol{{a}}\right)}{\boldsymbol{{bc}}}}\:\:\boldsymbol{{and}}\:\boldsymbol{{so}}\:\boldsymbol{{on}}... \\ $$$$\frac{\boldsymbol{{a}}\left(\boldsymbol{{b}}+\boldsymbol{{c}}\right)}{\boldsymbol{{bccos}}^{\mathrm{2}} \frac{\boldsymbol{{A}}}{\mathrm{2}}}=\frac{\boldsymbol{{a}}\left(\boldsymbol{{b}}+\boldsymbol{{c}}\right)}{\boldsymbol{{p}}\left(\boldsymbol{{p}}−\boldsymbol{{a}}\right)}\:\:\:\boldsymbol{{and}}\:\boldsymbol{{so}}\:\boldsymbol{{on}}.... \\ $$$$\Rightarrow{lhs}=\frac{\boldsymbol{{a}}\left(\boldsymbol{{b}}+\boldsymbol{{c}}\right)}{\boldsymbol{{p}}\left(\boldsymbol{{p}}−\boldsymbol{{a}}\right)}+\frac{\boldsymbol{{b}}\left(\boldsymbol{{c}}+\boldsymbol{{a}}\right)}{\boldsymbol{{p}}\left(\boldsymbol{{p}}−\boldsymbol{{b}}\right)}+\frac{\boldsymbol{{c}}\left(\boldsymbol{{a}}+\boldsymbol{{b}}\right)}{\boldsymbol{{p}}\left(\boldsymbol{{p}}−\boldsymbol{{c}}\right)}= \\ $$$$\geqslant\sqrt[{\mathrm{3}}]{\frac{\boldsymbol{{abc}}\left(\boldsymbol{{a}}+\boldsymbol{{b}}\right)\left(\boldsymbol{{b}}+\boldsymbol{{c}}\right)\left(\boldsymbol{{c}}+\boldsymbol{{a}}\right)}{\boldsymbol{{p}}^{\mathrm{2}} \boldsymbol{{S}}^{\mathrm{2}} }}\geqslant \\ $$$$\geqslant\sqrt[{\mathrm{3}}]{\frac{\boldsymbol{{abc}}×\mathrm{2}\sqrt{\boldsymbol{{ab}}}.\mathrm{2}\sqrt{\boldsymbol{{ac}}}.\mathrm{2}\sqrt{\boldsymbol{{c}}{b}}}{\boldsymbol{{p}}^{\mathrm{2}} \boldsymbol{{S}}^{\mathrm{2}} }}= \\ $$$$=\mathrm{2}\sqrt[{\mathrm{3}}]{\frac{\left(\boldsymbol{{abc}}\right)^{\mathrm{2}} }{\boldsymbol{{p}}^{\mathrm{2}} \boldsymbol{{S}}^{\mathrm{2}} }}=\mathrm{2}\sqrt[{\mathrm{3}}]{\frac{\mathrm{16}{R}^{\mathrm{2}} {S}^{\mathrm{2}} }{\boldsymbol{{p}}^{\mathrm{2}} \boldsymbol{{S}}^{\mathrm{2}} }}=\mathrm{2}\sqrt[{\mathrm{3}}]{\frac{\mathrm{16}{R}^{\mathrm{2}} }{\boldsymbol{{p}}^{\mathrm{2}} }}\geqslant \\ $$$$\geqslant\mathrm{2}\sqrt[{\mathrm{3}}]{\mathrm{16}×\mathrm{4}}=\mathrm{8}\:\:\:.\blacksquare \\ $$$$ \\ $$$$\left[{p}=\frac{{a}+{b}+{c}}{\mathrm{2}}=\frac{\mathrm{2}{R}\left(\Sigma{sinA}\right)}{\mathrm{2}}\geqslant{R}×\frac{\mathrm{3}\sqrt{\mathrm{3}}}{\mathrm{2}}\Rightarrow\right. \\ $$$$\left.\Rightarrow\boldsymbol{{p}}^{\mathrm{2}} \geqslant\frac{\mathrm{27}}{\mathrm{4}}{R}^{\mathrm{2}} \Rightarrow\frac{{R}^{\mathrm{2}} }{\boldsymbol{{p}}^{\mathrm{2}} }\leqslant\frac{\mathrm{4}}{\mathrm{27}}<\mathrm{4}\right] \\ $$
Answered by mahdipoor last updated on 03/Sep/22
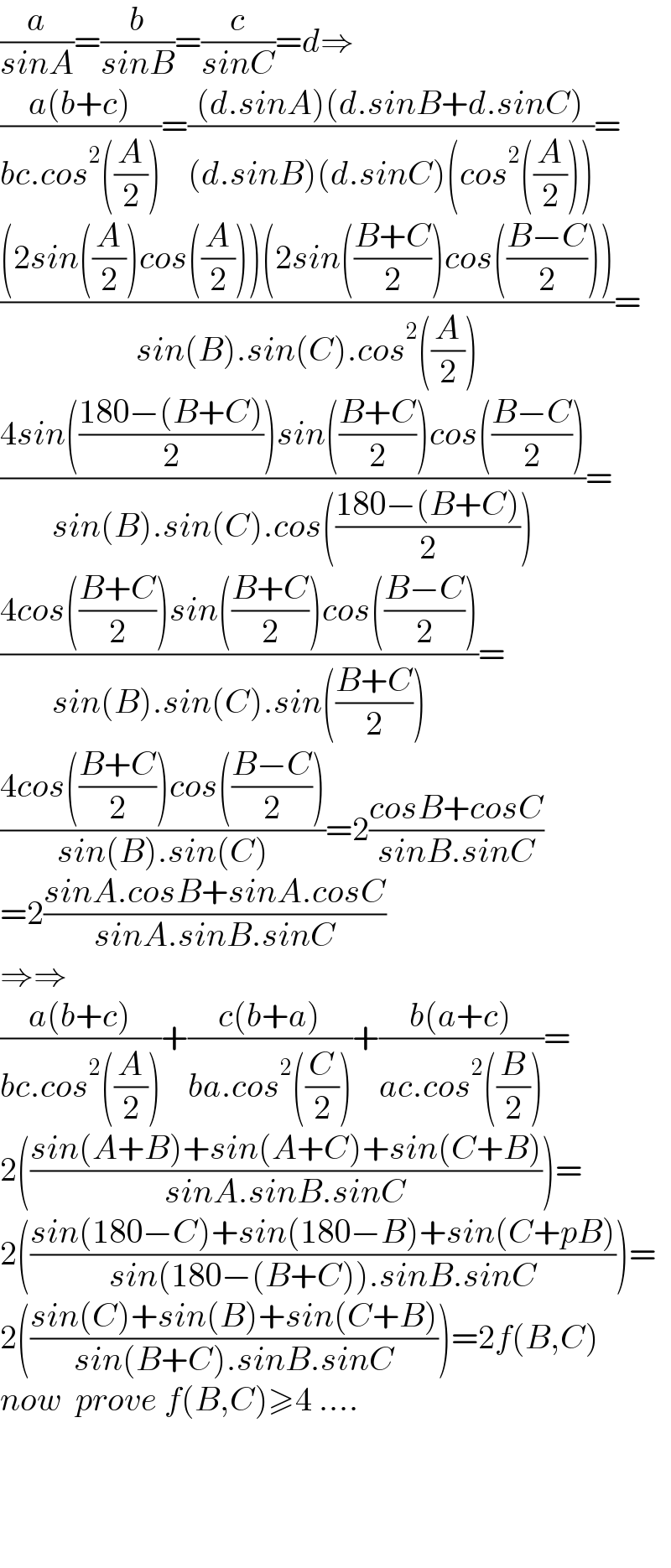
$$\frac{{a}}{{sinA}}=\frac{{b}}{{sinB}}=\frac{{c}}{{sinC}}={d}\Rightarrow \\ $$$$\frac{{a}\left({b}+{c}\right)}{{bc}.{cos}^{\mathrm{2}} \left(\frac{{A}}{\mathrm{2}}\right)}=\frac{\left({d}.{sinA}\right)\left({d}.{sinB}+{d}.{sinC}\right)}{\left({d}.{sinB}\right)\left({d}.{sinC}\right)\left({cos}^{\mathrm{2}} \left(\frac{{A}}{\mathrm{2}}\right)\right)}= \\ $$$$\frac{\left(\mathrm{2}{sin}\left(\frac{{A}}{\mathrm{2}}\right){cos}\left(\frac{{A}}{\mathrm{2}}\right)\right)\left(\mathrm{2}{sin}\left(\frac{{B}+{C}}{\mathrm{2}}\right){cos}\left(\frac{{B}−{C}}{\mathrm{2}}\right)\right)}{{sin}\left({B}\right).{sin}\left({C}\right).{cos}^{\mathrm{2}} \left(\frac{{A}}{\mathrm{2}}\right)}= \\ $$$$\frac{\mathrm{4}{sin}\left(\frac{\mathrm{180}−\left({B}+{C}\right)}{\mathrm{2}}\right){sin}\left(\frac{{B}+{C}}{\mathrm{2}}\right){cos}\left(\frac{{B}−{C}}{\mathrm{2}}\right)}{{sin}\left({B}\right).{sin}\left({C}\right).{cos}\left(\frac{\mathrm{180}−\left({B}+{C}\right)}{\mathrm{2}}\right)}= \\ $$$$\frac{\mathrm{4}{cos}\left(\frac{{B}+{C}}{\mathrm{2}}\right){sin}\left(\frac{{B}+{C}}{\mathrm{2}}\right){cos}\left(\frac{{B}−{C}}{\mathrm{2}}\right)}{{sin}\left({B}\right).{sin}\left({C}\right).{sin}\left(\frac{{B}+{C}}{\mathrm{2}}\right)}= \\ $$$$\frac{\mathrm{4}{cos}\left(\frac{{B}+{C}}{\mathrm{2}}\right){cos}\left(\frac{{B}−{C}}{\mathrm{2}}\right)}{{sin}\left({B}\right).{sin}\left({C}\right)}=\mathrm{2}\frac{{cosB}+{cosC}}{{sinB}.{sinC}} \\ $$$$=\mathrm{2}\frac{{sinA}.{cosB}+{sinA}.{cosC}}{{sinA}.{sinB}.{sinC}} \\ $$$$\Rightarrow\Rightarrow \\ $$$$\frac{{a}\left({b}+{c}\right)}{{bc}.{cos}^{\mathrm{2}} \left(\frac{{A}}{\mathrm{2}}\right)}+\frac{{c}\left({b}+{a}\right)}{{ba}.{cos}^{\mathrm{2}} \left(\frac{{C}}{\mathrm{2}}\right)}+\frac{{b}\left({a}+{c}\right)}{{ac}.{cos}^{\mathrm{2}} \left(\frac{{B}}{\mathrm{2}}\right)}= \\ $$$$\mathrm{2}\left(\frac{{sin}\left({A}+{B}\right)+{sin}\left({A}+{C}\right)+{sin}\left({C}+{B}\right)}{{sinA}.{sinB}.{sinC}}\right)= \\ $$$$\mathrm{2}\left(\frac{{sin}\left(\mathrm{180}−{C}\right)+{sin}\left(\mathrm{180}−{B}\right)+{sin}\left({C}+{pB}\right)}{{sin}\left(\mathrm{180}−\left({B}+{C}\right)\right).{sinB}.{sinC}}\right)= \\ $$$$\mathrm{2}\left(\frac{{sin}\left({C}\right)+{sin}\left({B}\right)+{sin}\left({C}+{B}\right)}{{sin}\left({B}+{C}\right).{sinB}.{sinC}}\right)=\mathrm{2}{f}\left({B},{C}\right) \\ $$$${now}\:\:{prove}\:{f}\left({B},{C}\right)\geqslant\mathrm{4}\:.... \\ $$$$ \\ $$$$ \\ $$$$ \\ $$
Commented by Tawa11 last updated on 15/Sep/22

$$\mathrm{Great}\:\mathrm{sir} \\ $$
