
Question Number 166419 by ajfour last updated on 19/Feb/22

Commented by ajfour last updated on 19/Feb/22

$${Blue}\:{curve}\:\:{y}={x}^{\mathrm{3}} −{x} \\ $$$${Red}\:{curve}\:\:\:\:{y}=\left({x}−{h}\right)−\left({x}−{h}\right)^{\mathrm{3}} +{k} \\ $$$${Find}\:{x}=\:{p}\:\:{when}\:\:{y}={c}. \\ $$
Answered by ajfour last updated on 20/Feb/22
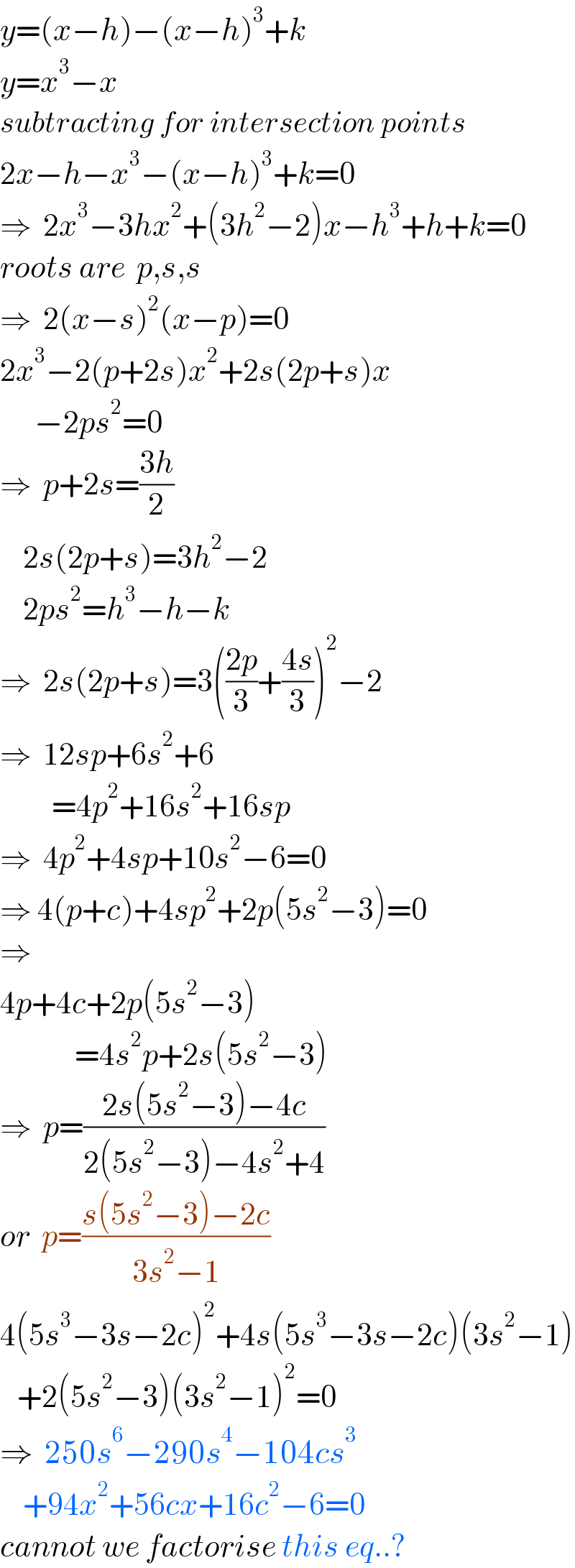
$${y}=\left({x}−{h}\right)−\left({x}−{h}\right)^{\mathrm{3}} +{k} \\ $$$${y}={x}^{\mathrm{3}} −{x} \\ $$$${subtracting}\:{for}\:{intersection}\:{points} \\ $$$$\mathrm{2}{x}−{h}−{x}^{\mathrm{3}} −\left({x}−{h}\right)^{\mathrm{3}} +{k}=\mathrm{0} \\ $$$$\Rightarrow\:\:\mathrm{2}{x}^{\mathrm{3}} −\mathrm{3}{hx}^{\mathrm{2}} +\left(\mathrm{3}{h}^{\mathrm{2}} −\mathrm{2}\right){x}−{h}^{\mathrm{3}} +{h}+{k}=\mathrm{0} \\ $$$${roots}\:{are}\:\:{p},{s},{s} \\ $$$$\Rightarrow\:\:\mathrm{2}\left({x}−{s}\right)^{\mathrm{2}} \left({x}−{p}\right)=\mathrm{0} \\ $$$$\mathrm{2}{x}^{\mathrm{3}} −\mathrm{2}\left({p}+\mathrm{2}{s}\right){x}^{\mathrm{2}} +\mathrm{2}{s}\left(\mathrm{2}{p}+{s}\right){x} \\ $$$$\:\:\:\:\:\:−\mathrm{2}{ps}^{\mathrm{2}} =\mathrm{0} \\ $$$$\Rightarrow\:\:{p}+\mathrm{2}{s}=\frac{\mathrm{3}{h}}{\mathrm{2}} \\ $$$$\:\:\:\:\mathrm{2}{s}\left(\mathrm{2}{p}+{s}\right)=\mathrm{3}{h}^{\mathrm{2}} −\mathrm{2} \\ $$$$\:\:\:\:\mathrm{2}{ps}^{\mathrm{2}} ={h}^{\mathrm{3}} −{h}−{k} \\ $$$$\Rightarrow\:\:\mathrm{2}{s}\left(\mathrm{2}{p}+{s}\right)=\mathrm{3}\left(\frac{\mathrm{2}{p}}{\mathrm{3}}+\frac{\mathrm{4}{s}}{\mathrm{3}}\right)^{\mathrm{2}} −\mathrm{2} \\ $$$$\Rightarrow\:\:\mathrm{12}{sp}+\mathrm{6}{s}^{\mathrm{2}} +\mathrm{6} \\ $$$$\:\:\:\:\:\:\:\:\:=\mathrm{4}{p}^{\mathrm{2}} +\mathrm{16}{s}^{\mathrm{2}} +\mathrm{16}{sp} \\ $$$$\Rightarrow\:\:\mathrm{4}{p}^{\mathrm{2}} +\mathrm{4}{sp}+\mathrm{10}{s}^{\mathrm{2}} −\mathrm{6}=\mathrm{0} \\ $$$$\Rightarrow\:\mathrm{4}\left({p}+{c}\right)+\mathrm{4}{sp}^{\mathrm{2}} +\mathrm{2}{p}\left(\mathrm{5}{s}^{\mathrm{2}} −\mathrm{3}\right)=\mathrm{0} \\ $$$$\Rightarrow \\ $$$$\mathrm{4}{p}+\mathrm{4}{c}+\mathrm{2}{p}\left(\mathrm{5}{s}^{\mathrm{2}} −\mathrm{3}\right) \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:=\mathrm{4}{s}^{\mathrm{2}} {p}+\mathrm{2}{s}\left(\mathrm{5}{s}^{\mathrm{2}} −\mathrm{3}\right) \\ $$$$\Rightarrow\:\:{p}=\frac{\mathrm{2}{s}\left(\mathrm{5}{s}^{\mathrm{2}} −\mathrm{3}\right)−\mathrm{4}{c}}{\mathrm{2}\left(\mathrm{5}{s}^{\mathrm{2}} −\mathrm{3}\right)−\mathrm{4}{s}^{\mathrm{2}} +\mathrm{4}} \\ $$$${or}\:\:{p}=\frac{{s}\left(\mathrm{5}{s}^{\mathrm{2}} −\mathrm{3}\right)−\mathrm{2}{c}}{\mathrm{3}{s}^{\mathrm{2}} −\mathrm{1}} \\ $$$$\mathrm{4}\left(\mathrm{5}{s}^{\mathrm{3}} −\mathrm{3}{s}−\mathrm{2}{c}\right)^{\mathrm{2}} +\mathrm{4}{s}\left(\mathrm{5}{s}^{\mathrm{3}} −\mathrm{3}{s}−\mathrm{2}{c}\right)\left(\mathrm{3}{s}^{\mathrm{2}} −\mathrm{1}\right) \\ $$$$\:\:\:+\mathrm{2}\left(\mathrm{5}{s}^{\mathrm{2}} −\mathrm{3}\right)\left(\mathrm{3}{s}^{\mathrm{2}} −\mathrm{1}\right)^{\mathrm{2}} =\mathrm{0} \\ $$$$\Rightarrow\:\:\mathrm{250}{s}^{\mathrm{6}} −\mathrm{290}{s}^{\mathrm{4}} −\mathrm{104}{cs}^{\mathrm{3}} \\ $$$$\:\:\:\:+\mathrm{94}{x}^{\mathrm{2}} +\mathrm{56}{cx}+\mathrm{16}{c}^{\mathrm{2}} −\mathrm{6}=\mathrm{0} \\ $$$${cannot}\:{we}\:{factorise}\:{this}\:{eq}..? \\ $$
