
Question Number 154465 by Eric002 last updated on 18/Sep/21

Commented by Eric002 last updated on 18/Sep/21

$${a}\:{circle}\:{rolls}\:{inside}\:{a}\:{prabola}.{does}\:{the} \\ $$$${center}\:{of}\:{that}\:{circle}\:{also}\:{trace}\:{a}\:{prabola}? \\ $$$${fond}\:{its}\:{equation} \\ $$$$ \\ $$
Commented by MJS_new last updated on 18/Sep/21
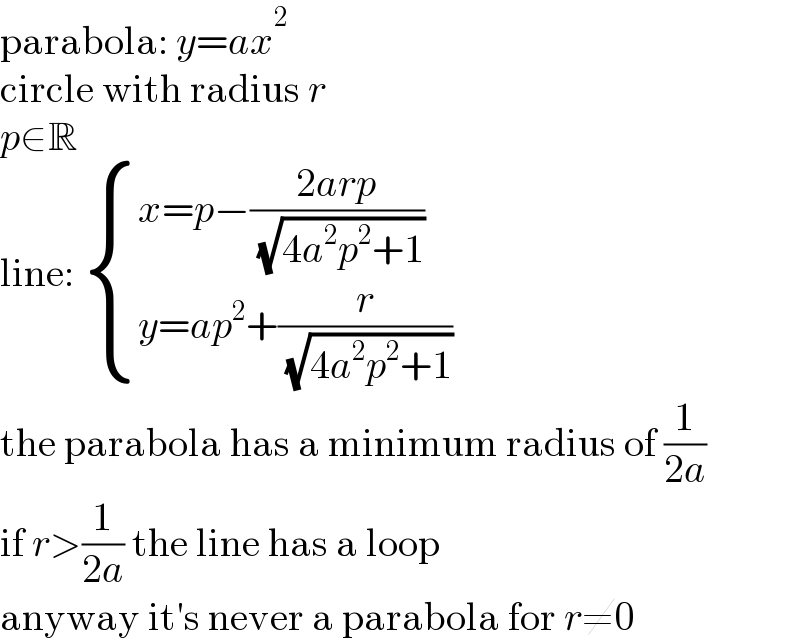
$$\mathrm{parabola}:\:{y}={ax}^{\mathrm{2}} \\ $$$$\mathrm{circle}\:\mathrm{with}\:\mathrm{radius}\:{r} \\ $$$${p}\in\mathbb{R} \\ $$$$\mathrm{line}:\:\begin{cases}{{x}={p}−\frac{\mathrm{2}{arp}}{\:\sqrt{\mathrm{4}{a}^{\mathrm{2}} {p}^{\mathrm{2}} +\mathrm{1}}}}\\{{y}={ap}^{\mathrm{2}} +\frac{{r}}{\:\sqrt{\mathrm{4}{a}^{\mathrm{2}} {p}^{\mathrm{2}} +\mathrm{1}}}}\end{cases} \\ $$$$\mathrm{the}\:\mathrm{parabola}\:\mathrm{has}\:\mathrm{a}\:\mathrm{minimum}\:\mathrm{radius}\:\mathrm{of}\:\frac{\mathrm{1}}{\mathrm{2}{a}} \\ $$$$\mathrm{if}\:{r}>\frac{\mathrm{1}}{\mathrm{2}{a}}\:\mathrm{the}\:\mathrm{line}\:\mathrm{has}\:\mathrm{a}\:\mathrm{loop} \\ $$$$\mathrm{anyway}\:\mathrm{it}'\mathrm{s}\:\mathrm{never}\:\mathrm{a}\:\mathrm{parabola}\:\mathrm{for}\:{r}\neq\mathrm{0} \\ $$
