Question and Answers Forum

All Questions      Topic List

Coordinate Geometry Questions

Previous in All Question      Next in All Question      

Previous in Coordinate Geometry      Next in Coordinate Geometry      

Question Number 153912 by cherokeesay last updated on 12/Sep/21

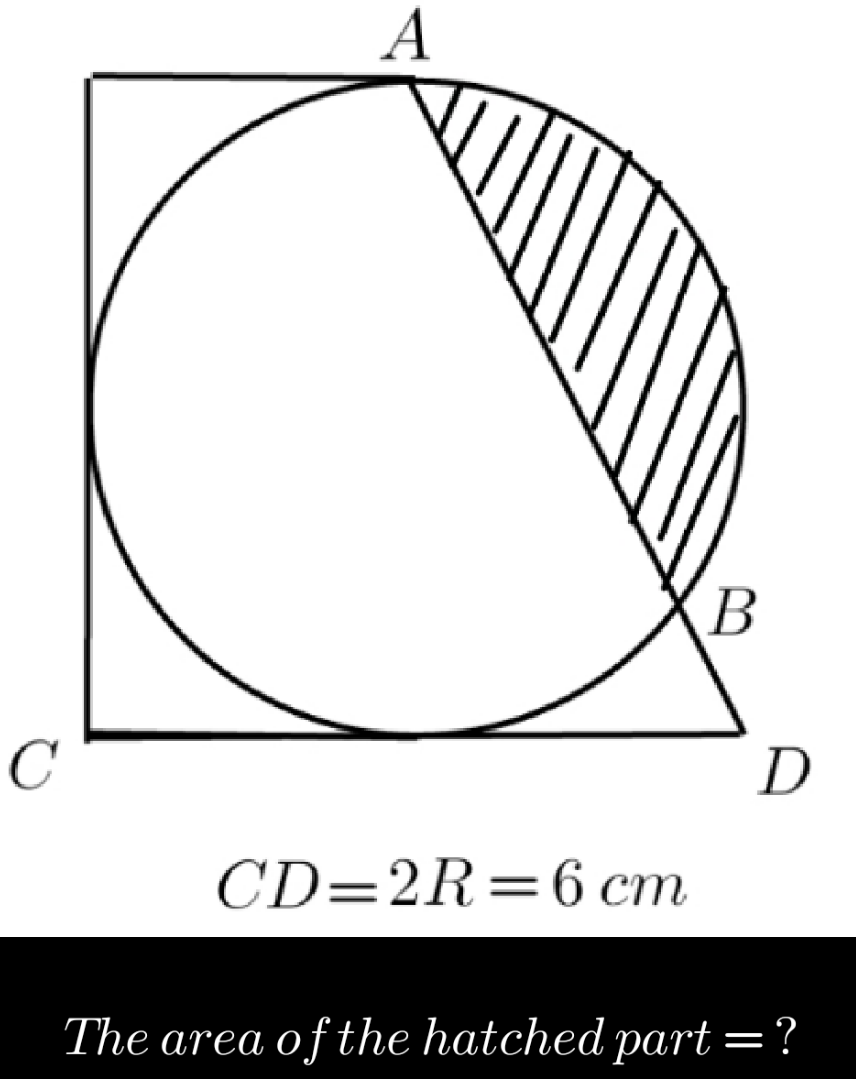
Answered by mr W last updated on 12/Sep/21

Commented by mr W last updated on 12/Sep/21

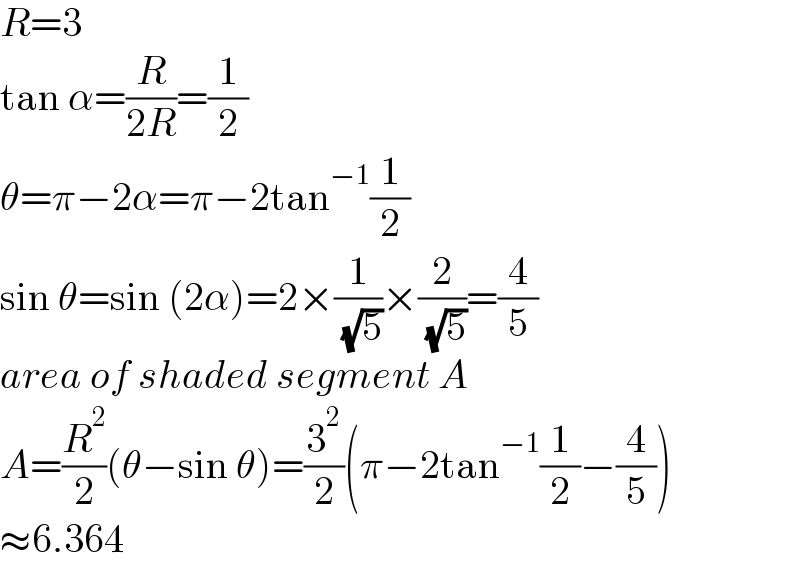
R=3 tan α=(R/(2R))=(1/2) θ=π−2α=π−2tan^(−1) (1/2) sin θ=sin (2α)=2×(1/( (√5)))×(2/( (√5)))=(4/5) area of shaded segment A A=(R^2 /2)(θ−sin θ)=(3^2 /2)(π−2tan^(−1) (1/2)−(4/5)) ≈6.364

$${R}=\mathrm{3} \\ $$$$\mathrm{tan}\:\alpha=\frac{{R}}{\mathrm{2}{R}}=\frac{\mathrm{1}}{\mathrm{2}} \\ $$$$\theta=\pi−\mathrm{2}\alpha=\pi−\mathrm{2tan}^{−\mathrm{1}} \frac{\mathrm{1}}{\mathrm{2}} \\ $$$$\mathrm{sin}\:\theta=\mathrm{sin}\:\left(\mathrm{2}\alpha\right)=\mathrm{2}×\frac{\mathrm{1}}{\:\sqrt{\mathrm{5}}}×\frac{\mathrm{2}}{\:\sqrt{\mathrm{5}}}=\frac{\mathrm{4}}{\mathrm{5}} \\ $$$${area}\:{of}\:{shaded}\:{segment}\:{A} \\ $$$${A}=\frac{{R}^{\mathrm{2}} }{\mathrm{2}}\left(\theta−\mathrm{sin}\:\theta\right)=\frac{\mathrm{3}^{\mathrm{2}} }{\mathrm{2}}\left(\pi−\mathrm{2tan}^{−\mathrm{1}} \frac{\mathrm{1}}{\mathrm{2}}−\frac{\mathrm{4}}{\mathrm{5}}\right) \\ $$$$\approx\mathrm{6}.\mathrm{364} \\ $$

Commented by Tawa11 last updated on 12/Sep/21

Weldone sir

$$\mathrm{Weldone}\:\mathrm{sir} \\ $$

Commented by cherokeesay last updated on 12/Sep/21

it′s very beautiful ! thank you mr W !

$${it}'{s}\:{very}\:{beautiful}\:! \\ $$$${thank}\:{you}\:{mr}\:{W}\:! \\ $$

Commented by talminator2856791 last updated on 12/Sep/21

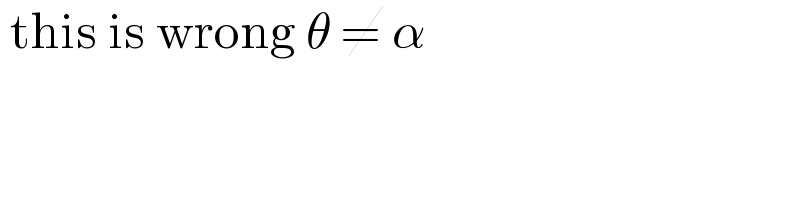
this is wrong θ ≠ α

$$\:\mathrm{this}\:\mathrm{is}\:\mathrm{wrong}\:\theta\:\neq\:\alpha \\ $$

Commented by mr W last updated on 12/Sep/21

who said θ=α? didn′t you see θ=π−2α?

$${who}\:{said}\:\theta=\alpha?\:{didn}'{t}\:{you}\:{see} \\ $$$$\theta=\pi−\mathrm{2}\alpha? \\ $$

Answered by talminator2856791 last updated on 12/Sep/21

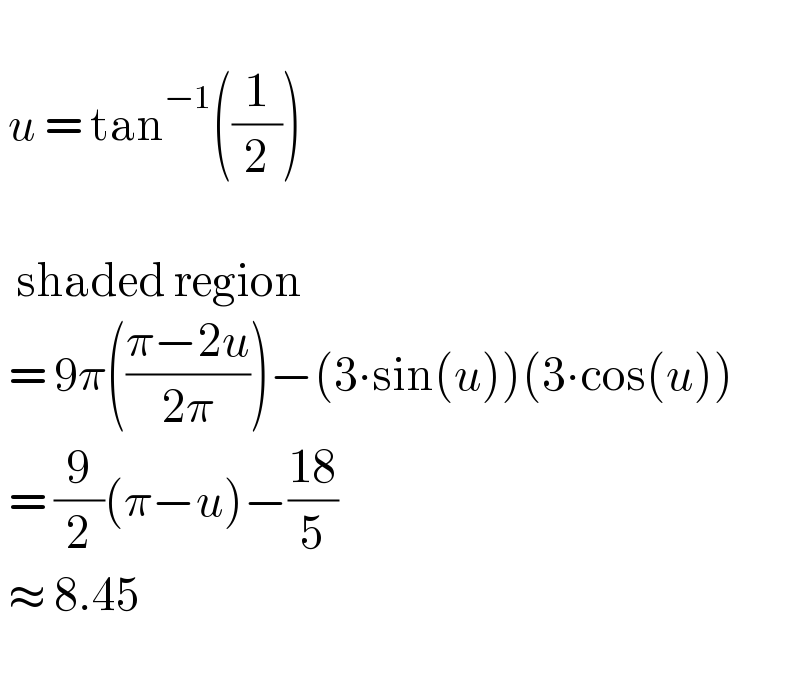
u = tan^(−1) ((1/2)) shaded region = 9π(((π−2u)/(2π)))−(3∙sin(u))(3∙cos(u)) = (9/2)(π−u)−((18)/5) ≈ 8.45

$$\: \\ $$$$\:{u}\:=\:\mathrm{tan}^{−\mathrm{1}} \left(\frac{\mathrm{1}}{\mathrm{2}}\right) \\ $$$$\: \\ $$$$\:\:\mathrm{shaded}\:\mathrm{region} \\ $$$$\:=\:\mathrm{9}\pi\left(\frac{\pi−\mathrm{2}{u}}{\mathrm{2}\pi}\right)−\left(\mathrm{3}\centerdot\mathrm{sin}\left({u}\right)\right)\left(\mathrm{3}\centerdot\mathrm{cos}\left({u}\right)\right)\: \\ $$$$\:=\:\frac{\mathrm{9}}{\mathrm{2}}\left(\pi−{u}\right)−\frac{\mathrm{18}}{\mathrm{5}} \\ $$$$\:\approx\:\mathrm{8}.\mathrm{45} \\ $$$$\: \\ $$

Commented by cherokeesay last updated on 12/Sep/21

numerically : A_(A.S.P) = ((π.9[180°−(2×26,565°)])/(360°)) −3,6= = 9,96 − 3,6 = 6,36 cm^2

$${numerically}\:: \\ $$$$\mathscr{A}_{{A}.{S}.{P}} =\:\frac{\pi.\mathrm{9}\left[\mathrm{180}°−\left(\mathrm{2}×\mathrm{26},\mathrm{565}°\right)\right]}{\mathrm{360}°}\:−\mathrm{3},\mathrm{6}= \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:=\:\mathrm{9},\mathrm{96}\:−\:\mathrm{3},\mathrm{6}\:=\:\mathrm{6},\mathrm{36}\:{cm}^{\mathrm{2}} \\ $$

Commented by cherokeesay last updated on 12/Sep/21

thank you sir !

$${thank}\:{you}\:{sir}\:! \\ $$

Commented by mr W last updated on 12/Sep/21

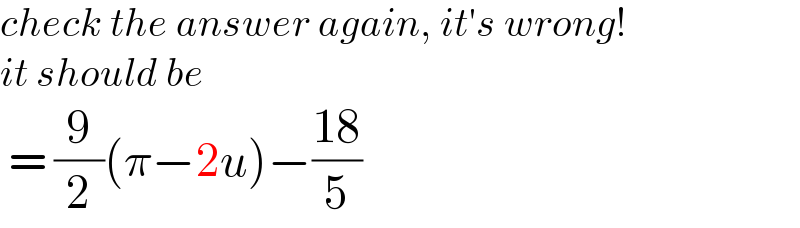
check the answer again, it′s wrong! it should be = (9/2)(π−2u)−((18)/5)

$${check}\:{the}\:{answer}\:{again},\:{it}'{s}\:{wrong}!\: \\ $$$${it}\:{should}\:{be} \\ $$$$\:=\:\frac{\mathrm{9}}{\mathrm{2}}\left(\pi−\mathrm{2}{u}\right)−\frac{\mathrm{18}}{\mathrm{5}} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com