Question and Answers Forum

All Questions      Topic List

Differentiation Questions

Previous in All Question      Next in All Question      

Previous in Differentiation      Next in Differentiation      

Question Number 152904 by DELETED last updated on 03/Sep/21

Answered by DELETED last updated on 03/Sep/21

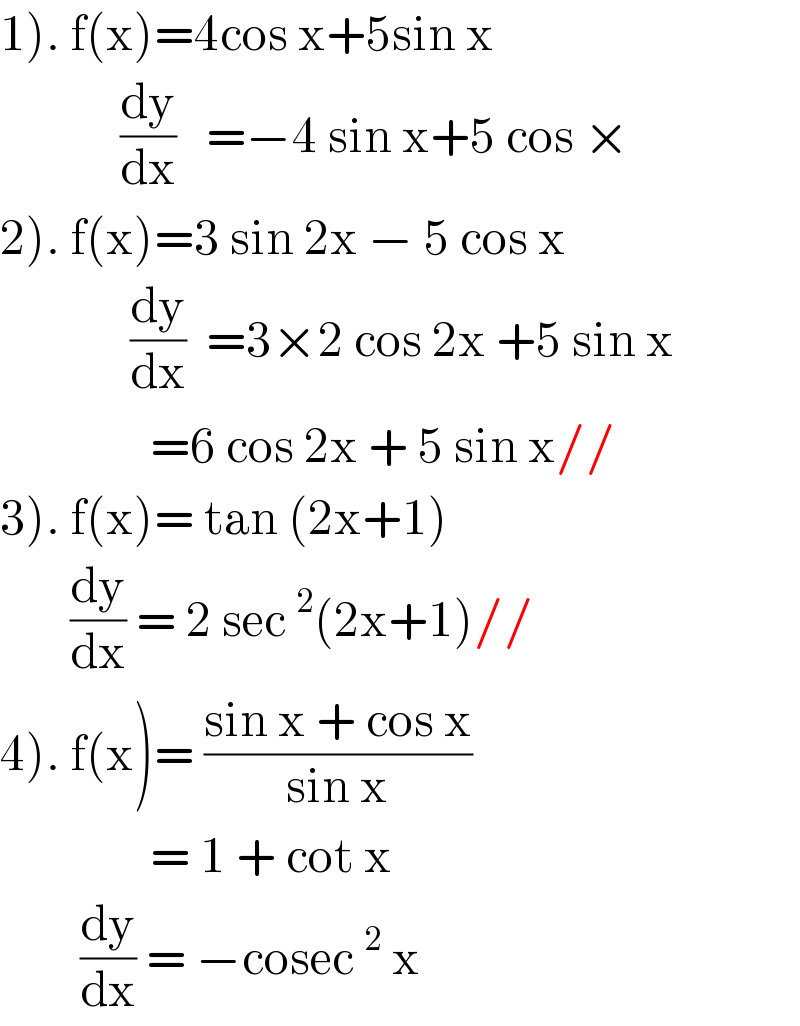
1). f(x)=4cos x+5sin x (dy/dx) =−4 sin x+5 cos × 2). f(x)=3 sin 2x − 5 cos x (dy/dx) =3×2 cos 2x +5 sin x =6 cos 2x + 5 sin x// 3). f(x)= tan (2x+1) (dy/dx) = 2 sec^2 (2x+1)// 4). f(x)= ((sin x + cos x)/(sin x)) = 1 + cot x (dy/dx) = −cosec^2 x

$$\left.\mathrm{1}\right).\:\mathrm{f}\left(\mathrm{x}\right)=\mathrm{4cos}\:\mathrm{x}+\mathrm{5sin}\:\mathrm{x} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\frac{\mathrm{dy}}{\mathrm{dx}}\:\:\:=−\mathrm{4}\:\mathrm{sin}\:\mathrm{x}+\mathrm{5}\:\mathrm{cos}\:× \\ $$$$\left.\mathrm{2}\right).\:\mathrm{f}\left(\mathrm{x}\right)=\mathrm{3}\:\mathrm{sin}\:\mathrm{2x}\:−\:\mathrm{5}\:\mathrm{cos}\:\mathrm{x} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\frac{\mathrm{dy}}{\mathrm{dx}}\:\:=\mathrm{3}×\mathrm{2}\:\mathrm{cos}\:\mathrm{2x}\:+\mathrm{5}\:\mathrm{sin}\:\mathrm{x} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\mathrm{6}\:\mathrm{cos}\:\mathrm{2x}\:+\:\mathrm{5}\:\mathrm{sin}\:\mathrm{x}// \\ $$$$\left.\mathrm{3}\right).\:\mathrm{f}\left(\mathrm{x}\right)=\:\mathrm{tan}\:\left(\mathrm{2x}+\mathrm{1}\right) \\ $$$$\:\:\:\:\:\:\:\frac{\mathrm{dy}}{\mathrm{dx}}\:=\:\mathrm{2}\:\mathrm{sec}\:^{\mathrm{2}} \left(\mathrm{2x}+\mathrm{1}\right)// \\ $$$$\left.\mathrm{4}\right).\:\mathrm{f}\left(\mathrm{x}\right)=\:\frac{\mathrm{sin}\:\mathrm{x}\:+\:\mathrm{cos}\:\mathrm{x}}{\mathrm{sin}\:\mathrm{x}} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\:\mathrm{1}\:+\:\mathrm{cot}\:\mathrm{x} \\ $$$$\:\:\:\:\:\:\:\:\frac{\mathrm{dy}}{\mathrm{dx}}\:=\:−\mathrm{cosec}\:^{\mathrm{2}} \:\mathrm{x} \\ $$

Answered by DELETED last updated on 03/Sep/21

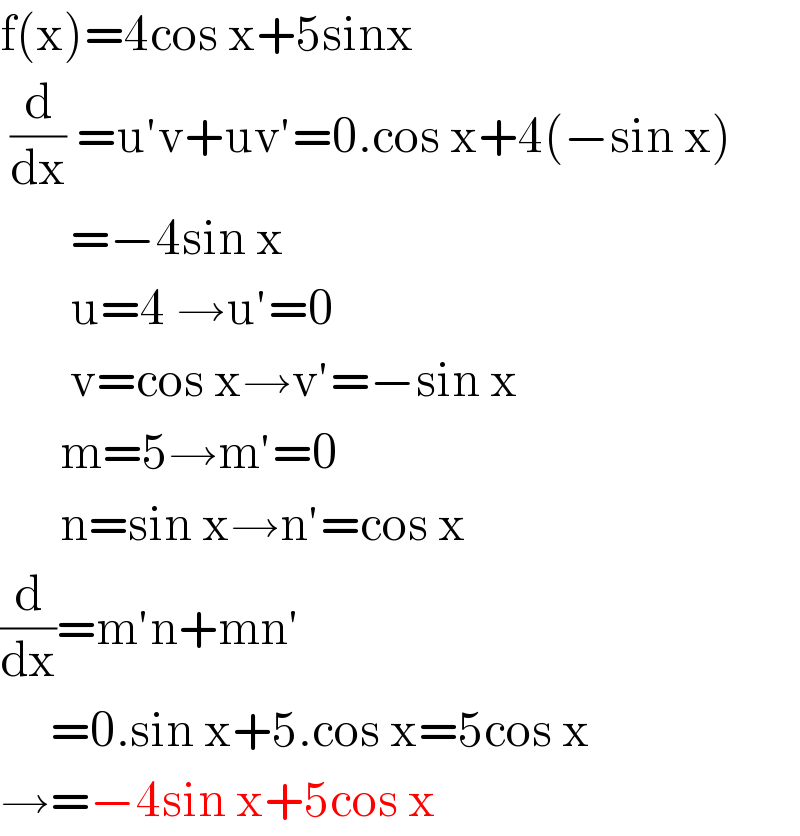
f(x)=4cos x+5sinx (d/dx) =u′v+uv′=0.cos x+4(−sin x) =−4sin x u=4 →u′=0 v=cos x→v′=−sin x m=5→m′=0 n=sin x→n′=cos x (d/dx)=m′n+mn′ =0.sin x+5.cos x=5cos x →=−4sin x+5cos x

$$\mathrm{f}\left(\mathrm{x}\right)=\mathrm{4cos}\:\mathrm{x}+\mathrm{5sinx} \\ $$$$\:\frac{\mathrm{d}}{\mathrm{dx}}\:=\mathrm{u}'\mathrm{v}+\mathrm{uv}'=\mathrm{0}.\mathrm{cos}\:\mathrm{x}+\mathrm{4}\left(−\mathrm{sin}\:\mathrm{x}\right) \\ $$$$\:\:\:\:\:\:\:=−\mathrm{4sin}\:\mathrm{x} \\ $$$$\:\:\:\:\:\:\:\mathrm{u}=\mathrm{4}\:\rightarrow\mathrm{u}'=\mathrm{0} \\ $$$$\:\:\:\:\:\:\:\mathrm{v}=\mathrm{cos}\:\mathrm{x}\rightarrow\mathrm{v}'=−\mathrm{sin}\:\mathrm{x} \\ $$$$\:\:\:\:\:\:\mathrm{m}=\mathrm{5}\rightarrow\mathrm{m}'=\mathrm{0} \\ $$$$\:\:\:\:\:\:\mathrm{n}=\mathrm{sin}\:\mathrm{x}\rightarrow\mathrm{n}'=\mathrm{cos}\:\mathrm{x} \\ $$$$\frac{\mathrm{d}}{\mathrm{dx}}=\mathrm{m}'\mathrm{n}+\mathrm{mn}' \\ $$$$\:\:\:\:\:=\mathrm{0}.\mathrm{sin}\:\mathrm{x}+\mathrm{5}.\mathrm{cos}\:\mathrm{x}=\mathrm{5cos}\:\mathrm{x} \\ $$$$\rightarrow=−\mathrm{4sin}\:\mathrm{x}+\mathrm{5cos}\:\mathrm{x} \\ $$

Answered by DELETED last updated on 03/Sep/21

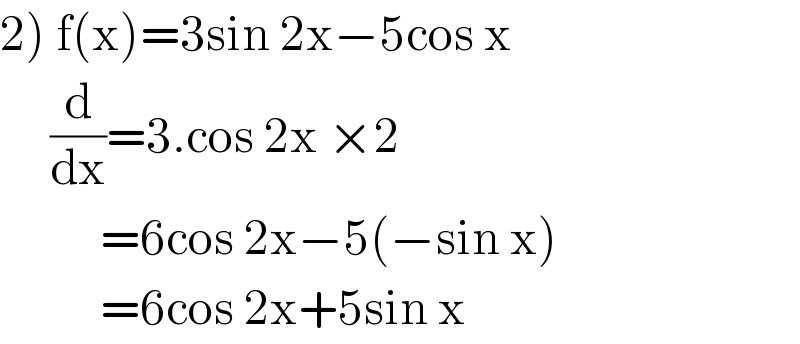
2) f(x)=3sin 2x−5cos x (d/dx)=3.cos 2x ×2 =6cos 2x−5(−sin x) =6cos 2x+5sin x

$$\left.\mathrm{2}\right)\:\mathrm{f}\left(\mathrm{x}\right)=\mathrm{3sin}\:\mathrm{2x}−\mathrm{5cos}\:\mathrm{x} \\ $$$$\:\:\:\:\:\frac{\mathrm{d}}{\mathrm{dx}}=\mathrm{3}.\mathrm{cos}\:\mathrm{2x}\:×\mathrm{2}\: \\ $$$$\:\:\:\:\:\:\:\:\:\:=\mathrm{6cos}\:\mathrm{2x}−\mathrm{5}\left(−\mathrm{sin}\:\mathrm{x}\right) \\ $$$$\:\:\:\:\:\:\:\:\:\:=\mathrm{6cos}\:\mathrm{2x}+\mathrm{5sin}\:\mathrm{x} \\ $$

Answered by DELETED last updated on 03/Sep/21

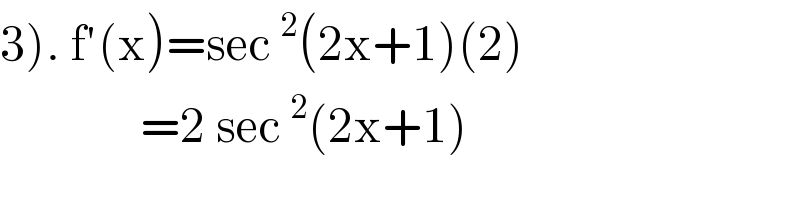
3). f′(x)=sec^2 (2x+1)(2) =2 sec^2 (2x+1)

$$\left.\mathrm{3}\right).\:\mathrm{f}'\left(\mathrm{x}\right)=\mathrm{sec}\:^{\mathrm{2}} \left(\mathrm{2x}+\mathrm{1}\right)\left(\mathrm{2}\right) \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\mathrm{2}\:\mathrm{sec}\:^{\mathrm{2}} \left(\mathrm{2x}+\mathrm{1}\right) \\ $$

Answered by DELETED last updated on 03/Sep/21

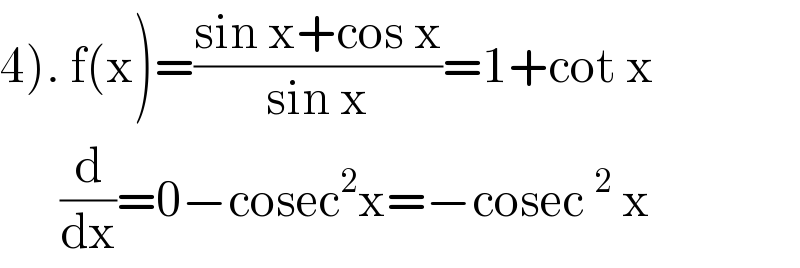
4). f(x)=((sin x+cos x)/(sin x))=1+cot x (d/dx)=0−cosec^2 x=−cosec^2 x

$$\left.\mathrm{4}\right).\:\mathrm{f}\left(\mathrm{x}\right)=\frac{\mathrm{sin}\:\mathrm{x}+\mathrm{cos}\:\mathrm{x}}{\mathrm{sin}\:\mathrm{x}}=\mathrm{1}+\mathrm{cot}\:\mathrm{x} \\ $$$$\:\:\:\:\:\:\frac{\mathrm{d}}{\mathrm{dx}}=\mathrm{0}−\mathrm{cosec}^{\mathrm{2}} \mathrm{x}=−\mathrm{cosec}\:^{\mathrm{2}} \:\mathrm{x} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com